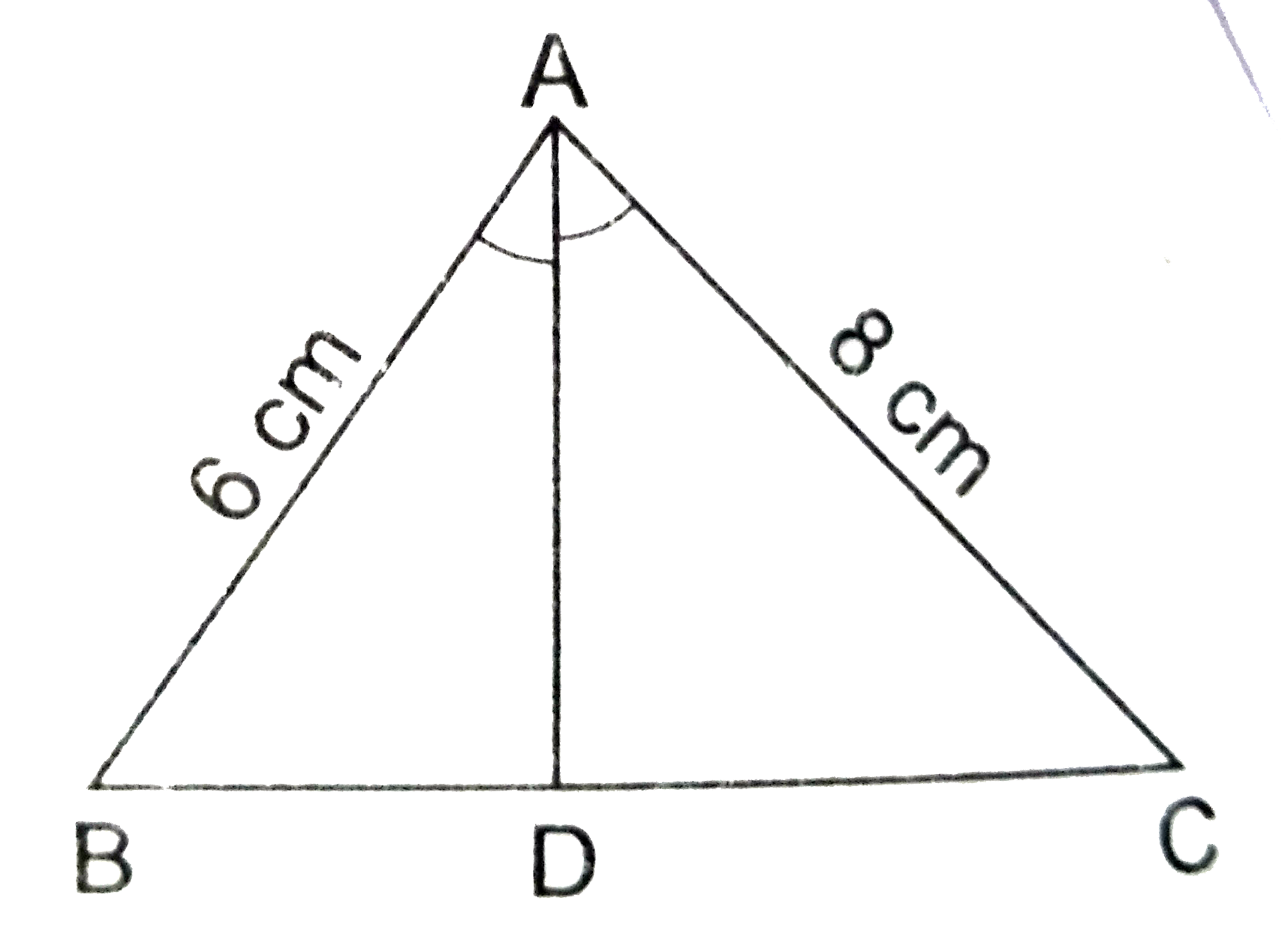
An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides. In Fig. 3, $△ A B C$ is given in which AB =AC and AD is the bisector of $∠ B A C$ Then, AD is the line of symmetry of $△ A B C$ .true or false?

Video Solution

Answer

Step by step video & image solution for An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides. In Fig. 3, triangle ABC is given in which AB =AC and AD is the bisector of angle BACThen, AD is the line of symmetry of triangle ABC.true or false? by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.

Updated on:21/07/2023

Class 7 MATHS SYMMETRY

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One or More
In a triangle, with usual notations, the length of the bisector of angle A is
A $2 \frac{b c}{b + c} sin \frac{A}{2}$
B $2 \frac{b c}{b + c} cos \frac{A}{2}$
C $\frac{a b c}{2 R (b + c)} cos e c \frac{A}{2}$
D $\frac{4 A}{b + c} cos e c \frac{A}{2}$
Question 2 - Select One
In a $△ A B C$ . AB = 5 cm, AC = 7 cm. If AD is the angle bisector of angle A, then BD :CD is :
A25:49
B49:25
C6:1
D5:7
Question 3 - Select One
In the adjoining figure, if ABC is a triangle in which AD is the bisector of $∠ A$ . If $A D ⊥ B C$ , then

A $Δ A B C$ is an isosceles triangle
B $Δ A B C$ is an equilateral triangle
C $Δ A B C$ is an scalene triangle
DBD = AC

RS AGGARWAL-REFLECTION AND ROTATIONAL SYMMETRY-Exercise B

Home
Ask Doubt
Profile

An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides. In Fig. 3, △ABC is given in which AB =AC and AD is the bisector of ∠BACThen, AD is the line of symmetry of △ABC.true or false?

Topper's Solved these Questions

Similar Questions

Knowledge Check

In a triangle, with usual notations, the length of the bisector of angle A is

In a △ABC. AB = 5 cm, AC = 7 cm. If AD is the angle bisector of angle A, then BD :CD is :

In the adjoining figure, if ABC is a triangle in which AD is the bisector of ∠A. If AD⊥BC, then

RS AGGARWAL-REFLECTION AND ROTATIONAL SYMMETRY-Exercise B

An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides. In Fig. 3, $△ A B C$ is given in which AB =AC and AD is the bisector of $∠ B A C$ Then, AD is the line of symmetry of $△ A B C$ .true or false?

In a $△ A B C$ . AB = 5 cm, AC = 7 cm. If AD is the angle bisector of angle A, then BD :CD is :

In the adjoining figure, if ABC is a triangle in which AD is the bisector of $∠ A$ . If $A D ⊥ B C$ , then