An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides. true or false?
To determine whether the statement "An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides" is true or false, we can follow these steps:
1. Understand the Properties of an Isosceles Triangle:
- An isosceles triangle has two sides that are equal in length. These sides are often referred to as the "equal sides," and the third side is called the "base."
2. Identify the Angle Bisector:
- The angle included between the equal sides is the angle formed at the vertex where the two equal sides meet. The bisector of this angle divides it into two equal angles.
3. Draw the Isosceles Triangle:
- Sketch an isosceles triangle with vertices labeled A (the vertex where the equal sides meet), B, and C (the endpoints of the equal sides). Draw the angle bisector from point A to the base BC.
4. Analyze the Symmetry:
- When you draw the angle bisector, it will divide the triangle into two smaller triangles: triangle ABD and triangle ACD. Since AB = AC (the equal sides), and the angle bisector divides the angle at A into two equal parts, triangles ABD and ACD are congruent.
5. Conclusion on Symmetry:
- Because the angle bisector divides the triangle into two congruent halves, it acts as a line of symmetry. This means that if you were to fold the triangle along this bisector, the two halves would match perfectly.
6. Final Answer:
- Therefore, the statement is true: An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides.
- An isosceles triangle has two sides that are equal in length. These sides are often referred to as the "equal sides," and the third side is called the "base."
- The angle included between the equal sides is the angle formed at the vertex where the two equal sides meet. The bisector of this angle divides it into two equal angles.
- Sketch an isosceles triangle with vertices labeled A (the vertex where the equal sides meet), B, and C (the endpoints of the equal sides). Draw the angle bisector from point A to the base BC.
- When you draw the angle bisector, it will divide the triangle into two smaller triangles: triangle ABD and triangle ACD. Since AB = AC (the equal sides), and the angle bisector divides the angle at A into two equal parts, triangles ABD and ACD are congruent.
- Because the angle bisector divides the triangle into two congruent halves, it acts as a line of symmetry. This means that if you were to fold the triangle along this bisector, the two halves would match perfectly.
- Therefore, the statement is true: An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides.
Topper's Solved these Questions
- TWO-DIMENSIONAL REFLECTION SYMMETRY (LINEAR SYMMETRY)BOOK - RS AGGARWALCHAPTER - TWO-DIMENSIONAL REFLECTION SYMMETRY (LINEAR SYMMETRY)EXERCISE - EXERCISE
10 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 16B (objective questions)
11 Videos
- WHOLE NUMBERSBOOK - RS AGGARWALCHAPTER - WHOLE NUMBERSEXERCISE - TEST PAPER ( true and false )
4 Videos
Similar Questions
An isosceles triangle is symmetrical about the bisector of the angle included between the equal sides. In Fig. 3, △ABC is given in which AB =AC and AD is the bisector of ∠BACThen, AD is the line of symmetry of △ABC.true or false?
View SolutionAn equilateral triangle is symmetrical about each one of the bisectors of its interior angles. true or false ?
View Solution
Knowledge Check
Assertion: In the given figure, LM = ON and NL = MO, then △NOM≅△MLN
Reason: Two triangles are congruent if two sides and the angle included between them in one of the triangles are equal to the corresponding sides and the angle included between them of the other triangle
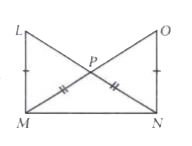 AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is falseDIf assertion is false but reason is true.
AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is falseDIf assertion is false but reason is true.The length of each equal side of an isosceles triangle is 25 cm and the included angle between those two sides is 90°. Find the area of triangle.
Aa. 625/2 cm2Bb. 225/2 cm2Cc. 125/2 cm2Dd. 225 cm2
An equilateral triangle is symmetrical about each one of the bisectors of its interior angles. true or false?
View SolutionA given angle having equal arms is symmetrical about the bisector of the angle. true or false ?
View SolutionLength of each equal side of an isosceles triangle is 10 cm and the included angle between those two sides is 45∘. Find the area of the triangle.
View SolutionA line segement is symmetrical about its perpendicular bisector. true or false ?
View SolutionA line segment is symmetrical about its perpendicular bisector.True or false?
View SolutionLet triangle ABC be an isosceles triangle with AB=AC. Suppose that the angle bisector of its angle B meets the side AC at a point D and that BC=BD+AD. Find angle A (in degrees).
View Solution
