How long will it take a force of 10 N to stop a mass of 2.5 kg which is moving at 20 m/s?
The correct Answer is:5 s
To solve the problem of how long it will take a force of 10 N to stop a mass of 2.5 kg moving at 20 m/s, we can follow these steps:
Step 1: Identify the given values
- Mass (m) = 2.5 kg
- Initial velocity (u) = 20 m/s
- Force (F) = 10 N
Step 2: Calculate the acceleration (or retardation)
Using Newton's second law of motion, we can find the acceleration produced by the force:
F=m⋅a
Rearranging this gives us:
a=Fm
Substituting the values:
a=10N2.5kg=4m/s2
Since the force is acting in the opposite direction to the motion (to stop the object), the acceleration will be negative:
a=−4m/s2
Step 3: Use the first equation of motion
We will use the first equation of motion to find the time taken to stop:
v=u+at
Where:
- v = final velocity = 0 m/s (since the object stops)
- u = initial velocity = 20 m/s
- a = acceleration = -4 m/s²
Substituting the known values:
0=20+(−4)t
This simplifies to:
0=20−4t
Rearranging gives:
4t=20
Now, solving for t:
t=204=5seconds
Conclusion
The time taken to stop the mass of 2.5 kg moving at 20 m/s with a force of 10 N is 5 seconds.
---
- Mass (m) = 2.5 kg
- Initial velocity (u) = 20 m/s
- Force (F) = 10 N
Using Newton's second law of motion, we can find the acceleration produced by the force:
F=m⋅a
Rearranging this gives us:
a=Fm
Substituting the values:
a=10N2.5kg=4m/s2
Since the force is acting in the opposite direction to the motion (to stop the object), the acceleration will be negative:
a=−4m/s2
We will use the first equation of motion to find the time taken to stop:
v=u+at
Where:
- v = final velocity = 0 m/s (since the object stops)
- u = initial velocity = 20 m/s
- a = acceleration = -4 m/s²
0=20+(−4)t
This simplifies to:
0=20−4t
Rearranging gives:
4t=20
Now, solving for t:
t=204=5seconds
The time taken to stop the mass of 2.5 kg moving at 20 m/s with a force of 10 N is 5 seconds.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
How long will it take to stop a car travelling at a speed of 20m/sec, if the uniform acc. during braking is −5m/s2?
A100sB4sC(1/4)sD(1/100)s - Question 2 - Select One
The masses of 10kig and 20kg respectively are connected by a massless spring as shown in figure. A force of 200N acts on the 20kg mass. At the instant shown, the 10kg mass has acceleration 12m/sec2 . What is the acceleration of 20kg mass?
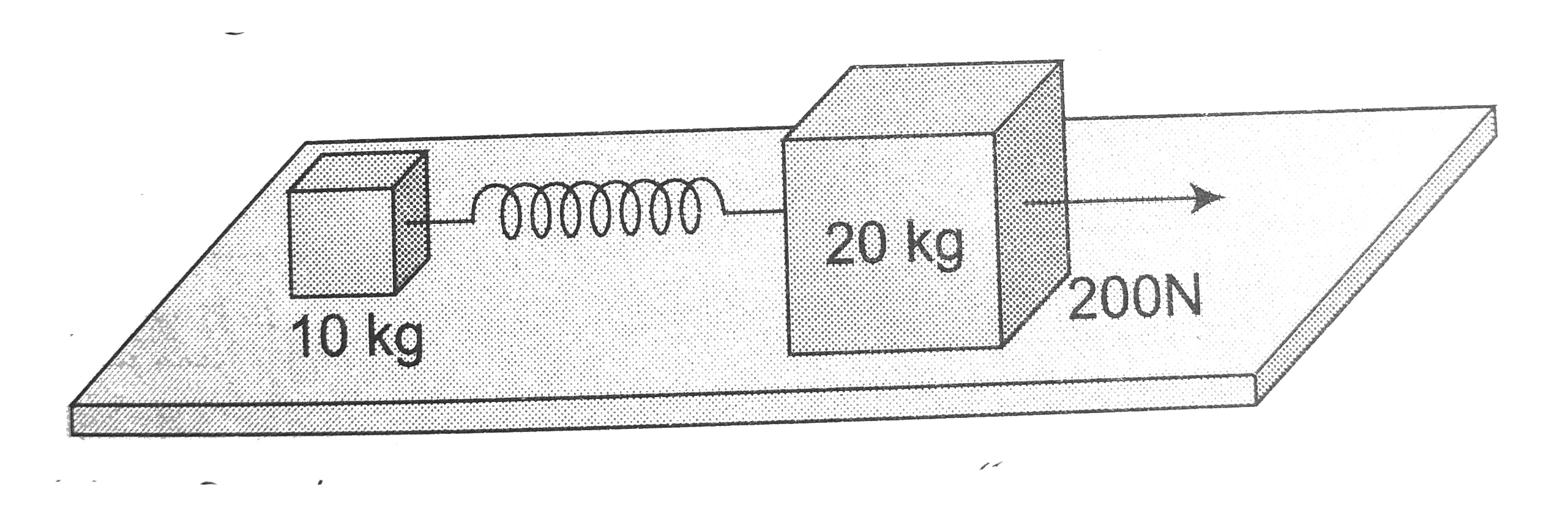 A12m/sec2B4m/sec2C10m/sec2DZero
A12m/sec2B4m/sec2C10m/sec2DZero - Question 3 - Select One
The acceleration produced by a force of 5N acting on a mass of 20 Kg in m/s2 is
A4,B100,mC0.25,D2.5
A constant retarding force of 50 N is applied to a body of mass 20 kg moving intially with a speed of 15ms−1 How long does the body take to stop ?
View SolutionTwo masses 10 kg and 20 kg respectively are connected by a massless spring as shown in figure force of 200 N acts on the 20 kg mass. At the instant shown is figure the 10 kg mass has acceleration of 12m/s2 what is the acceleration of 20 kg mass?
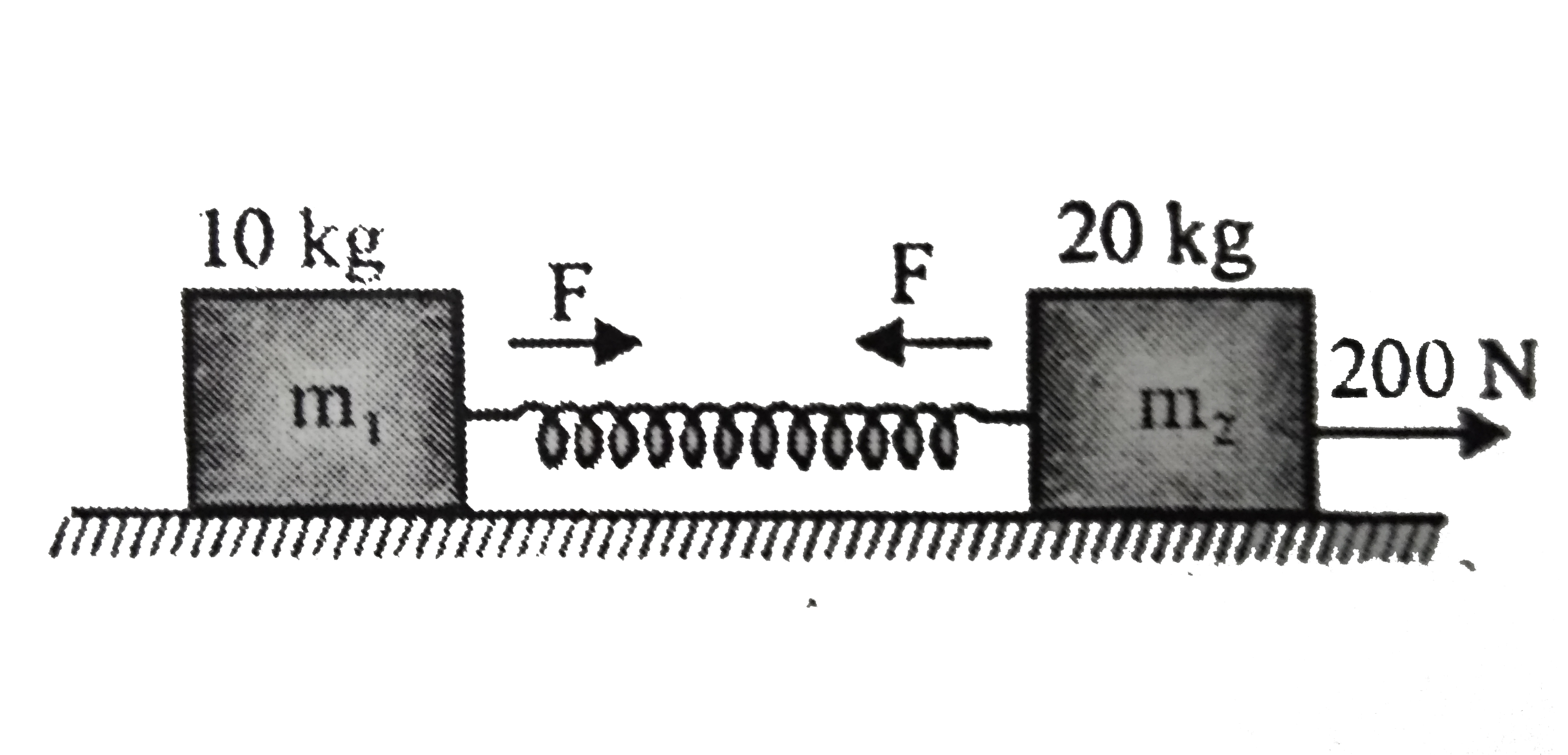 View Solution
View Solution
Two blocks of masses 5 kg and 10 kg are connected by a massless spring as shown in the diagram. A force of 100 N acts on 10kg mass as shown. At a certain instant the acceleration of 5 kg mass is 12 m/s2 find the force in the spring and acceleration of 10 kg mass.View SolutionThe masses of 10 kg and 20 kg respectively are connected by a massless spring as shown in figure. A force of 200 N acts on the 20 kg mass. At the instant shown, the 10 kg mass has acceleration 12m/sec2 . What is the acceleration of 20 kg mass
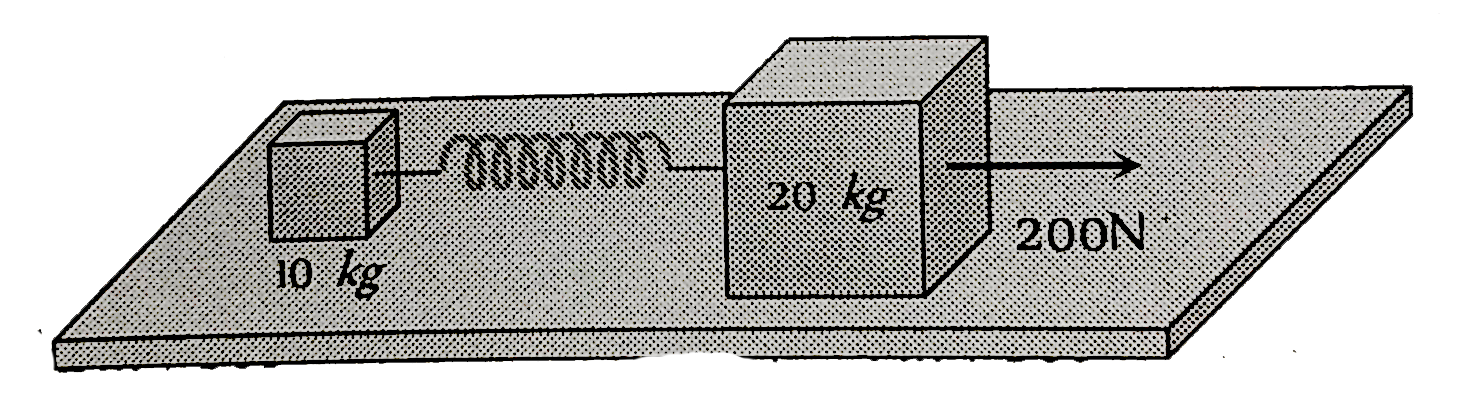 View Solution
View SolutionThe masses of 10 kg and 20 kg, respectively, are connected by a light spring as shown in the figure. A force of 200 N acts upon the 20 kg mass. At the instant shown, the 10 kg mass the acceleration of 12m/s2. The acceleration of 20 kg mass is
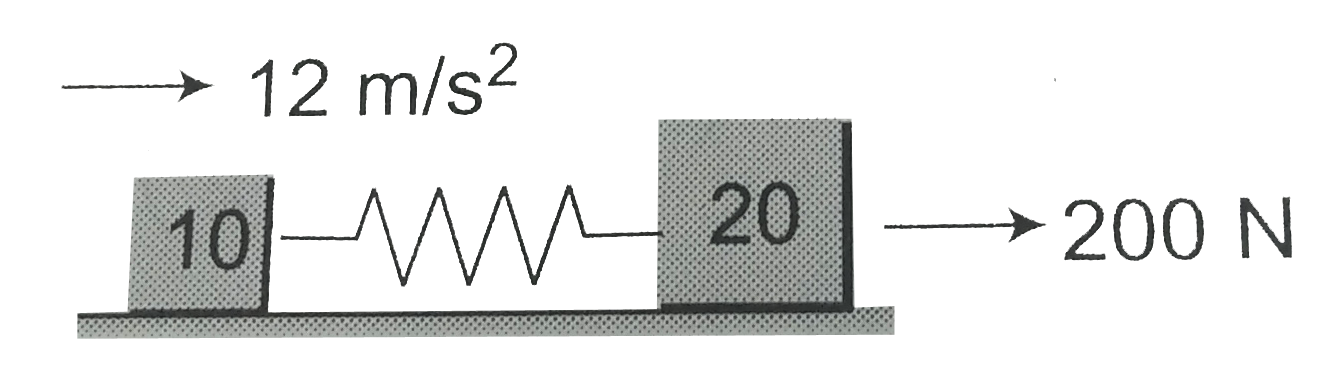 View Solution
View Solution

