The following shows the graph of a continuous function y=f(x)on the interval [1,3]. The points A, B, C have coordinates (1,1),(3,2),(2,3) respectively, and the lines L1 and L2 are parallel, with L1 being tangent to the curve at C. If the area under the graph of y=f(x) from x=1 to x=3 is 4 square units, then the area of the shaded region is -
![The following shows the graph of a continuous function y = f(x)on the interval [1,3]. The points A, B, C have coordinates (1,1), (3,2), (2,3) respectively, and the lines L(1) and L(2) are parallel, with L(1) being tangent to the curve at C. If the area under the graph of y = f(x) from x = 1 to x = 3 is 4 square units, then the area of the shaded region is -](https://d10lpgp6xz60nq.cloudfront.net/physics_images/KVPY_CP_2010_XII_P1_E01_013_Q01.png)
2
3
4
5
The correct Answer is:A
Equation of l2→y−1=2−13−1(x−1)
2y−2=x−1
2y−x=1
⇒ slope of l1=1/2
Equation of l1=y−3=12(x−2)
2y−6=x−2
2y−x=4
D→(1,52),E→(3,72)
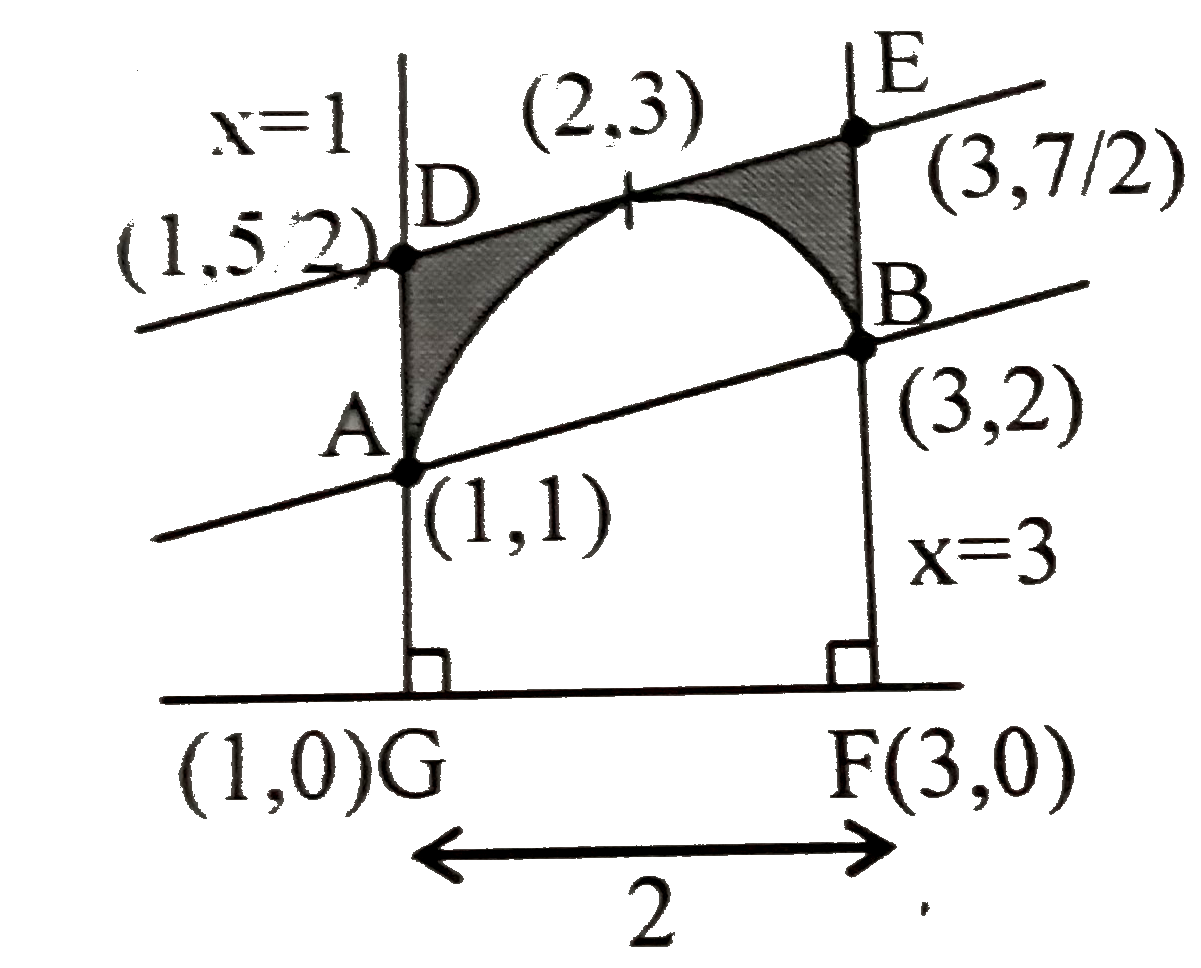
area under f(x)=4
shaded area = area of trapezium DEFG - area under f(x)
=12(52+72)×2−4
=6−4=2
Topper's Solved these Questions
Similar Questions
The folloiwng figure shows the graph of a continuous function y = f(x) on the interval [1, 3]. The points A, B, C have coordinates (1,1), (3,2),(2,3), respectively , and the lines L1andL2 are parrallel, with L1 being tangent to the curve at C. If the area under the graph of y = f(x) from x = 1 to x = 3 is 4 square units, then the area of the shaded region is

View SolutionGraph of a function y=f(x) is shown in the adjacent figure.Four limits l1,l2,l3 and I4, are given as:
View Solution
Knowledge Check
A function y = f ( x) has a second order derivative f''(x) = 6 (x-1). If the graph passes through the point (2, 1) and at this point tangent to the graph is y = 3x - 1, then function is :
A(x−1)3B(x−1)2C(x+1)3D(x+1)2A function y = f ( x) has a second order derivative f''(x) = 6 (x-1). If the graph passes through the point (2, 1) and at this point tangent to the graph is y = 3x - 1, then function is :
A(x+1)3B(x−1)3C(x+1)2D(x−1)2
Coordinates of the point on the graph of the function f(x) = x−12−x where the tangent lines are perpendicular to the lines 8x + 2y = 1, are
View SolutionIf graph of the function y=f(x) is continuous and passes through point (3, 1) then limx→3loge(3f(x)−2)2(1−f(x)) is equal
View SolutionA function y=f(x) has a second order derivative f(x)=6(x−1). If its graph passes through the point (2,1) and at that point the tangent to the graph is y=3x−5 then the function is
View SolutionThe equation of line l1 is y=2x+3, and the equation of line l2 is y=2x-5.
View SolutionIf the graph of the function f(x)=ax−1xn(ax+1) is symmetrical about the y-axis ,then n equals (a)2 (b) 23 (c) 14 (d) 13
View SolutionA function y=f(x) has a second order derivative f''(x)=6(x−1). If its graph passes through the point (2, 1) and at that point the tangent to the graph is y=3x−5, then the function is
View Solution
