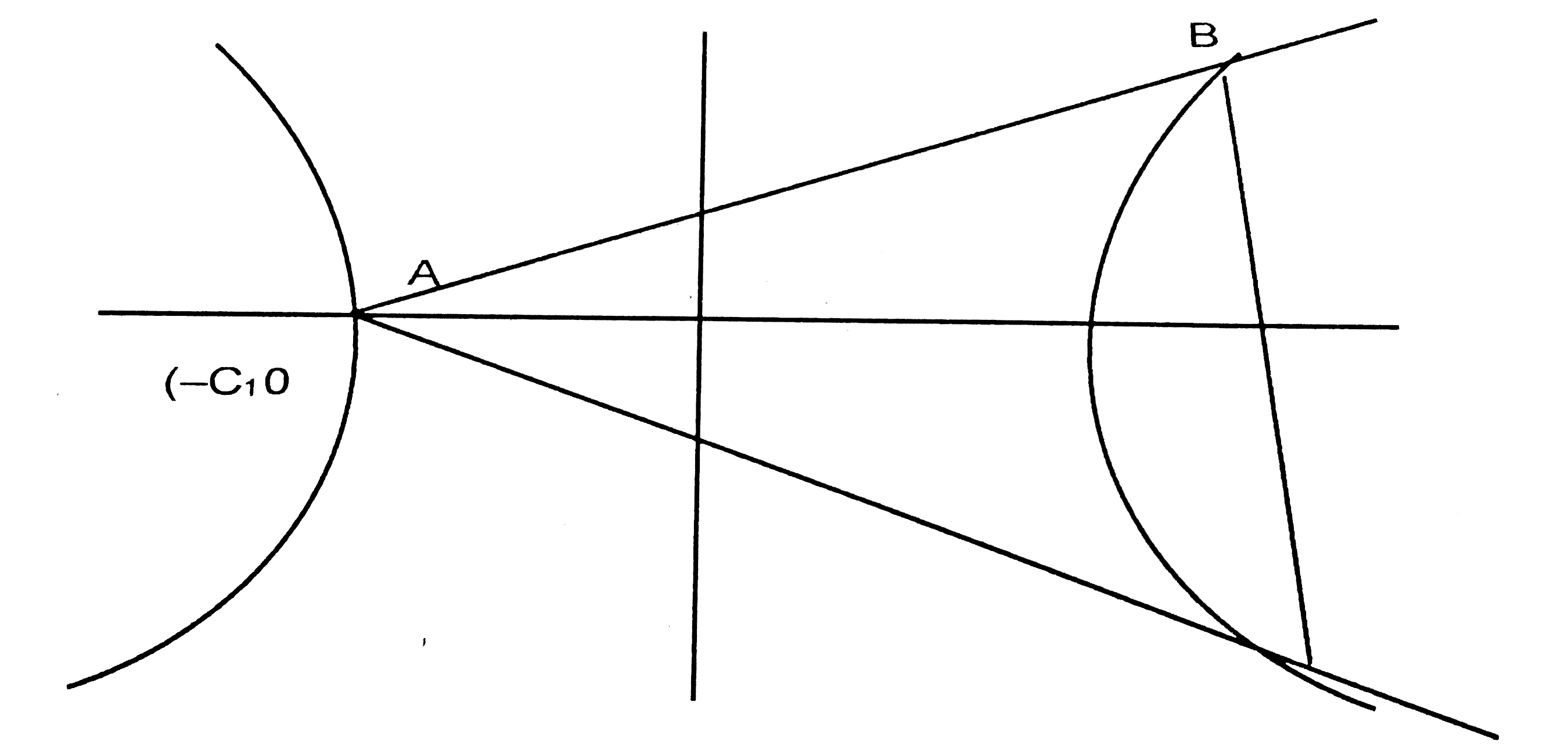
On a rectangular hyperbola $x^{2} - y^{2} = a^{2}, a > 0$ , three points A,B,C are taken as follows : A = (-a,0): B and C are placed symmetrically with respect to the x-axis on the branch of the hyperbola not containing A suppose that the triangle ABC is equilateral. If the side-length of the triangle ABC is ka,then k lies in the interval

A

(0,2]

B

(2,4]

C

(4,6]

D

(6,8]

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

$x^{2} - y^{2} = a^{2}$
A(-a,0)
$B (a sec θ, a tan θ)$
$C (a sec θ, - a tan θ)$
$M_{A B} = {tan 30}^{\circ} = \frac{a tan θ}{a sin θ + a} = \frac{1}{\sqrt{3}}$
$\sqrt{3} tan θ = 1 + sin θ$
$\sqrt{3} sin θ = 1 + sec θ$
${(\sqrt{3} tan θ - 1)}^{2} = {sec}^{2} θ$
$3 {tan}^{2} θ = 2 \sqrt{3} tan θ + 1 = 1 + {tan}^{2} θ$
$2 {tan}^{2} θ - 2 \sqrt{3} tan θ = 0$
$tan θ = \sqrt{3}$
Side length = $2 a tan θ$
$= 2 a \sqrt{3}$
$= 2 \sqrt{3} a$
$K = 2 \sqrt{3}$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Let D be the middle point of the side BC of a triangle ABC. If the triangle ADC is equilateral, then $a^{2} : b^{2} : c^{2}$ is equal to
A $1 : 4 : 3$
B $4 : 1 : 3$
C $4 : 3 : 1$
D $3 : 4 : 1$
Question 2 - Select One or More
If sides of triangle ABC are a, b, and c such that $2 b = a + c$ , then
A $\frac{b}{c} > \frac{2}{3}$
B $\frac{b}{c} > \frac{1}{3}$
C $\frac{b}{c} < 2$
D $\frac{b}{c} < \frac{3}{2}$
Question 3 - Select One or More
If $α, β, γ$ are the parameters of points A,B,C on the circle $x^{2} + y^{2} = a^{2}$ and if the triangle ABC is equilateral ,then
A $\sum cos α = 0$
B $\sum sin α = 0$
C $\sum tan α = 0$
D $\sum cot α = 0$

KVPY PREVIOUS YEAR-KVPY-PART-1 MATHEMATICS

Home
Ask Doubt
Profile