Let $x^{2} = 4 k y, k > 0$ be a parabola with vertex A. Let BC be its latus rectum. An ellipse with center on BC touches the parabola at A, and cuts BC at point D and E such that BD=DE=EC (B,D,E,C in that order). The eccentricity of the ellipse is

A

$\frac{1}{\sqrt{2}}$

B

$\frac{1}{\sqrt{3}}$

C

$\frac{\sqrt{5}}{3}$

D

$\frac{\sqrt{3}}{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

n For the parabola,
verter A(0,0)
Four F:(0,k)
end point of latus rectum:
B(-2k,k): c(2k,k)
Length of BC = 4k,
BD=DE=EC
And BD+DE+EC=4k
1 $\Rightarrow B D = D E = E C = \frac{4 k}{3}$ …….(i)

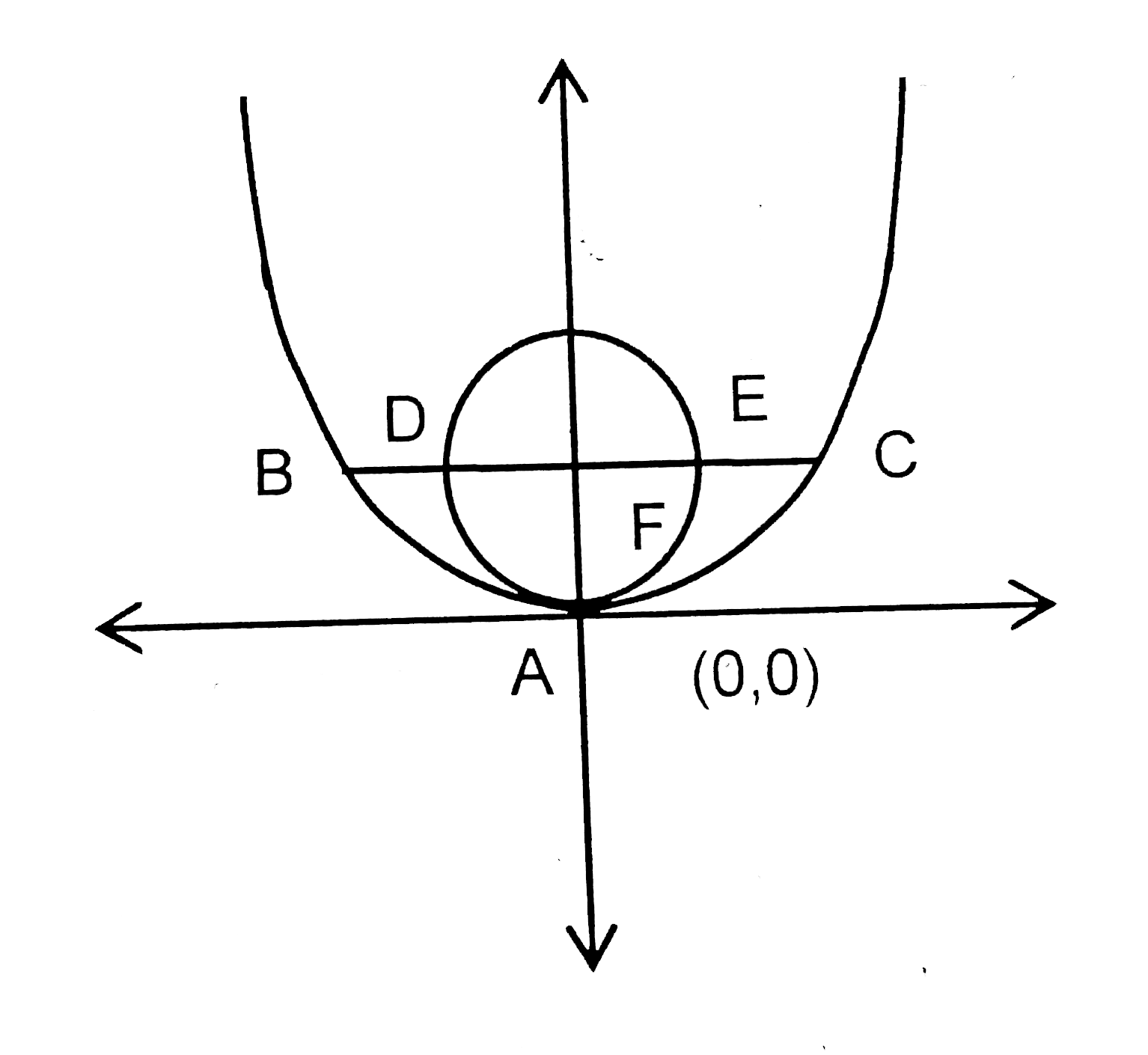
So major Axis of ellipse =2AF= $2 \times$ minor Axis of Elllipse = $D E = \frac{4 k}{3}$
Eccentricity = $C = \sqrt{1 - \frac{b^{2}}{a_{2}} = \sqrt{\frac{1 - {(\frac{2 k}{3})}^{2}}{k^{2}}} 2 = \frac{\sqrt{5}}{3}}$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
In $Δ A B C, ∠ B = 90^{\circ}$ . If points D and E are on side BC such that $B D = D E = E C$ , then which is true?
A $A E^{2} = 5 A C^{2} + 3 A D^{2}$
B $5 A E^{2} = 2 A C^{2} + 3 A D^{2}$
C $8 A E^{2} = 3 A C^{2} + 5 A D^{2}$
D $5 A E^{2} = 3 A C^{2} + 2 A D^{2}$
Question 2 - Select One
ABC is a triangle right- angled at B and D is a point on BC produced $(B D > B C)$ , such that $B D = 2 D C .$ Which one of the following is correct?
A $A C^{2} = A D^{2} - 3 C D^{2}$
B $A C^{2} = A D^{2} - 2 C D^{2}$
C $A C^{2} = A D^{2} - 4 C D^{2}$
D $A C^{2} = A D^{2} - 5 C D^{2}$
Question 3 - Select One
Let a, b and $λ$ positive real numbers. Suppoose P is an end point of the latus rectum of the parabola $y^{2} = 4 λ x$ and suppoose the ellipse $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1$ passes thorugh the point. P. if the tangents to the parabola and the ellipse at the point P are perpendicular to each other, then the eccentricity of the ellipse is
A $\frac{1}{\sqrt{2}}$
B $\frac{1}{2}$
C $\frac{1}{3}$
D $\frac{2}{5}$

KVPY PREVIOUS YEAR-KVPY-PART-1 MATHEMATICS

Home
Ask Doubt
Profile