Let OP=rand∠XOP=θ then the position of P in polar coordinate system is represented by (r,θ) OX and O are called the initial line and pole respectively.
If p be the length of perpendicular from origin to line and if α is the angle which the perpendicular to the line makes with the initial line . The equation of line is given by r cos (θ−α)=p
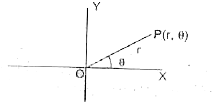
Let OP = r and angle XOP = theta then the position of P in polar coordinate system is represented by (r,theta) OX and O are called the initial line and pole respectively. If p be the length of perpendicular from origin to line and if alpha is the angle which the perpendicular to the line makes with the initial line . The equation of line is given by r cos (theta - alpha) = p Perpendicular distance from pole to the line l/r = 4 cos theta +2 sin theta is
Perpendicular distance from pole to the line lr=4cosθ+2sinθ is

A

2√5

B

l2√5

C

2√5l

D

none of these

Text Solution
Verified by Experts

The correct Answer is:B

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 12MATHSSTRAIGHT LINE

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Let OP=rand∠XOP=θ then the position of P in polar coordinate system is represented by (r,θ) OX and O are called the initial line and pole respectively.
    If p be the length of perpendicular from origin to line and if α is the angle which the perpendicular to the line makes with the initial line . The equation of line is given by r cos (θ−α)=p
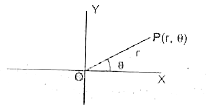
    ocr_image
    The equation of straight line x+√3y=1 in polar coordinate is

    Ar=12cos(θ+π6)
    B2r−cosec(θ+π6)
    Cr=sin(θ+π6)
    Dr=2cos(θ+π6)

  • Let OP=rand∠XOP=θ then the position of P in polar coordinate system is represented by (r,θ) OX and O are called the initial line and pole respectively.
    If p be the length of perpendicular from origin to line and if α is the angle which the perpendicular to the line makes with the initial line . The equation of line is given by r cos(θ−α)=p
    ocr_image
    The polar coordinates of the foot of perpendicular from the pole on the line joining the two points (r1,θ1)and(r2,θ2) are

    A⎡⎢ ⎢⎣r1r2sin(θ2−θ1)√r21+r22−2r1r2cos(θ1−θ2),tan−1(r1cosθ1−r2cosθ2r2sinθ2−r1sinθ2)⎤⎥ ⎥⎦
    B⎡⎢ ⎢⎣r1r2sin(θ2+θ1)√r21+r22−2r1r2cos(θ1−θ2),tan−1(r1cosθ1−r2cosθ2r2sinθ2−r1sinθ1)⎤⎥ ⎥⎦
    C⎡⎢ ⎢⎣−r1r2sin(θ2−θ1)√r21+r22−2r1r2cos(θ1−θ2),tan−1(r1cosθ1−r2cosθ2r2sinθ2−r1sinθ1)⎤⎥ ⎥⎦
    Dnone of these

  • The length of perpendicular from the origin to a line is 5 units and the line makes an angle 120∘ with the positive direction of x-axis. The equation of the line is

    Ax+√3y=5
    B√3x+y=10
    C√3x−y=10
    DNone of these