Doubtnut Promotions Sticky

While defining inverse trigonometric functions , a new system is followed where co-domains has been redefined as follows :
While defining inverse trigonometric functions , a new system is followed where co-domains has been redefined as follows : The minimum of (sin^(-1)x)^(3)+(cos^(-1)x))^(3) is equal to
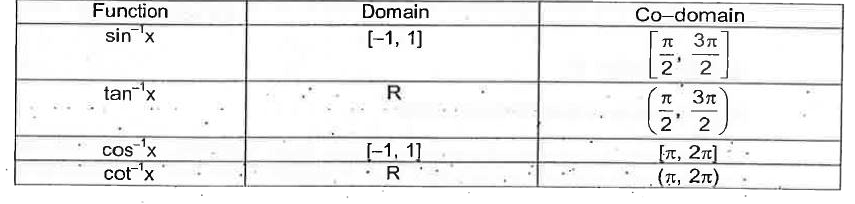
The minimum of (sin−1x)3+(cos−1x))3 is equal to

A

−sin−1x

B

π+sin−1x

C

2π−sin−1x

D

3π=−cos−1√1−x2x>0

Text Solution
Verified by Experts

The correct Answer is:a

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 12MATHSTRIGONOMETIC EQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    While defining inverse trigonometric functions , a new system is followed where co-domains has been redefined as follows :
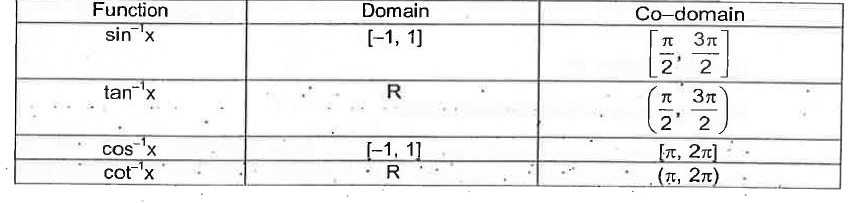
    ocr_image
    sin−1(−x) is equal to

    Anπ
    B(n+12)π
    C(n−12)π
    Dnone of these
  • Question 2 - Select One

    While defining inverse trigonometric functions , a new system is followed where co-domains has been redefined as follows :
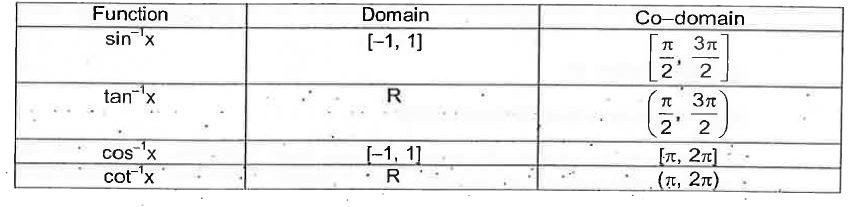
    ocr_image
    If f(X)=3sin−1x−2cos−1x then f(x) is

    Acos2xis35
    Bcos2xis12
    Ctan x is 12
    Dtan x is 13
  • Question 3 - Select One

    The domain of f(x)=√sin−1(3x−4x3)+√cos−1x is equal to

    A[−1,−√32]∪[0,√32]
    B[−1,−12]∪[0,12]
    C[0,12]
    DNone of these