The sets S1 and S2 are given by
even function
odd function
neither even nor odd
even as well as odd function
The correct Answer is:a
Topper's Solved these Questions
- TRIGONOMETIC EQUATIONS BOOK - FIITJEECHAPTER - TRIGONOMETIC EQUATIONS EXERCISE - COMPREHENSIONS III
2 Videos
- TRIGONOMETIC EQUATIONS BOOK - FIITJEECHAPTER - TRIGONOMETIC EQUATIONS EXERCISE - MATCH THE COLUMN
5 Videos
- TRIGONOMETIC EQUATIONS BOOK - FIITJEECHAPTER - TRIGONOMETIC EQUATIONS EXERCISE - COMPREHENSIONS I
3 Videos
- TRIGNOMETRIC RATIOS AND IDENTITIESBOOK - FIITJEECHAPTER - TRIGNOMETRIC RATIOS AND IDENTITIESEXERCISE - All Questions
1 Videos
- VECTORBOOK - FIITJEECHAPTER - VECTOREXERCISE - NUMERICAL BASED
4 Videos
Similar Questions
Let S1 be the set of all those solutions of the equation (1+a)cosθcos(2θ−b)=(1+a cos2θ)cos(θ−b) which are independent of a and b and S2 be the set of all such solutions which are dependent on a and b. The set S1and S2 are given by
View SolutionIn the given figure switches S1andS2 are in open condition. The resistance across ab when the switches S1andS2 are closed is ……………… Ω.
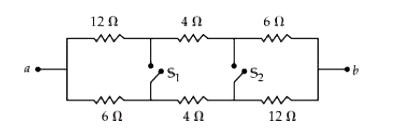
View Solution
Knowledge Check
The number of steps involved in S1N and S2N mechanisms are given by the set -
A1,2B3,1C2,1D2,2Let S1 be the set of all those solution of the equation (1+a)cosθcos(2θ−b)=(1+acos2θ)cos(θ−b)
which are independent of a and b and S2 be the set of all such solutions which are dependent on a and b. Then
The set S1 and S2 areA{nπ,n∈Z} and 12{nπ+(−1)nsin−1(asinb)+b,n∈Z}B{nπ2,n∈Z} and {nπ+(−1)nsin−1(asinb),n∈Z}C{nπ2,n∈Z} and {nπ+(−1)nsin−1(a2sinb),n∈Z}Dnone of theseConsider an ellipse x213+y24=1 and a variaote point P(1-,2t) is takne in such a way that ∠S1PS2 is maximum where S1andS2 are the foci of the given ellipse then the value of S1PS2P is
A√2√3B1√5C1√3D1√2
In the structure given below, the sites S1andS2 represent
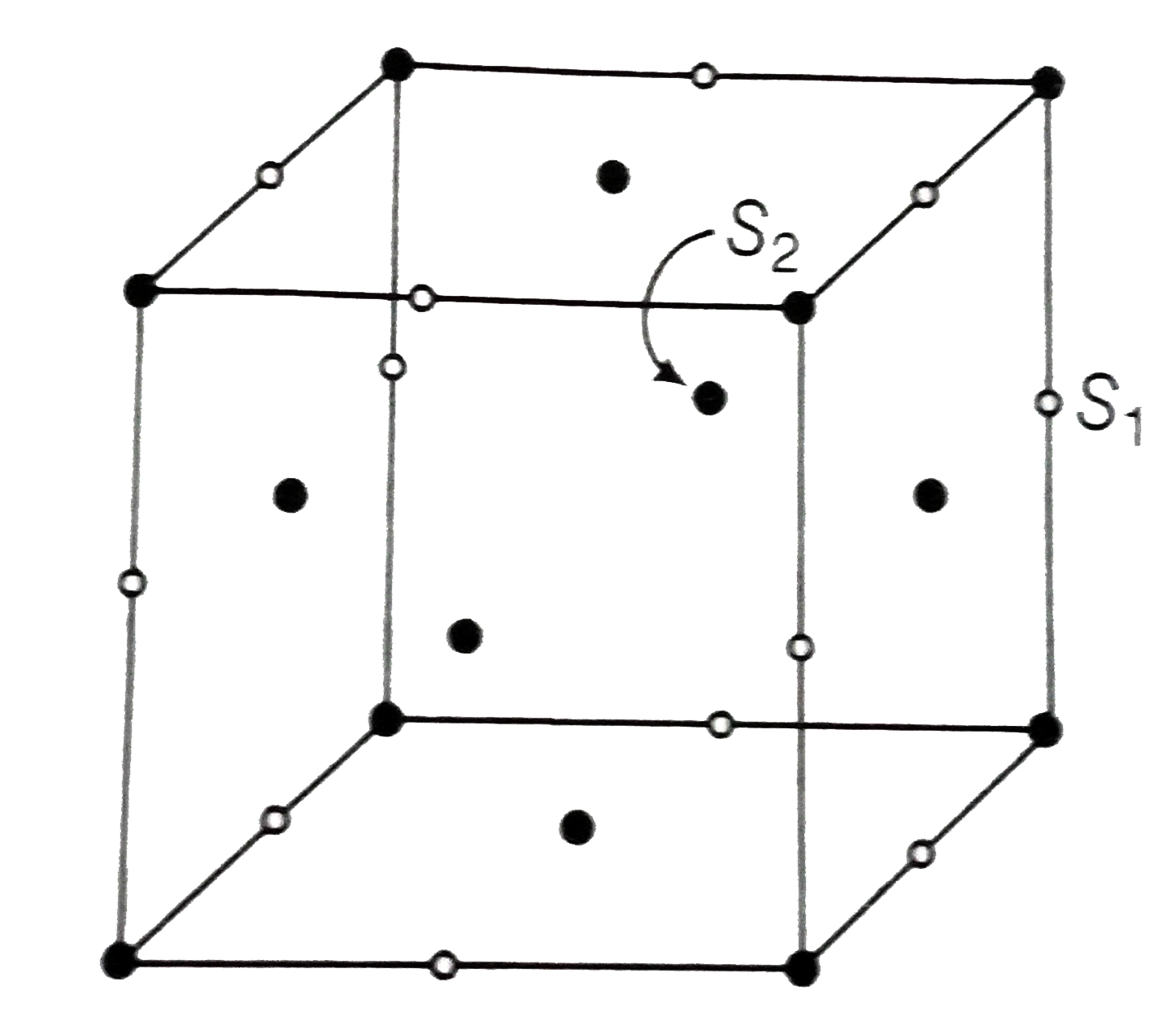
View SolutionIn a modified set up of Young's double slit experiment, it is given that SS2−SS1=λ4 i.e., the source S is not equidistant from the slits S1andS2.
(a) Obtain the condition for constructive and destructive interference at any point P on the screen in terms of the path difference Δ=S2P−S1P.
(b) Does the observed central bright fringe lie above or below O ? Give reason in support of your answer.
View SolutionLet S1 be the set of all those solution of the equation (1+a)cosθcos(2θ−b)=(1+acos2θ)cos(θ−b)
which are independent of a and b and S2 be the set of all such solutions which are dependent on a and b. Then
The set S1 and S2 are
View SolutionThe sets S1,S2,S3, .......... are given by S1={21},S2={32,52},S3={43,73103},S4={54,94,134,174},.. Then the sum of the numbers in the set S25 is 320 (b) 322 (c) 326 (d) 325
View SolutionFor any natural number n the sets S1,S2,….. are defined as below:
S1={1}.S2={2,3},S3={4,5,6}
S4={7,8,9,10},S5={11,12,13,14,15}: etc.
The sum of the elements of set S25 is :
View Solution
