Prove that the internal bisector of the angle A of a triangle ABC divides BC in the ratio AB:AC
To prove that the internal bisector of angle A in triangle ABC divides side BC in the ratio of AB:AC, we will follow these steps:
Step 1: Draw Triangle ABC
Let triangle ABC be given, where A is the vertex of the angle we are bisecting, and B and C are the other two vertices.
Hint: Start by clearly labeling the triangle and its vertices.
Step 2: Draw the Angle Bisector
Draw the internal bisector of angle A, and let it intersect side BC at point D.
Hint: Ensure that the angle bisector divides angle A into two equal angles.
Step 3: Apply the Angle Bisector Theorem
According to the Angle Bisector Theorem, the internal bisector of an angle in a triangle divides the opposite side into segments that are proportional to the lengths of the other two sides. Therefore, we can write:
BDDC=ABAC
Hint: Remember that BD is the segment of BC adjacent to vertex B, and DC is the segment adjacent to vertex C.
Step 4: Express the Ratios
Let AB=c and AC=b. Then we can express the ratio as:
BDDC=cb
Hint: Use variables for the sides to simplify the notation.
Step 5: Conclusion
Thus, we have shown that the internal bisector of angle A divides side BC in the ratio of AB to AC, which is what we wanted to prove.
Hint: Restate the conclusion clearly to emphasize that the proof is complete.
Final Statement
Therefore, the internal bisector of angle A in triangle ABC divides side BC in the ratio of AB:AC.
---
Let triangle ABC be given, where A is the vertex of the angle we are bisecting, and B and C are the other two vertices.
Draw the internal bisector of angle A, and let it intersect side BC at point D.
According to the Angle Bisector Theorem, the internal bisector of an angle in a triangle divides the opposite side into segments that are proportional to the lengths of the other two sides. Therefore, we can write:
Let AB=c and AC=b. Then we can express the ratio as:
Thus, we have shown that the internal bisector of angle A divides side BC in the ratio of AB to AC, which is what we wanted to prove.
Therefore, the internal bisector of angle A in triangle ABC divides side BC in the ratio of AB:AC.
Topper's Solved these Questions
- VECTORBOOK - FIITJEECHAPTER - VECTOREXERCISE - EXERCISE 7
2 Videos
- VECTORBOOK - FIITJEECHAPTER - VECTOREXERCISE - ASSIGNMENT PROBLEMS (SUBJECTIVE) LEVEL-1
15 Videos
- VECTORBOOK - FIITJEECHAPTER - VECTOREXERCISE - EXERCISE 5
2 Videos
- TRIGONOMETIC EQUATIONS BOOK - FIITJEECHAPTER - TRIGONOMETIC EQUATIONS EXERCISE - NUMERICAL BASED
3 Videos
Similar Questions
Knowledge Check
Assertion (A) : AD is angle bisector of ∠A of the triangle ABC. If AB = 6 cm, BC = 7 cm, AC = 8 cm then BD = 3 cm and CD = 4 cm.
Reason (R) : The angle bisector AD of the triangle divides base BC in the ratio AB : AC.ABoth Assertion A and Reason R are correct and Reason R is a correct explanation of Assertion A.BBoth Assertion A and Reason R are correct but Reason R is not the correct explanation of Assertion A.CAssertion A is correct, Reason R is wrong.DAssertion A is wrong, Reason R is correct.The internal /external bisector of an angled of a triangle divides the opposite side internally / externally in the ratio of the sides containing the angle .
In the given figure ,Ae is the bisector of the exterior ∠CAD meeting BC produced in E . If AB=15 cm , AC = 8 cm and BC =14 cm , find CE .
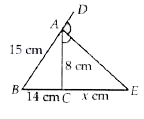 A12 cmB16 cmC20 cmD18 cm
A12 cmB16 cmC20 cmD18 cmIn a triangle ABC, D is a point on BC such that AD is the internal bisector of ∠A. Let ∠B=2∠C and CD = AB. Then ∠A is
A18∘B36∘C54∘D72∘
The internal angle bisector of an angle of a triangle divide the opposite side internally in the ratio of the sides containgthe angle
View SolutionIf the bisector of the angle A of triangle ABC meets BC in D, prove that a=(b+c)[1−[AD]2bc]12
View SolutionIf the bisector of angle A of the triangle ABC makes an angle θ with BC, then sinθ=
View SolutionIn a ΔABC, it is give ttha AD is the internal bisector of ∠A. If AB=10cm,AC=14cmandBC=6cm then CD=?

View SolutionIn triangle ABC, AB = 12 cm, ∠B=60∘, the perpendicular from A to BC meets it at D. The bisector of ∠ABC meets AD at E. Then E divides AD in the ratio
View Solution
