Let P be a point in the plane of ΔABC such that the triangles PAB,PCA all have the same perimeter and the same area.
If P lies outside the ΔABC, then ΔABC
must be equilateral
may not be equilateral
must be right angled
none of these
The correct Answer is:C
Topper's Solved these Questions
- MATHEMATICS BOOK - FIITJEECHAPTER - MATHEMATICS EXERCISE - NUMERICAL BASED QUESTIONS
23 Videos
- MATHEMATICS BOOK - FIITJEECHAPTER - MATHEMATICS EXERCISE - NUMERICAL DECIMAL BASED QUESTIONS
15 Videos
- MATHEMATICS BOOK - FIITJEECHAPTER - MATHEMATICS EXERCISE - MCQ (MULTIPLE CORRECT)
30 Videos
- MATHEMATICAL REASONING BOOK - FIITJEECHAPTER - MATHEMATICAL REASONING EXERCISE - ASSIGNMENT PROBLEMS (OBJECTIVE) LEVEL-2
18 Videos
- MATHEMATICS TIPSBOOK - FIITJEECHAPTER - MATHEMATICS TIPSEXERCISE - NUMERICAL DECIMAL BASED QUESTIONS
21 Videos
Similar Questions
A triangle ABC is formed by three lines x+y+2=0,x−2y+5−0and7x+y−10=0. P is a point inside the triangle ABC such that areas of the triangles PAB, PBC and PCA are equal. If the co-ordinates of the point P are (a, b) and the area of the triangle ABC is δ, then find (a+b+δ).
View SolutionLet A(5,−3),B(2,7)andC(−1,2) be the vertices of a △ABC. If P is a point inside the triangle ABC such that the triangle APC,APB and BPC have equal areas, then length of the line segment PB is:
View Solution
Knowledge Check
Let P be a point in the plane of ΔABC such that the triangles PAB,PCA all have the same perimeter and the same area.
If P lies inside the ΔABC, then ΔABCAmust be equilateralBmay not be equilateralCmust be right angledDnone of theseLet P be a point in the plane of ΔABC such that the triangles PAB,PCA all have the same perimeter and the same area.
If P lies outside the ΔABC, then the quadrialteral formed by A, B, C and P is necessarilyArectangleBsquareCrhombusDnone of theseLet P(x,y) be a point inside the equilateral ΔABC such that d(P, AB) + d(P, AC) + d(P, BC) = K where k is the altitude of the Δ then
Statement - 1 area of the region of p (x,y) or of (ΔABC)
Statement - 2, For equilateral tringle if d (P,AB) + d(P,AC) + d(P,BC) = k then P will lie inside the pedal triangle of ΔABCAStatement - 1 is True, statement -2 is true, statement-2 is a correct explanation fro statement - 1BStatement - 1 is True. Statement - 2 is true statement -2 is Not a correct explanation for statement -1CStatement -1 is True, statement -2 is FalseDStatement -1 is False, Statement -2 is True
Let A(5,−3),B(2,7)andC(−1,2) be the vertices of a △ABC. If P is a point inside the triangle ABC such that the triangle APC,APB and BPC have equal areas, then length of the line segment PB is:
View SolutionG is the centroid of the triangle ABC. Prove that the area of the triangle ABG is one third of the area of the triangle ABC.
View SolutionIn Fig. 9.29 ratio of the area of triangle ABC to the area of triangle ACD is the same as the ratio of base BC of triangle ABC to the base CD of triangle ACD.
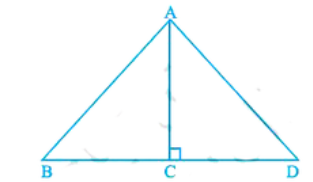
View SolutionIn A ΔABC,AD,BEandCF are three medians . The perimeter of ΔABC is always
View SolutionIf the perimeter of the triangle formed by feet of altitudes of the triangle ABC is equal to four times the circumradius of ΔABC, then identify the type of ΔABC
View Solution
