A five digit number (having all different digits) is formed using the digits 1,2,3,4,5,6,7,8and9. The probability that the formed number either begins or ends with an odd digit, is equal to
56
16
13
23
The correct Answer is:A
Step by step video, text & image solution for A five digit number (having all different digits) is formed using the digits 1, 2, 3, 4, 5, 6, 7, 8 and 9. The probability that the formed number either begins or ends with an odd digit, is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - MATHEMATICS TIPSEXERCISE - ASSIGNMENT -OBJECTIVE84videos
MATHEMATICS TIPS
- BOOK - FIITJEECHAPTER - MATHEMATICS TIPSEXERCISE - ASSERTION - REASONING8videos
MATHEMATICS TIPS
- BOOK - FIITJEECHAPTER - MATHEMATICS TIPSEXERCISE - NUMERICAL DECIMAL BASED QUESTIONS21videos
MATHEMATICS TIPS
- BOOK - FIITJEECHAPTER - MATHEMATICS EXERCISE - NUMERICAL DECIMAL BASED QUESTIONS15videos
MATHEMATICS
- BOOK - FIITJEECHAPTER - MATRICES EXERCISE - NUMERICAL BASED3videos
MATRICES
Similar Questions
Knowledge Check
- Question 1 - Select One
A five digit number if formed by the digits 1,2,3,4,5,6 and 8. The probability that the number has even digit at both ends is
A2/7B3/7C4/7Dnone - Question 2 - Select One
A number of 3 different digits is formed from the digits 0, 1, 2, 3, 4, 5, 6 Probability that the number formed is '340' is
A1/180B7/180C111/180D113/180 - Question 3 - Select One
Write the largest number of different digits formed by using the digits 2,4,0,3,6 and 9.
A996432B964322C964302D964320
Using the digits 1, 2, 3, 4, 5, 6,7, a number of 4 different digits is formed. Find
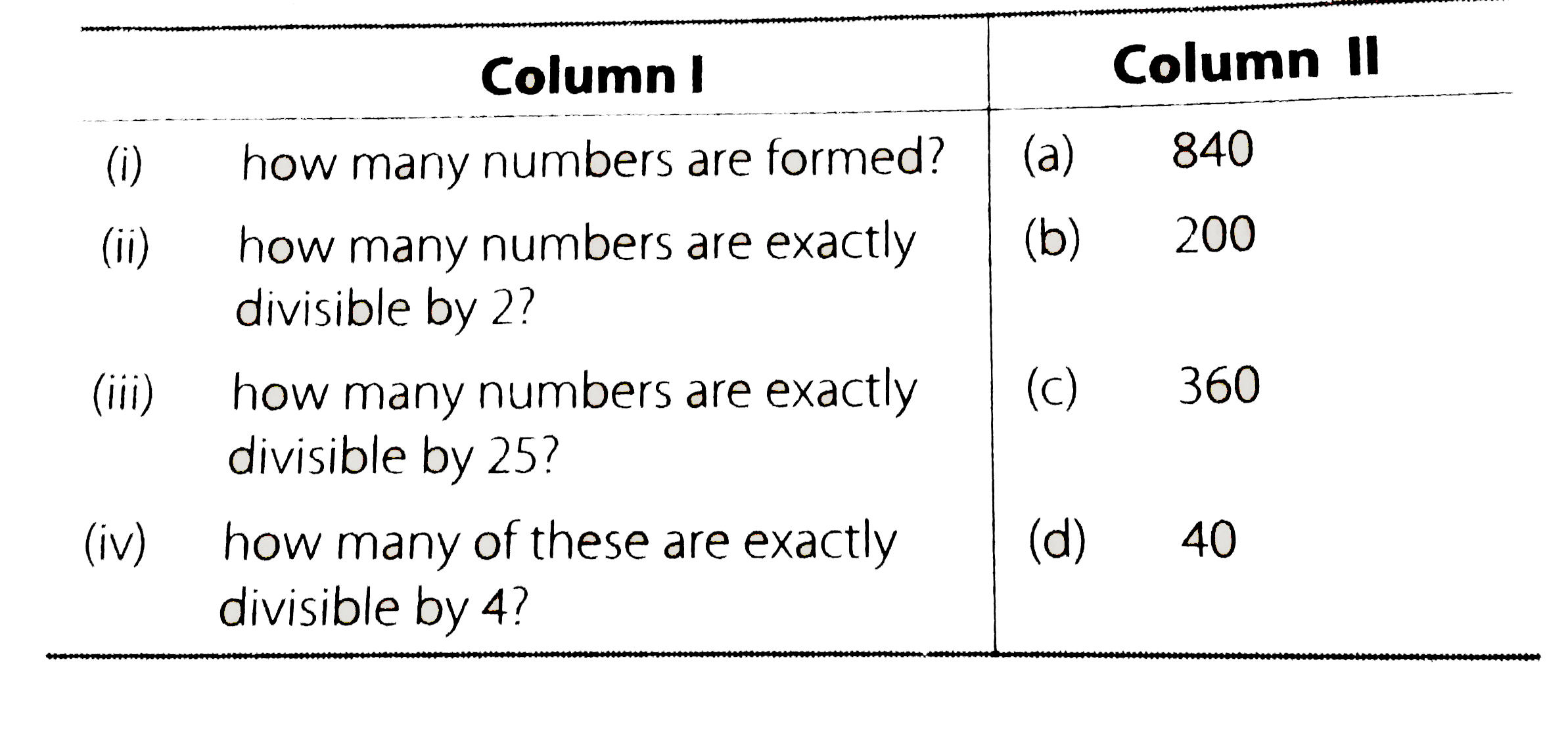 View Solution
View SolutionA five digit number if formed by the digits 1,2,3,4,5,6 and 8. The probability that the number has even digit at both ends is
View SolutionA four digit number is formed by the digits 1, 2, 3, 4 with no repetition. The probability that the number is odd is
View SolutionA nine - digit number is formed using the digits 1, 2, 3, 5 and 7. The probability that the product of all digits is always 1920 is
View SolutionA four -digit number is formed by the digits 1,2,3,4 with no repetition. The probability that the number is odd ,is
View Solution

