A projectile is projected from top of a wall 20m height with a speed of 10m/s horizontally. Find range (g=10ms2)
Step by step video & image solution for A projectile is projected from top of a wall 20m height with a speed of 10m/s horizontally. Find range (g = 10m/s^(2)) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
A projectile is projected from horizontal ground with velocity 18km/hr at an angle of 60∘ from horizontal. Find angular speed (in rad/s) as observed from the point of projection at the time of landing.[ g=10m/s2]
View SolutionA particle is projected at an angle 60∘ with horizontal with a speed v=20m/s. Taking g=10m/s2. Find the time after which the speed of the particle remains half of its initial speed.
View Solution
Knowledge Check
A projectile is projected with the initial velocity (6i+8j)m/s. The horizontal range is (g=10m/s2)
A96mB960mC9.6mD4.8mA particle is projected with velocity of 10ms at an angle of 15° with horizontal.The horizontal range will be(g=10ms2)
A10mB5mC2.5mD1mA particle is projected at an angle 60∘ with the horizontal with a speed 10m/s. Then, latus rectum is (Take g=10m/s2)
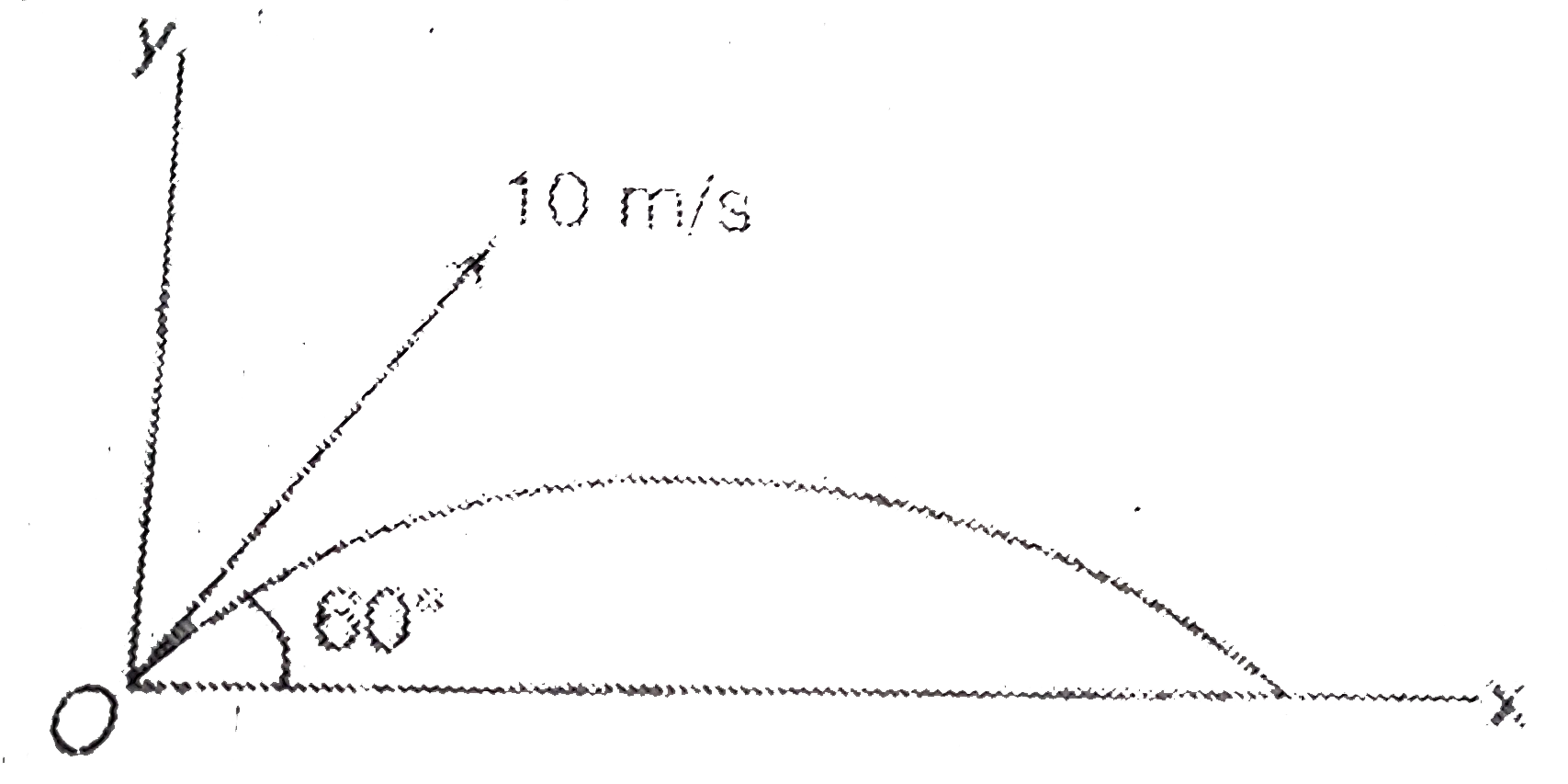 A5mB15mC10mD0
A5mB15mC10mD0
A projectile is projected with a speed = 20 m/s from the floor of a 5 m high room as shown. Find the maximum horizontal range of the projectile and the corresponding angle of projection θ.
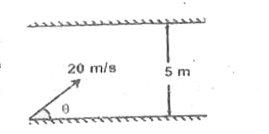
View SolutionWhat are the two angles of projection of a projectile projected with velocity of 30m/s, so that the horizontal range is 45m. Take, g=10m/s2.
View SolutionFrom the ground, a projectile is fired at an angle of 60 degree to the horizontal with a speed of 20 m s−1 The horizontal range of the projectile is
View SolutionA particle of mass 50 g is projected horizontally from the top of a tower of height 50 m with a velocity 20m/s. If g=10m/s2, then find the :-
View SolutionA ball is projected vertically up with speed 20 m/s. Take g=10m/s2
View Solution
