Question

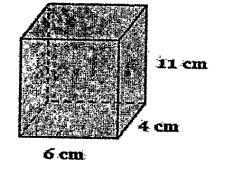
Volume of prism

Explanation

Prism

In this article, we will focus on prism. Students would also be able to learn about the volume of the prism.  We would also work on the examples of the prism where it would make things quite clear to you.

What is Prism?

The three-dimensional solid object whose two ends are exactly of the same shape is the prism. It is the combination of the identical bases, flat faces and same cross-sections. Without the bases, the faces are parallelograms. In case you wish to take the cross-section or the prism parallel to the base, then you would find that the cross-sections would look like the bases.

What is the shape of the prism?

A prism has got a solid shape where it consists of two equal ends as well as identical cross-section and flat faces or surfaces. It is also called triangular prism as the cross-section looks like a triangle. The shape of the prism does not have any curve. Therefore, it should be kept in mind that a prism can be rectangular, cubic, pentagonal as well as other polygon shapes, excluding the circular shape.

What are the types of prisms?

Based on the cross-sections, the prisms are of two types which are Regular Prism and Irregular Prism. We will have a look at these types of prisms as below:

• Regular Prism:  A regular prism is the one where the bases of the prism are in the shape of a regular polygon.


• Irregular Prism: An irregular prism has the base in the shape of an irregular polygon.

The classification of the prism can be further done into two types which are Right Prism and Oblique Prism

• Right Prism: Right prism is the one whose faces and the joining edges are perpendicular to the base faces. Here the side faces are rectangle.


• Oblique Prism: In this type of prism, the faces and the joining edges are not perpendicular to the base faces. The side faces are parallelograms.

Based on the shape of the bases, prisms are further classified as below:

• Rectangular


• Pentagonal


• Triangular


• Square


• Hexagonal

Prism Formulas

It is based on the surface area and volume of the prism for which the formulas are defined. It is because of the three-dimensional shape, the prism has both the properties, which are volume and surface area.

Surface Area of a Prism

The total area which is covered by the faces of the prism is the surface area of the prism.

So, the formula of the Surface Area of a Prism = 2(Base Area) + (Base perimeter × length)

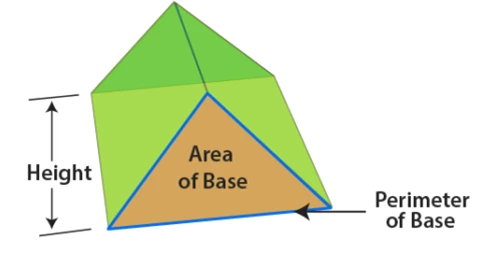
What is the Volume of a Prism?

The total space which is occupied by the three-dimensional object is called the volume of a prism. So, in math, it is the product of the area of the base and the length.

Therefore, the volume of a Prism = Base Area × Length

Volume of a Prism Formula

Now, we will discuss the formula of the volume of a prism. Here, we will concentrate on the rectangular prism, triangular prism, pentagonal prism, and so on.

Volume of a Rectangular prism

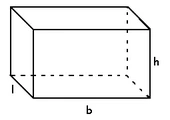
There are four rectangular faces and two parallel rectangular bases in a rectangular prism. The rectangular prism is also called “Cuboid”.

So, the volume of a Rectangular Prism = lbh cubic units.

Here,

l = Base width of a rectangular prism

b = Base length of a rectangular prism

h = height of a rectangular prism

Volume of a Triangular Prism

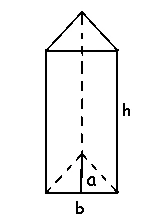
A triangular prism has got three rectangular faces and two triangular bases. The formula for the volume of a triangular prism is given as:

The volume of a Triangular Prism = (½) abh cubic units.

Here

a = Apothem length of a triangular prism

b = Base length of a triangular prism

h = height of a triangular prism

Volume of a Pentagonal Prism

There are five rectangular faces and two parallel pentagonal bases in a Pentagonal Prism.

So, the Volume of a Pentagonal Prism = (5/2) abh cubic units

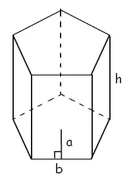
Here,

a = Apothem length of the pentagonal prism.

b = Base length of the pentagonal prism.

h = Height of the pentagonal prism

Volume of a Hexagonal Prism

There are six rectangular faces and two parallel hexagonal bases in a hexagonal prism.

The volume of a Hexagonal Prism = 3abh cubic units

Here,

a = Apothem length of the hexagonal prism.

b = Base length of the hexagonal prism.

h = Height of the hexagonal prism.

Related Videos:

• Area and Perimeter of rectangle


• Area and Perimeter of square


• Area and Perimeter of triangle


• Area and Perimeter of trapezium


• Area and Perimeter of rhombus


• Area and Perimeter of circle

We will concentrate on some of the examples of prism to get some good understanding about it.

Example 1: The area of a triangular prism is 50 cm² and height is 8 cm? Can you find the volume?

Solution: Given,

Area = 50 cm²

Height = 8 cm

We are familiar that,

(V) volume of a prism = Base area × Height cubic units

So, V = 50 × 8 = 400

Therefore, the volume of a triangular prism = 400 cm³.

Example 2:  The volume of a square is 400 cm³ and the base area is 80 cm²? Can you calculate its height?

Solution: Given,

The volume of a square prism = 400cm³

Base Area = 80cm²

Now by calculating the height of the square prism, we get,

The volume of square prism = Base area × height

400 = 80 × prism height

So, the height, h = 400/80

So, the height of the prism, h = 5 cm.

Example 3: A right prism has a height of 12 cm. It stands on a base which is a regular hexagon. The area of the whole surface of the prism is 1152√3 sq.cm.  Can you find the volume of the prism?

Solution: Let us take 'a' be the length of a side of the regular hexagon. Here, the area of its base = area of the regular hexagon and the area of side-faces of the prism

= 6a . 12 sq. cm. sq. cm. and

= (6a²/4) cot (π/4), [since, the area of a regular polygon of n sides = (na²)/4 cot (π/5)]

= {(3√3)/2}a² sq,. cm.

According to problem, the area of the whole surface of the prism = 1152√3

Therefore, 6a ∙ 12 + 2 ∙ {(3√3)/2}a² = 1152√3

Or, a² + 8√3 a - 384 = 0

Or, a² + 16√3 a - 8√3 a - 384 = 0

Or, a(a + 16√3) – 8√3 (a + 16√3 a) = 0

Or, (a + 16√3 a)(a - 8√3) = 0

Therefore, either, a = - 16√3 or, a = 8√3

Since a is positive , the value of a = -16√3 is admissible.

Therefore, we take a = 8√3

Therefore, the required volume of the prism

= area of its base × its height

= (3√3)/2 ∙ (8√3)² ∙ 12 c.c.

= 3456√3 c.c.

Example 4:  The area of a triangular prism is 40 cm² and height is 6 cm? Can you find the volume?

Solution: Given,

Area of prism = 40 cm²

Height = 6 cm

We are familiar that,

(V) volume of a prism = Base area × Height cubic units

So, V = 60 × 4 = 240

Therefore, the volume of a triangular prism = 240 cm³.

Important Chapters:

• NCERT Solutions for Maths


• NCERT Solution for Class 6 Maths Chapter Mensuration


• NCERT Solution for Class 8 Maths Chapter Mensuration