The equations of $L_{1} and L_{2}$ are $y = m x and y = n x,$ respectively. Suppose $L_{1}$ make twice as large of an angle with the horizontal (measured counterclockwise from the positive x-axis) as does $L_{2}$ and that $L_{1}$ has 4 times the slope of $L_{2} .$ If $L_{1}$ is not horizontal, then the value of the product $(m n)$ equals:

Video Solution

Answer

Step by step video & image solution for The equations of L_1 and L_2 are y = mx and y = nx, respectively. Suppose L_1 make twice as large of an angle with the horizontal (measured counterclockwise from the positive x-axis) as does L_2 and that L_1 has 4 times the slope of L_2. If L_1 is not horizontal, then the value of the product (mn) equals: by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Similar Questions

Given equation of line $L_{1}$ is $y = 4$

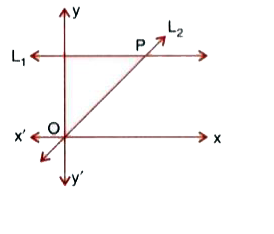
Write the slope of line $L_{1}$ if $L_{2}$ is the bisector of angle O.
01:33
View Solution
If $L_{1} & a m p; L_{2}$ are the lengths of the segments of any focal chord of the parabola $y^{2} = x,$ then (a) $\frac{1}{L_{1}} + \frac{1}{L_{2}} = 2$ (b) $\frac{1}{L_{1}} + \frac{1}{L_{2}} = \frac{1}{2}$ (c) $\frac{1}{L_{1}} + \frac{1}{L_{2}} = 4$ (d) $\frac{1}{L_{1}} + \frac{1}{L_{2}} = \frac{1}{4}$
07:24
View Solution
The line $l_{1} x + m_{1} y + n = 0 and l_{2} x + m_{2} y + n = 0$ will cut the coordinate axes at concyclic points if (A) $l_{1} m_{1} = l_{2} m_{2}$ (B) $l_{1} m_{2} = l_{2} m_{1}$ (C) $l_{1} l_{2} = m_{1} m_{2}$ (D) $l_{1} l_{2} m_{1} m_{2} = n^{2}$
05:56
View Solution
If $l_{1} = \int_{e}^{e^{2}} \frac{d x}{log x}$ and $l_{2} = \int_{1}^{2} \frac{e^{x}}{x} d x$ , then (A) $l_{1} = 2 l_{2}$ (B) $l_{1} + l_{2} = 0$ (C) $2 l_{1} = l_{2}$ (D) $l_{1} = l_{2}$
01:31
View Solution
If $l_{1} : \frac{x - 5}{3} = \frac{y - 7}{- 16} = \frac{z - 3}{7} and l_{2} : \frac{x - 9}{3} = \frac{y - 13}{8} = \frac{z - 15}{- 5}$ the (A) $l_{1} and l_{2}$ intersect (B) $l_{1} and l_{2}$ are skew (C) distance between $l_{1} and l_{2}$ is 14 (D) none of these
08:19
View Solution
Lines $L_{1}$ and $L_{2}$ have slopes m and n, respectively, suppose $L_{1}$ makes twice as large angle with the horizontal (mesured counter clockwise from the positive x-axis as does $L_{2}$ and $L_{1}$ has 4 times the slope of $L_{2}$ . If $L_{1}$ is not horizontal, then the value of the proudct mn equals.
03:37
View Solution
Is the relation $R = {(L_{1}, L_{2}) : L_{1}, L_{2} \in L}$ and $L_{1}$ is perpendicular on $L_{2}$ an equivalence relation in the set L of all straight lines in the coordinate plane?
05:02
View Solution
theta_1 and theta_2 are the inclination of lines L_1 and L_2 with the x-axis. If L_1 and L_2 pass through P(x_1,y_1) , then the equation of one of the angle bisector of these lines is
05:46
View Solution

The equations of L1andL2 are y=mxandy=nx, respectively. Suppose L1 make twice as large of an angle with the horizontal (measured counterclockwise from the positive x-axis) as does L2 and that L1 has 4 times the slope of L2. If L1 is not horizontal, then the value of the product (mn) equals:

Similar Questions

Recommended Questions