Two blocks of masses 2 kg and 4 kg tied to a string passing over a frictionless pulley. What will be the magnitude of acceleration of the masses, when they are allowed to move freely?
To find the magnitude of acceleration of the two blocks tied to a string passing over a frictionless pulley, we can follow these steps:
Step 1: Identify the masses and forces
We have two blocks:
- Mass m1=2kg (upward)
- Mass m2=4kg (downward)
The forces acting on the blocks due to gravity are:
- Weight of m1: W1=m1⋅g=2⋅g
- Weight of m2: W2=m2⋅g=4⋅g
Where g is the acceleration due to gravity (approximately 9.81m/s2).
Step 2: Write the net force equation
The net force acting on the system can be calculated by taking the difference between the weights of the two blocks:
Fnet=W2−W1=4g−2g=2g
Step 3: Calculate the total mass of the system
The total mass of the system is the sum of the masses of the two blocks:
mtotal=m1+m2=2kg+4kg=6kg
Step 4: Apply Newton's second law
According to Newton's second law, the acceleration a of the system can be calculated using the formula:
a=Fnetmtotal
Substituting the values we found:
a=2g6
Step 5: Substitute the value of g
Using g≈9.81m/s2:
a=2⋅9.816=19.626≈3.27m/s2
Conclusion
The magnitude of the acceleration of the masses when they are allowed to move freely is approximately 3.27m/s2.
---
We have two blocks:
- Mass m1=2kg (upward)
- Mass m2=4kg (downward)
- Weight of m1: W1=m1⋅g=2⋅g
- Weight of m2: W2=m2⋅g=4⋅g
The net force acting on the system can be calculated by taking the difference between the weights of the two blocks:
Fnet=W2−W1=4g−2g=2g
The total mass of the system is the sum of the masses of the two blocks:
mtotal=m1+m2=2kg+4kg=6kg
According to Newton's second law, the acceleration a of the system can be calculated using the formula:
a=Fnetmtotal
Substituting the values we found:
a=2g6
Using g≈9.81m/s2:
a=2⋅9.816=19.626≈3.27m/s2
The magnitude of the acceleration of the masses when they are allowed to move freely is approximately 3.27m/s2.
Similar Questions
Two blocks of masses 2 kg and 4kg tied to a spring passing over a frictionless pulley . What will br the magnitude of acceleration of the masses, when they are allowed to move freely ?
View SolutionTwo spheres of masses 2.6 kg and 4.1 kg are tied together by a light string looped over a frictionless pulley.
(i) What will the acceleration of each mass be?
(ii) Find the value of tension in the string.
View Solution
Knowledge Check
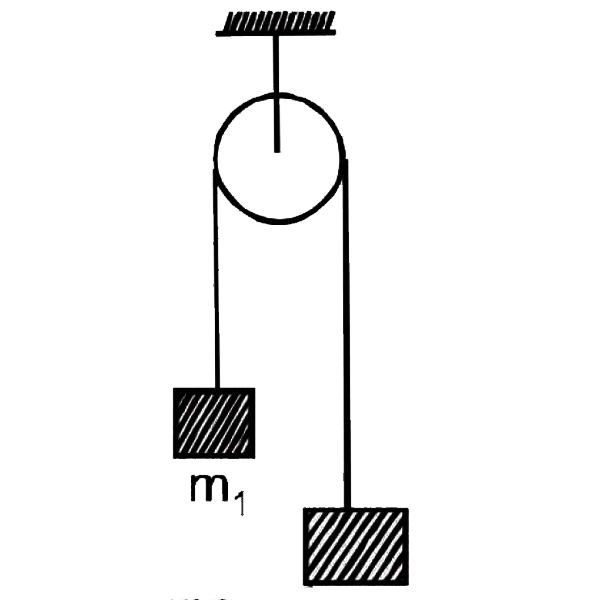
Two masses m1=5kg and m2=4.8kg tied to a string are hanging over a light frictionless pulley. What is the acceleration of the masses when left free to move?
 A(a) 5m/s2B(b) 9.8m/s2C(c) 0.2m/s2D(d) 4.8m/s2
A(a) 5m/s2B(b) 9.8m/s2C(c) 0.2m/s2D(d) 4.8m/s2Two blocks of masses 3 kg and 6 kg are connected by a string as shown in the figure over a frictionless pulley. The acceleration of the system is
 A4m/s2B2m/s2CzeroD6m/s2
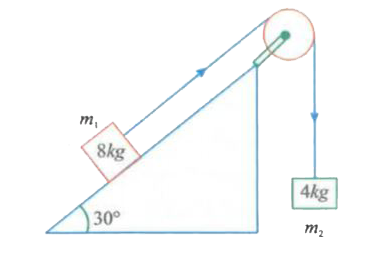
A4m/s2B2m/s2CzeroD6m/s2Two masses of 8 kg and 4 kg are connected by a string as shown in figure over a frictionless pulley. The acceleration of the system is
 A4m/s2B2m/s2CzeroD9.8m/s2
A4m/s2B2m/s2CzeroD9.8m/s2
Two bodies of mass 5 kg and 7 kg are suspended at the ends of massless string passing over frictionless pulley. The acceleration of the system.
View SolutionTwo masses of 8kg and 4kg are connected by a string as shown in figure over a frictionless pulley.The acceleration of the system is

View SolutionTwo blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.

View SolutionTwo bodies of masses 4 kg and 6 kg are tied to the ends of a massless string. The string passes over a frictionless pulley. The acceleration of the system is :
View SolutionTwo blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.

View Solution
