Two masses 2 kg and 4 kg are connected at the two ends of light inextensible string passing over a frictionless pulley. If the masses are released, then find the acceleration of the masses and the tension in the string.
To solve the problem, we need to find the acceleration of the two masses and the tension in the string connecting them. We have two masses: m1=2kg and m2=4kg.
Step 1: Identify the Forces
When the masses are released, the force acting on each mass due to gravity can be calculated as follows:
- For m1 (2 kg), the weight W1=m1⋅g=2⋅10=20N (acting upwards).
- For m2 (4 kg), the weight W2=m2⋅g=4⋅10=40N (acting downwards).
Step 2: Apply Newton's Second Law
According to Newton's second law, the net force acting on the system is equal to the mass of the system multiplied by its acceleration.
The net force acting on the system can be expressed as:
Fnet=W2−W1=40N−20N=20N
The total mass of the system is:
mtotal=m1+m2=2kg+4kg=6kg
Now we can find the acceleration a of the system using the formula:
Fnet=mtotal⋅a
Substituting the values we have:
20N=6kg⋅a
Solving for a:
a=20N6kg=103≈3.33m/s2
Step 3: Calculate the Tension in the String
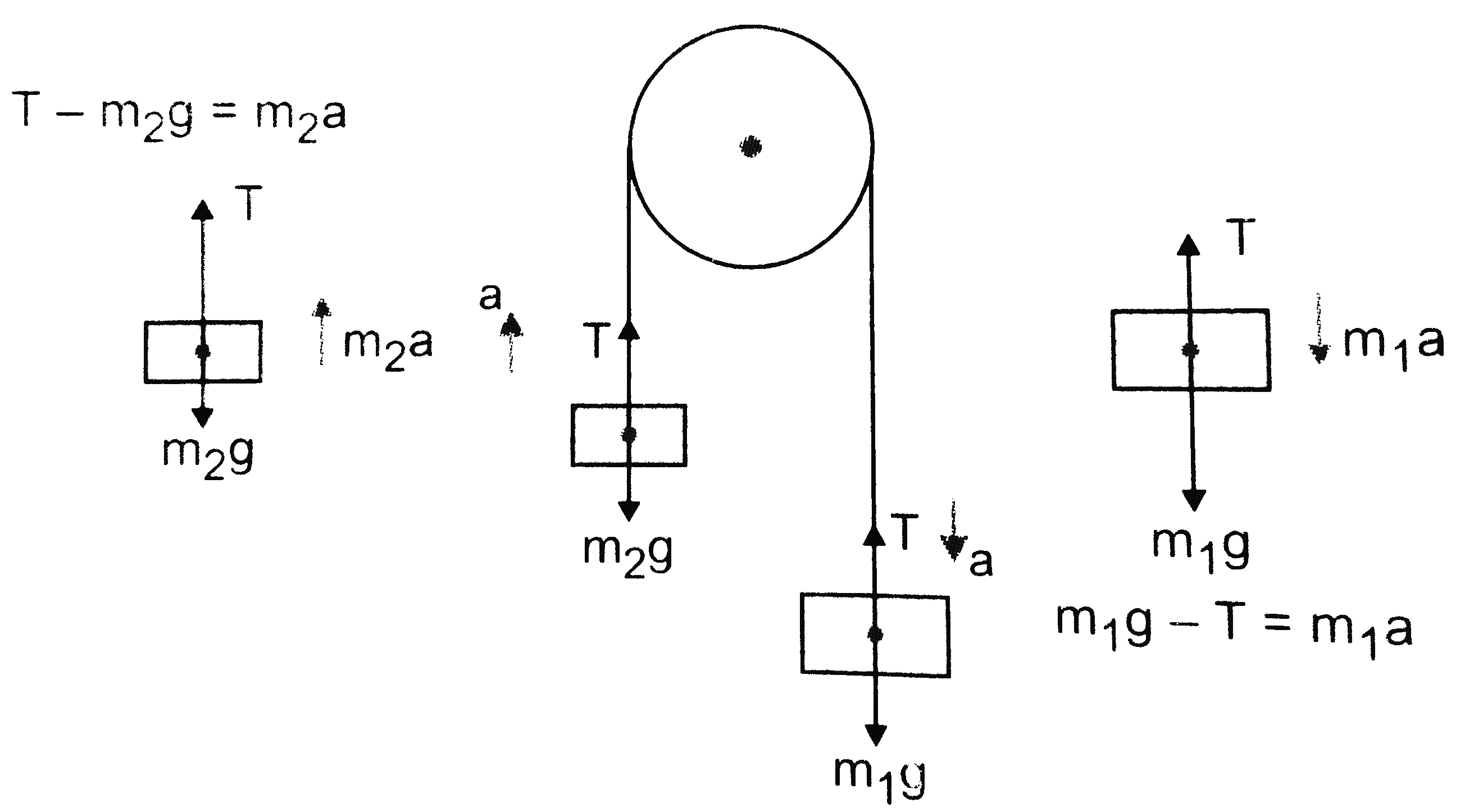
Next, we need to find the tension T in the string. We can analyze the forces acting on either mass. Let's consider the 4 kg mass.
Using Newton's second law for the 4 kg mass:
W2−T=m2⋅a
Substituting the known values:
40N−T=4kg⋅3.33m/s2
Calculating the right side:
40N−T=13.32N
Now, solving for T:
T=40N−13.32N=26.68N
Final Results
- The acceleration of the masses is approximately 3.33m/s2.
- The tension in the string is approximately 26.68N.
When the masses are released, the force acting on each mass due to gravity can be calculated as follows:
- For m1 (2 kg), the weight W1=m1⋅g=2⋅10=20N (acting upwards).
- For m2 (4 kg), the weight W2=m2⋅g=4⋅10=40N (acting downwards).
According to Newton's second law, the net force acting on the system is equal to the mass of the system multiplied by its acceleration.
Fnet=W2−W1=40N−20N=20N
mtotal=m1+m2=2kg+4kg=6kg
Fnet=mtotal⋅a
Substituting the values we have:
20N=6kg⋅a
Solving for a:
a=20N6kg=103≈3.33m/s2
Next, we need to find the tension T in the string. We can analyze the forces acting on either mass. Let's consider the 4 kg mass.
W2−T=m2⋅a
Substituting the known values:
40N−T=4kg⋅3.33m/s2
Calculating the right side:
40N−T=13.32N
Now, solving for T:
T=40N−13.32N=26.68N
- The acceleration of the masses is approximately 3.33m/s2.
- The tension in the string is approximately 26.68N.
Topper's Solved these Questions
- LAWS OF MOTIONBook:AAKASH INSTITUTEChapter:LAWS OF MOTIONExercise:EXERCISE
Explore 70 Videos
- LAWS OF MOTIONBook:AAKASH INSTITUTEChapter:LAWS OF MOTIONExercise:Assignment (SECTION-A)
Explore 65 Videos
- LAWS OF MOTIONBook:AAKASH INSTITUTEChapter:LAWS OF MOTIONExercise:ASSIGNMENT ( SECTION -J ) Aakash Challengers Questions
Explore 5 Videos
- KINETIC THEORYBook:AAKASH INSTITUTEChapter:KINETIC THEORYExercise:EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
Explore 10 Videos
- MAGNETISM AND MATTER Book:AAKASH INSTITUTEChapter:MAGNETISM AND MATTER Exercise:ASSIGNMENT (SECTION D)
Explore 26 Videos
Similar Questions
Two masses 2 kg and 4 kg are connected at two ends of light inextensible string passing over a frictionless pulley . If the masses are released , then find the accelaration of the masses and the tension in the string .
View SolutionTwo masses 7 kg and 12 kg are connected at the two ends of a light inetensible string that goes over a frictionless pulley. Find the acceleration of the masses and the tension in the string when the masses are released.
View Solution
Knowledge Check
Two masses M1=5kg,M2=10kg are connected at the ends of an inextensible string passing over a frictionless pulley as shown. When masses are released, then acceleration of masses will be
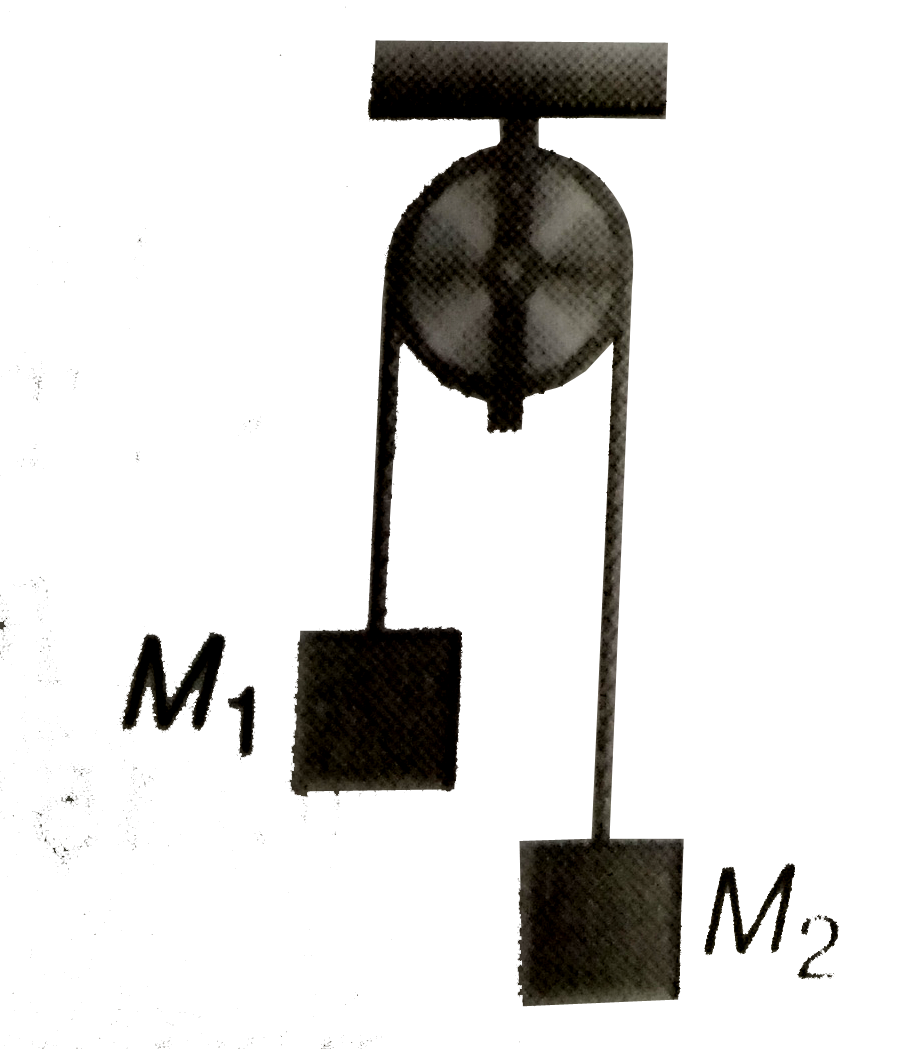 AgBg/2Cg/3Dg/4
AgBg/2Cg/3Dg/4Two masses M1=5kg,M2=10kg are connected at the ends of an inextensible string passing over a frictionless pulley as shown. When masses are released, then acceleration of masses will be
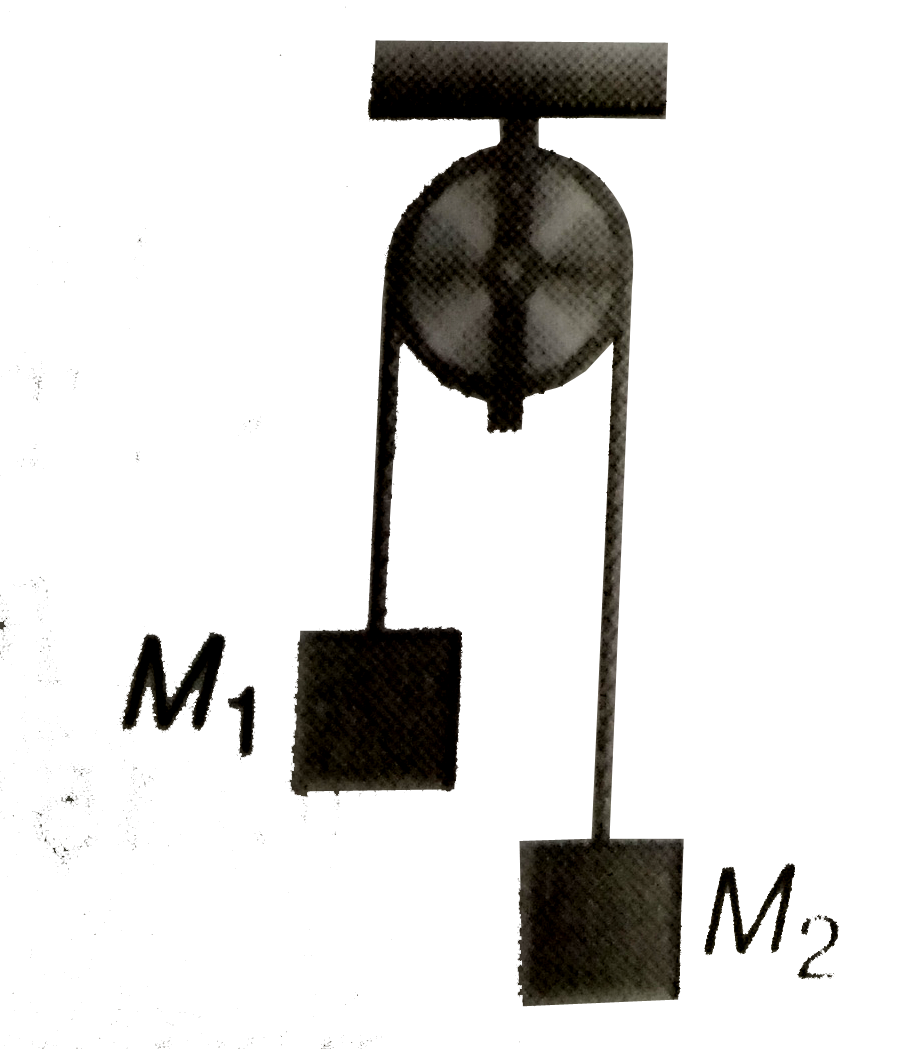 AgBg2Cg3Dg4
AgBg2Cg3Dg4Two masses of 10 kg and 6 kg connected at the two ends of an inextensible string pass over a smooth frictionless pulley . Calculate acceleration of the system and tension in the string .
A71.5 NB72.5 NC73.5 ND74.5 N
Two masses 8 kg and 12 kg are connected at the two ends of a light inextensible string that passes over a frictionless pulley Fibd the acceleration of the masses and tension in the string , when the masses are released
 .
.
View SolutionTwo masses M and m are connected at the two ends of an inextensible string. The string passes over a smooth frictionless pulley. Calculate the acceleration of the masses and the tension in the string. Given M>m.
View SolutionTwo bodies of masses 2kg and 3kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string (g=10ms−2)
View SolutionTwo blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.

View SolutionTwo blocks of masses 6kg and 4kg are attached to the two ends of a massless string passing over a smooth fixed pulley. if the system is released, the acceleration of the centre of mass of the system will be
View Solution