A particle is moving with constant speed v along x - axis in positive direction. Find the angular velocity of the particle about the point (0, b), when position of the particle is (a, 0).
To find the angular velocity of a particle moving with constant speed v along the x-axis about the point (0,b) when the position of the particle is (a,0), we can follow these steps:
Step 1: Identify the position of the particle and the reference point
The particle is located at (a,0) on the x-axis, and we need to calculate its angular velocity about the point (0,b).
Step 2: Determine the position vector R
The position vector R from the point (0,b) to the particle's position (a,0) can be expressed as:
R=(a−0,0−b)=(a,−b)
Step 3: Calculate the magnitude of R
The magnitude of the position vector R is given by:
R=√a2+b2
Step 4: Determine the velocity vector v
Since the particle is moving along the x-axis with a constant speed v, its velocity vector can be expressed as:
v=(v,0)
Step 5: Find the perpendicular component of the velocity v⊥
To find the angular velocity, we need the component of the velocity that is perpendicular to the position vector R. The angle θ between R and the x-axis can be found using the tangent function:
tan(θ)=−ba
Thus, we can find cos(θ):
cos(θ)=a√a2+b2
And the sine:
sin(θ)=−b√a2+b2
The component of the velocity that is perpendicular to R is given by:
v⊥=vsin(θ)=v(−b√a2+b2)=−bv√a2+b2
Step 6: Calculate the angular velocity ω
The angular velocity ω is given by the formula:
ω=v⊥R
Substituting the values we found:
ω=−bv√a2+b2√a2+b2=−bva2+b2
Final Result
Thus, the angular velocity of the particle about the point (0,b) when the position of the particle is (a,0) is:
ω=−bva2+b2
The particle is located at (a,0) on the x-axis, and we need to calculate its angular velocity about the point (0,b).
The position vector R from the point (0,b) to the particle's position (a,0) can be expressed as:
R=(a−0,0−b)=(a,−b)
The magnitude of the position vector R is given by:
R=√a2+b2
Since the particle is moving along the x-axis with a constant speed v, its velocity vector can be expressed as:
v=(v,0)
To find the angular velocity, we need the component of the velocity that is perpendicular to the position vector R. The angle θ between R and the x-axis can be found using the tangent function:
tan(θ)=−ba
Thus, we can find cos(θ):
cos(θ)=a√a2+b2
And the sine:
sin(θ)=−b√a2+b2
v⊥=vsin(θ)=v(−b√a2+b2)=−bv√a2+b2
The angular velocity ω is given by the formula:
ω=v⊥R
Substituting the values we found:
ω=−bv√a2+b2√a2+b2=−bva2+b2
Thus, the angular velocity of the particle about the point (0,b) when the position of the particle is (a,0) is:
ω=−bva2+b2
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTECHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - J) Aakash Challengers Questions13videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH INSTITUTECHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))5videos
SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITS
- BOOK - AAKASH INSTITUTECHAPTER - TEST 1EXERCISE - EXERCISE9videos
TEST 1
Similar Questions
A particle moves uniformly with speed v along a parabolic path y=kx2, where k is a positive constant. Find the acceleration of the particle at the point x = 0.
View SolutionParticles AandB move with constant and equal speeds in a circle as shown in (Fig. 5.164). Find the angular velocity of the particle A with respect to B, if the angular velocity of particle Aw.r.t.O is ω.
 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
A particle of mass m is moving with constant speed v on the line y=b in positive x-direction. Find its angular momentum about origin, when position coordinates of the particle are (a, b).
AmvbBmvb/2Cmvb/4Dnone - Question 2 - Select One
A particle is moving with constant speed v along the line y = a in positive x -direction. Find magnitude of its angular velocity about orgine when its position makes an angle θ with x-axis.
Avsin2θaBvcos2θaCvsinθDNone - Question 3 - Select One

A particle mass parallel to x-axis with constant velocity v as shown in the figure. The angular velocity of the particle about the origin OAremains constantBcontinuously increasesCcontinuously decreasesDoscillates.
A particle moving parallel to x-axis as shown in fig. such that at all instant the y-axis component of its position vector is constant and is equal to 'b'. Find the angular velocity of the particle about the origin when its radius vector makes angle θ from the axis.
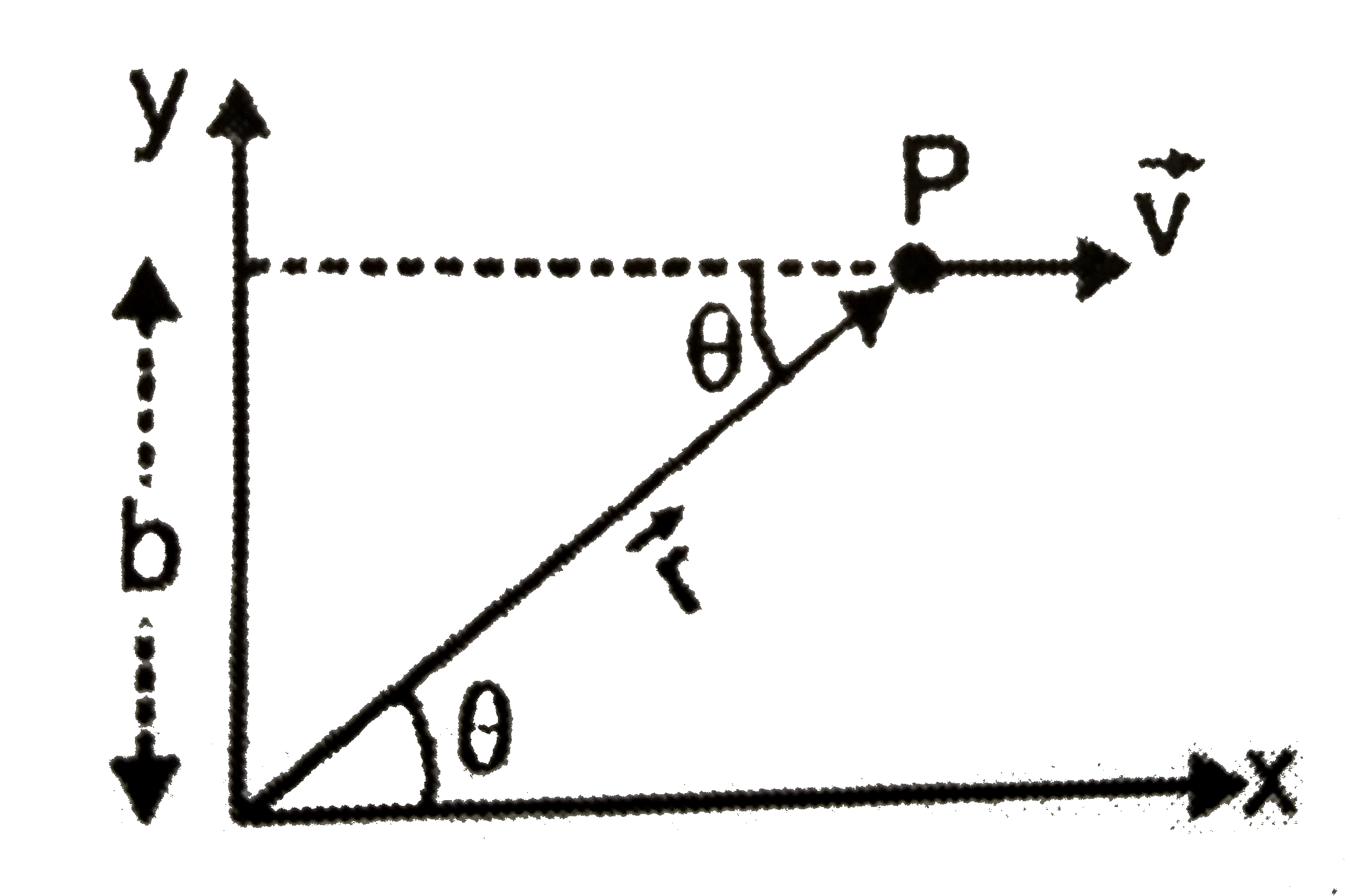 View Solution
View SolutionA particle is moving along x-direction with a constant acceleration a. The particle starts from x=x0 position with initial velocity u. We can define the position of the particle with time by the relation
x=x0+ut+12at2
plot the position of the particle in relation with time is following situations
(i) If initial position of the particle is on negativ x-axis, initial velocity is positive and acceleration is negative.
(ii) If initial position is positive, initial velocity is negative and acceleration is positive.View SolutionA particle is moving along a straight line parallel to x-axis with constant velocity. Find angular momentum about the origin in vector form :
 View Solution
View SolutionA particle is moving with constant speed v in xy plane as shown in figure. The magnitude of its angular velocity about point O is
 View Solution
View SolutionA particle moves in a circle with constant angular velocity ω about a point P on its circumference. The angular velocity of the particle about the centre C of the circle is
View Solution

