In the question number 36, at which distance a weight may be hung along the rod, in order to produce equal strains in both the wires?
43m from steel wire
43m from brass wire
1 m from steel wire
14m from brass wire
The correct Answer is:C
As Strain =SrtressYoung's Modulus ltbr ∴ Strain in stell wire =TSASYS
For equal strain in both the wire,
TSASYS=TBABYB
∴TSTB=ASABYSYB=0.1cm20.2cm2×2×1011Nm−21×1011Nm−2=1.......(i)
For the rotational equlibrium of the rod,
TSx=TB(2−x)
2−xx=TSTB=1 [Using (i)]
2−x=xorx=1m
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTIPSCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Applications Of Elastic Behaviour Of Materials
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTIPSCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Higher Order Thinking Skills
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTIPSCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Stress-Strain Curve
5 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTIPSCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Assertion And Reason
15 Videos
- MOTION IN A PLANE BOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason
15 Videos
Similar Questions
Alight rod of length 2 m is suspended horizontlly from the ceiling bty means of two vertical wirs of equal length tied to its ends One wire is mode of steel and is of cross - section 0.1 sq cm and the other is of brass of cross -section 0.2 sq cm Find the postion along the rod at which a wight may be hung to produce (i) equal stress in both wirs (ii) equal strain in both wires (Y for brass =10×1010Nm−2andYfor steel=20×1010Nm−2)
View SolutionA light rod AC of length 2.00 m is suspended from the ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 10−3m2 and the other is of brass of cross-section 2×10−3m2. The position of point D from end A along the rod at which a weight may be hung to produce equal stress in both the wires is [Young's modulus of steel is 2×1011Nm2 and for brass is 1×1011Nm−2]
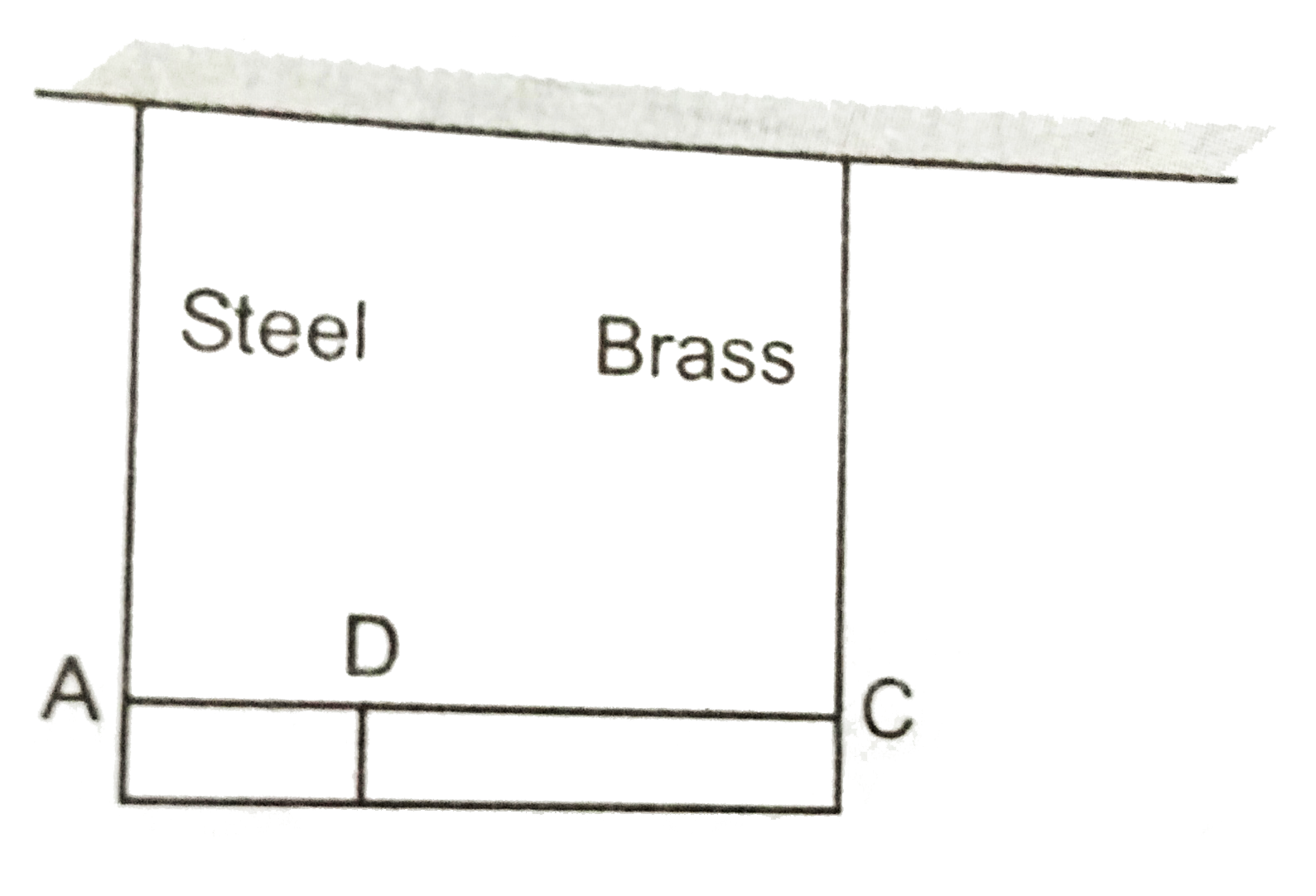
View Solution
Knowledge Check
A light rod of length 200 cm is suspended from the ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross- section 0.1 cm2 and the other of brass of cross-section 0.2 cm2 . Along the rod at which distance a weight may be hung to produce equal stresses in both the wires ?
A43 m from steel wireB43 m from brass wireC1 m, from steel wireD14 m from brass wireA light rod of length 2 m is suspended from ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 0.1 cm sq. and the other of brass of cross-section 0.2 cm sq. Along the rod at what distance a weight may be hung to produce equal stresses in the wires?
(YSteel=2×1011Nm−2,YBass=1×1011Nm−2)A43m from steel wireB43m from brass wireC1 m from steel wireD14m from brass wireA light rod AC of length 2.00 m is suspended from the ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 10−3m2 and the other is of brass of cross-section 2×10−3m2. The position of point D from end A along the rod at which a weight may be hung to produce equal stress in both the wires is [Young's modulus of steel is 2×1011Nm2 and for brass is 1×1011Nm−2]
 A1.00mB(2/3)mC(4/3)mD(5/3)m
A1.00mB(2/3)mC(4/3)mD(5/3)m
A light rod of length 4.0 m is suspended from the ceiling horizontally by means of two vertical wire of equal length tied to its ends. One of the wires is made of steel and is of cross-section 10−3m2 and the other is of brass of cross-section 2×10−3m2. Find out the position along the rod at which a weight may be hung to produce equal stresses in both wires.
View SolutionA light rod of length 4.0 m is suspended from the ceiling horizontally by means of two vertical wire of equal length tied to its ends. One of the wires is made of steel and is of cross-section 10−3m2 and the other is of brass of cross-section 2×10−3m2. Find out the position along the rod at which a weight may be hung to produce equal stresses in both wires.
View SolutionA light rod of length 200cm is suspended from the ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 0.1cm2 and the other of brass of cross-section 0.2cm2. A distance long the rod at which a weight may be hung to produce equal stresses in both the wires?
View SolutionIn the question number 46, if the mass M is hung the free end of the wire, then the extension produc in the wire is
View SolutionIn the question number 46, if the mass M is hung the free end of the wire, then the extension produc in the wire is
View Solution
