The radii and Young's moduli of two uniform wires A and B are in the ratio 2:1 and 1:2 respectively. Both wires are subjected to the same longitudinal force. If the increase in length of the wire A is one percent, the percentage increase in length of the wire B is
1.0
1.5
2.0
3.0
The correct Answer is:C
Young's modulus, Y=FLπr2ΔL
or ΔLL=Fπr2Y
For the same force,
∴ΔLBLBΔLALA=(rArB)2(YAYB)=(21)2(12)=2
ΔLBLB=2(ΔLALA)
ΔLBLB×100=2(ΔLALA×100)=2%
Topper's Solved these Questions
- BOOK - NCERT FINGERTIPSCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Applications Of Elastic Behaviour Of Materials8videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTIPSCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Higher Order Thinking Skills8videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTIPSCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Stress-Strain Curve5videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTIPSCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Assertion And Reason15videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - NCERT FINGERTIPSCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason15videos
MOTION IN A PLANE
Similar Questions
A wire has Piosson's ratio 0.3. If the change in the length of the wire on stretching it is 1% then percentage change in the volume of the wire will be
View SolutionA cylindrical wire is stretched to increase its length by 10%. The percentage increase in the resistance of the wire will be–
View Solution
Knowledge Check
- Question 1 - Select One
If the force constant of a wire is K, the work done in increasing the length of the wire by l is
AK/2BKlCKl2/2DKl2 - Question 2 - Select One
A wire is suspended by one end. At the other end a weight equivalent to 20 N force is applied. If the increase in length is 1.0 mm, the increase in energy of the wire will be
A0.01 JB0.02 JC0.04 JD1.00 J - Question 3 - Select One
The ratio of the lengths of two wires A and B of same material is 1:2 and the ratio of their diameters is 2:1. They are stretched by the same force, then the ratio of increase in length will be
A2:1B1:4C1:8D8:1
A stress of 1kg/mm2 is applied on a wire. If the modulus of elasticity of the wire is 1010dyne/cm2, then the percentage increase in the length of the wire will be
View SolutionIf the ratio of lengths, radii and young's modulii of steel and brass wires in the figure are a,b and c, respectively. Then, the corresponding ratio of increase in their lengths would be
 View Solution
View SolutionTwo parallel wires A and B of same meterial are fixed to rigid support at the upper ends and subjected to same load at the lower ends. The subjeted to same load at the lower ends. The lengths of the wire are in the ration 4 : 5 and their radii are in the ratio 4 : 3 the increase in the length of wire A is 1 mm. Calculate the increase in the length of wire A is 1mm. Calculate the increase in the length of the wire B.
View SolutionThe ratio of the lengths of two wires A and B of same material is 1:2 and the ratio of their diameter is 2:1. They are stretched by the same force, then the ratio of increase in length will be
View SolutionIf the ratio of diameters,lengths and Young's moduli of steel and brass wires shown in the figure are p, q and r respectively . Then the corresponding ratio of increase in their lenghts would be
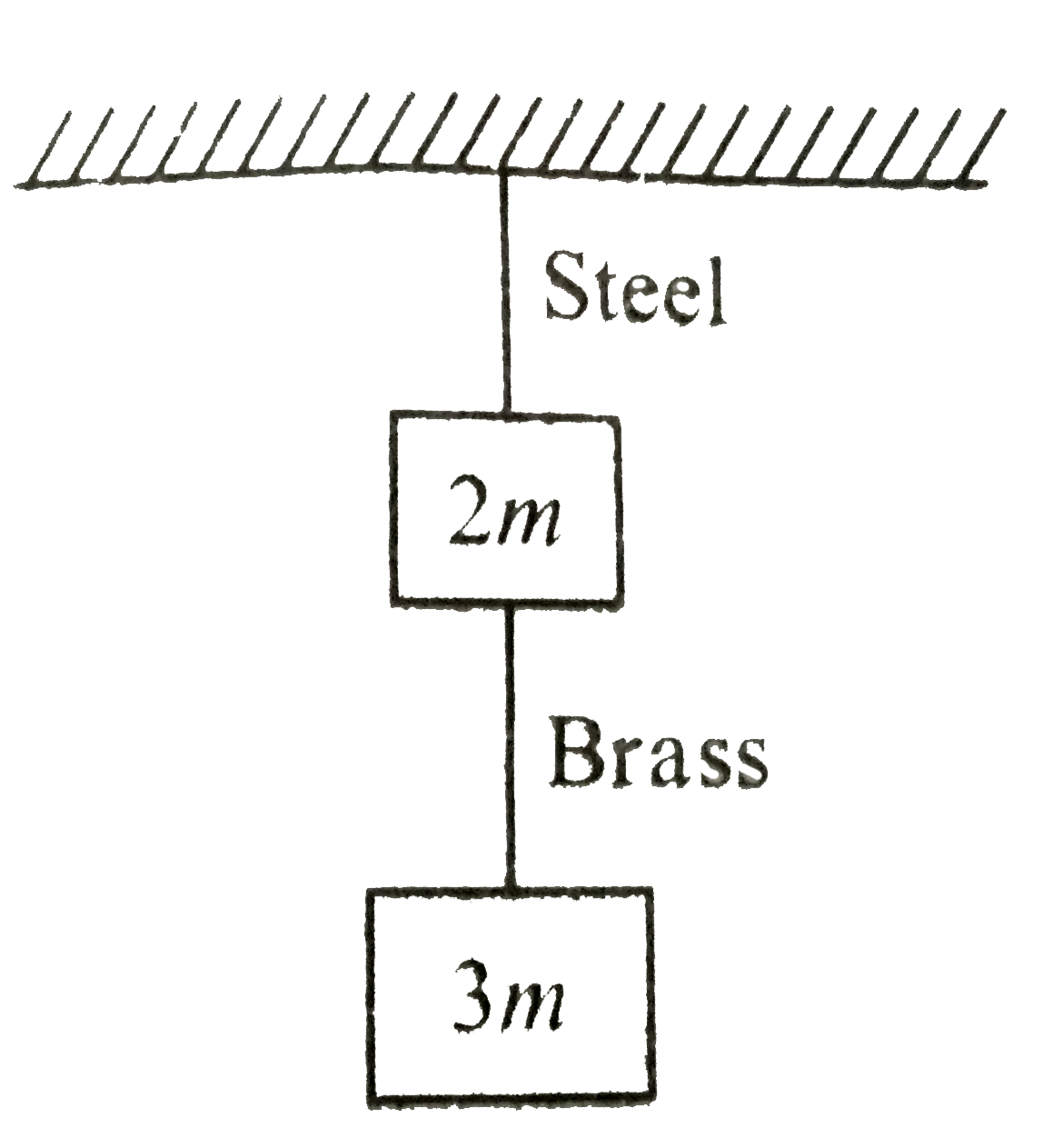 View Solution
View Solution

