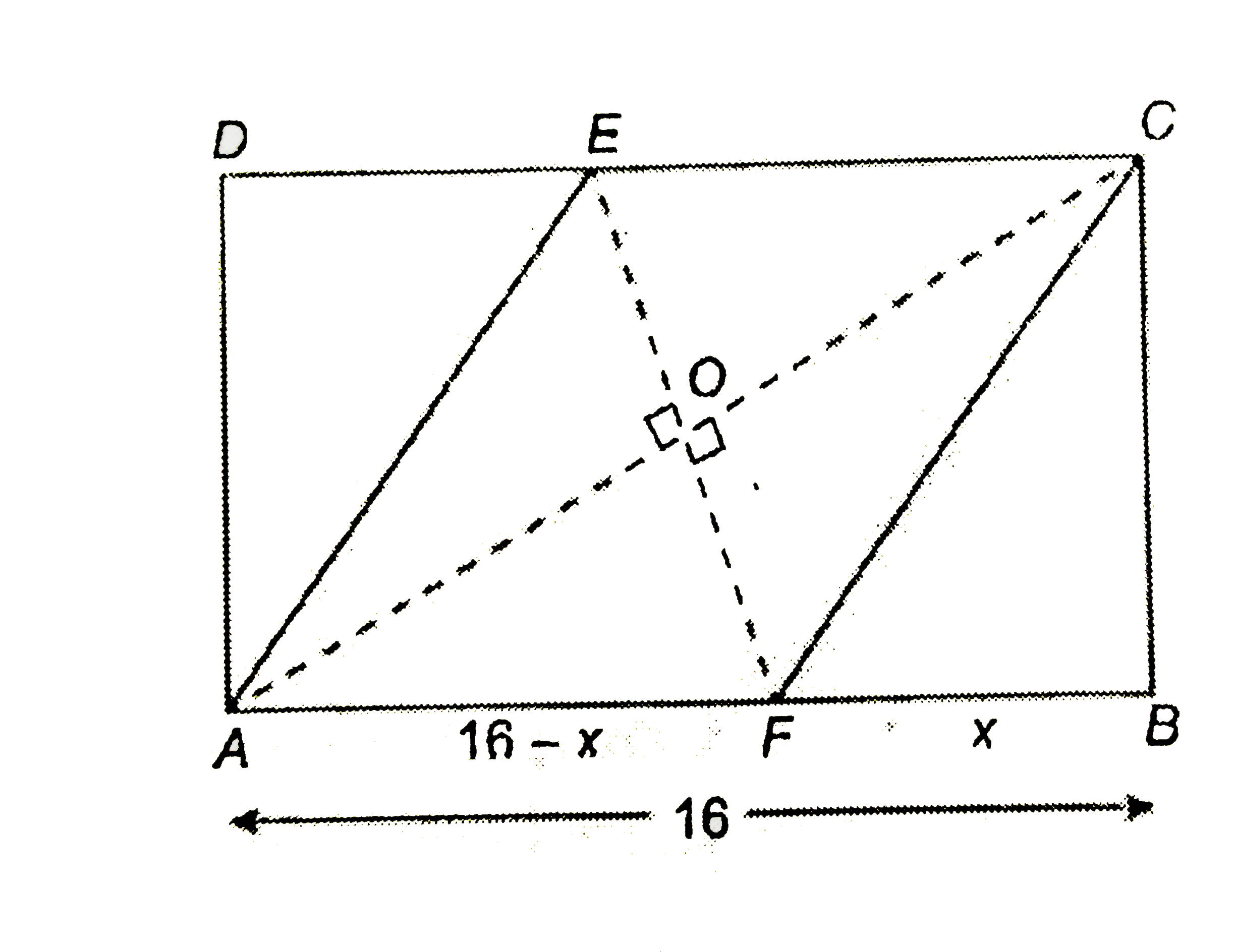
$A B C D$ is a rectangle with $A B = 16$ units and $B C = 12$ units. $F$ is a point on $A B$ and $E$ is a point on $C D$ such that $A F C E$ is a rhombus. Find the length of $E F .$

A

$15 units$

B

$16 units$

C

$17 units$

D

$18 units$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Let $F B$ $x$ units
$∴ A F = 16 - x$ units
Since AFCE is a rhombus.
$∴ F C = A F = 16 - x$
Now, by Pythagoras theorem,
$C F^{2} = F B^{2} + B C^{2}$
$\Rightarrow {(16 - x)}^{2} = x^{2} + 144$
$\Rightarrow 256 + x^{2} - 32 x = x^{2} + 144$
$\Rightarrow 32 x = 112$
$\Rightarrow x = \frac{112}{32} = \frac{7}{2}$ units
$∴$ Side of rhombus $= 16 - x = 16 - \frac{7}{2} = \frac{25}{2}$ units
By Phythagoras theorem,
$a C^{2} = A B^{2} + B C^{2}$
$\Rightarrow A C^{2} = {(16)}^{2} + {(12)}^{2} = 256 + 144 = 400 \Rightarrow A C = 20$
$∴ A O = \frac{1}{2} \times A C = 10$ units
$∴ A E^{2} = O E^{2} + A O^{2}$ (by Phythagoras theorem)
${(\frac{25}{2})}^{2} = O E^{2} + {(10)}^{2}$
$\Rightarrow O E^{2} = 156.25 - 100 = 56.25 \Rightarrow O E = 7.5$
$∴ E F = 2 \times O E = 2 \times 7.5 = 15$ units

|

Updated on:21/07/2023

Class 9 MATHS HERONS FORMULA

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Diagonal $A C$ of a rectangle $A B C D$ is produced to the point $E$ such that $A C : C E = 2 : 1, A B = 8 c m$ and $B C = 6 c m$ . Find the length of $D E$ .
A $2 \sqrt{19}$ $c m$
B $15$ $c m$
C $3 \sqrt{17}$ $c m$
D $13$ $c m$
Question 2 - Select One
ABCD is a rectangle where ratio of the length of AB and BC is 3 : 2. If P is the mid point of AB . Find sin $∠ C P B$
A $\frac{3}{5}$
B $\frac{2}{5}$
C $\frac{3}{4}$
D $\frac{4}{5}$
Question 3 - Select One
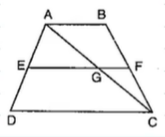
Let ABCD be a trapezium in which AB||DC and E be the mid-point of AD. If F be a point on BC such that EF||AB. Then EF, where F is the mid-point of BC, is equal to

AAB + DC
B $\frac{1}{2} (A B + D C)$
C $\frac{1}{3} (A B + D C)$
DNone of these

NAGEEN PRAKASHAN-HERONS'S FORMULA-Revision Exercise

Home
Ask Doubt
Profile