Assume that two radioactive substances A and B disintegrate as:
−d[A]dt=kA,t1/2(A)=0.693KA
−d[B]dt=kB[B]2,t1/2(B)=1kB[B]0
If both the half life periods and initial concentrations are equal, what will be the ratio of the rates of the two substances at the start of the reaction?
According to available data, t1/2(A)=t1/2(B)
0.693kA=1kB[B]0 or kAkB=0.693[B]0=0.693[A]0
At the start of the reaction
rA=kA[A]0, rB=kB[B]20=kB[A]20 ……......[∴[A]0=[B]0]
∴rArB=kA[A]0kB[A]20 or rArB=0.693[A]0×[A]0[A]02=0.693
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - DINESH PUBLICATIONCHAPTER - CHEMICAL KINETICSEXERCISE - NCERT Exercise
1 Videos
- CHEMICAL KINETICSBOOK - DINESH PUBLICATIONCHAPTER - CHEMICAL KINETICSEXERCISE - NCERT in text questions
38 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTUREBOOK - DINESH PUBLICATIONCHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTUREEXERCISE - Unit Test - 1
20 Videos
- CHEMICAL THERMODYNAMICS AND CHEMICAL ENERGETICS BOOK - DINESH PUBLICATIONCHAPTER - CHEMICAL THERMODYNAMICS AND CHEMICAL ENERGETICS EXERCISE - Exercise
507 Videos
Similar Questions
The rate law equation for the reaction A→B is found to be −d[A]dt=k[A]1/2
If [A]0 were the initial concentration of A, derive expressions for
(i) rate constant in the integrated form (ii) half-life period of the reaction
View SolutionThe rate of change of concentration of (A) for reaction A→B is given by
−d[A]dt=k[A]1/3
Derive expression for half-life period of the reaction.
View Solution
Knowledge Check
For the reaction 2A→B+3C, if −d[A]dt=k1[A]2,−d[B]dt=k2[A]2,−d[C]dt=k3[A]2 the correct reaction between k1,k2 and k3 is :
Ak1=k2=k3B2k1=k2=3k2C4k1=k2=3k2Dk12=k2=k33For a reaction, A+2B→C, rate is given by +d[C]dt=k[A][B], hence, the order of the reaction is
A1B2C1D03A→2B, rate of reaction +d[B]dt is equal to:
A−32d[A]dtB−23d[A]dtC−13d[A]dtD+2d[A]dt
Activities of three radioactive substances A, B and C are represented by the curves A, B and C, in the figure. Then their half- lives T12(A):T12(B):T12(C) are in the ratio :
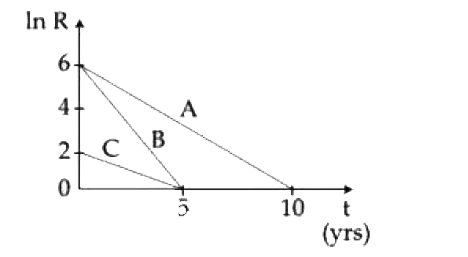
View SolutionIn the reaction 3A→2B, rate of reaction +d[B]dt is equal to :
View SolutionFor the reaction AK1⇐===⇒K2B, if 'a' is the initial concentration of A and n is the number of moles of A reacting if initially no B was present then :
View SolutionThe rate and mechanism of chemical reactions are studied in chemical kinetics. The elementary reactions are single step reactions having no mechanism. The order of reaction and molecularity are same for elementary reactions. The rate of forward reaction aA+bB→cC+dD is given as :
rate = =(dxdt)=−1ad[A]dt=−1bd[B]dt=1cd[C]dt=1dd[D]dt or rate =K[A]a[B]b. In case of reversible reactions net rate expression can be written as : rate =K1[A]a[B]b–K2[C]c[D]d. At equilibrium, rate = 0. The constants K,K1,K2, are rate constants of respective reaction. In case of reactions governed by two or more steps reactions mechanism, the rate is given by the solwest step of mechanism.
For the reaction : aA→bB,
log[−dAdt]=log[dBdt]+0.6, then a:b is :
View SolutionFor the reaction A B, the rate law expression is −d[A]dt=k[A]1/2. If initial concentration of [A] is [A]0, then
View Solution
