A block of mass (10α), where α is a constant is moving with velocity 3 m/s to the right collides inelastically with the block on the right with mass 10g and sticks to it. The right block is connected to three springs as shown in the figure. The spring constant of each spring is 2N/m. If the amplitude of the resulting simple harmonic motion is 12√2m, then the value of α is
 Solution in Telugu
Solution in Telugu
5
2.5
7
10
The correct Answer is:A
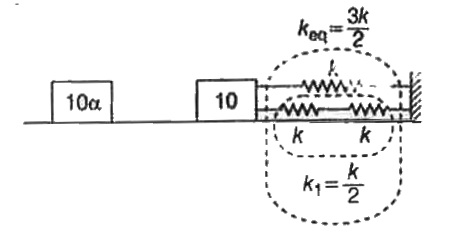
Equivalent spring constant of system of springs =3k2=3 N/m
Momentum is conserved in collision. Let blocks moves with velocity v just after collision, then
m1v1=(m1+m2)v
10α×10−3×3=(10α+10)×10−3×v
⇒v=30α10(α+1)=3αα+1ms−1
Displacement of blocks is 12√2m
So, energy conservation gives
12(m1+m2)v2=12keqx2 [friction is absent]
⇒(10α+10)×10−3×9α2(α+1)2=3×18
⇒9α2100(α+1)=38
24α2=100α+100⇒α=5
Topper's Solved these Questions
Similar Questions
If A represents density, B represents velocity, C represents specific heat capacity and D represents wavelength, then the quantity having the dimensions of product of A, B, C and D is
View SolutionMatch the entries in List I with those in List II.

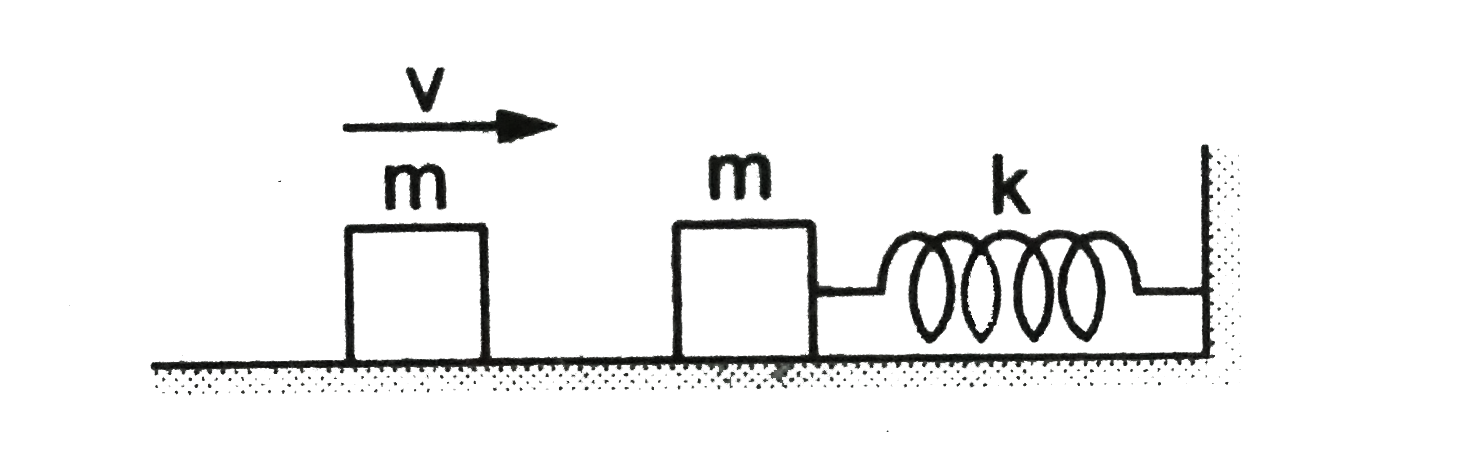
View SolutionThe left block in filgure collides inelastically with the right block and sticks to it. Find the amplitude of the resulting simple harmonic motion.
View SolutionA block of mass m is connected to another block of mass M by a spring (massless) of spring constant k. The block are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is unstretched. Then a constant force F starts acting on the block of mass M to pull it. Find the force of the block of mass M.
View SolutionA block of mass 'm' collides perfectly inelastically with another identical block connected to a spring of of force constant 'K'. The amplitude of resulting SHM will be
View SolutionA block of mass m is connected to another .block of mass M by a massless spring of spring constant k. A constant force f starts action as shown in figure, then:
View SolutionA block of mass 'm' is connected to another block of mass 'M' by a spring (massless) of spring constant 'k' The blocks are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is unstretched Then a constant force 'F' starts acting on the block of mass 'M' to pull it. Find the force on the block of mass 'm' :
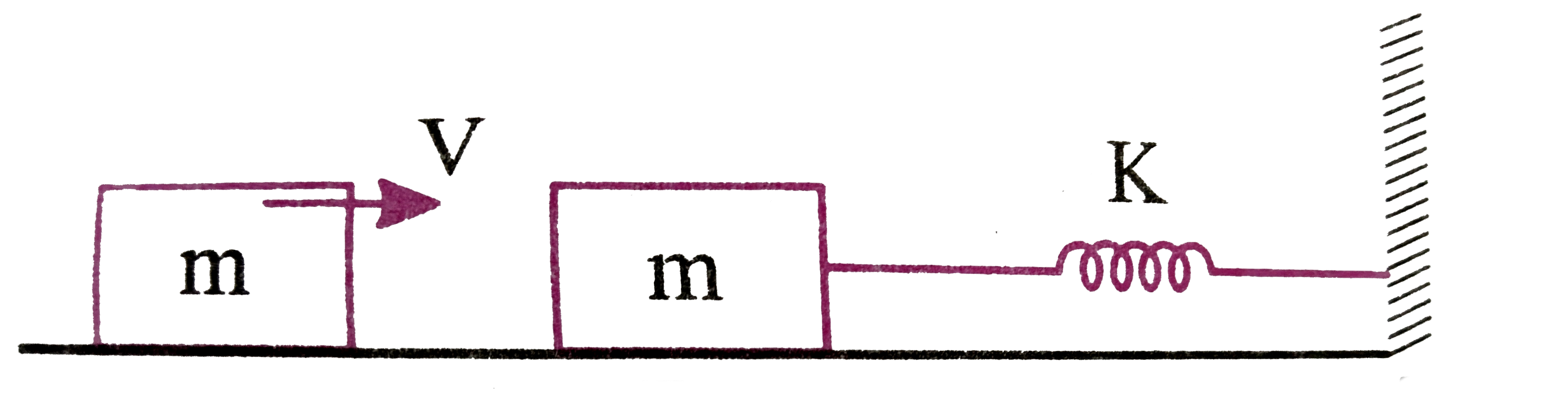
View SolutionThe left block in figure collides inelastically with the right block and striks to it. Find the amplitude of the resulting simple harmonic motion
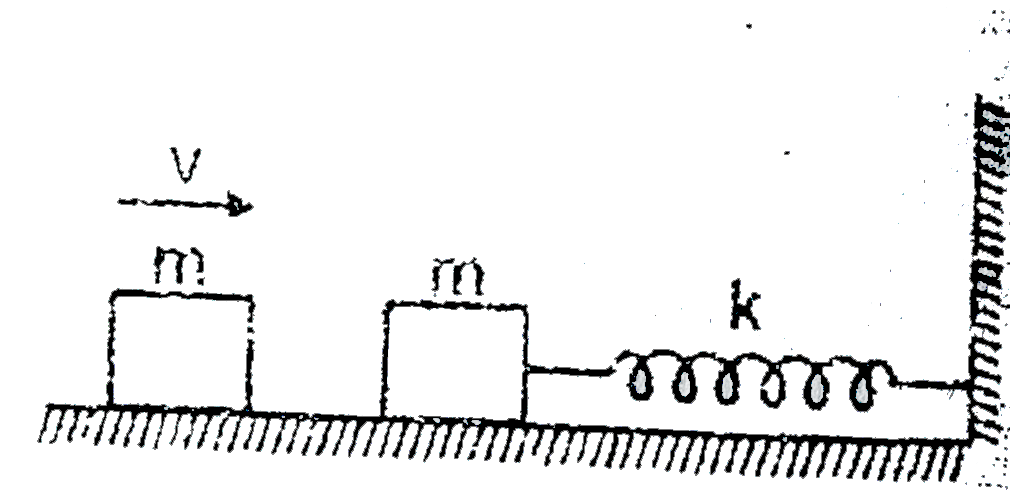
View SolutionA block of mass m is connected to another block of mass M by a massless spring of spring constant K. Blocks are kept on a smooth horizontal plane. Initally both the blocks the at rest and spring is unstressed. Force F is applied on M to right as shownin the figure then which of the following is correct ?

View SolutionTwo blocks 1 and 2 of masses m and 2m respectively are connected by a spring of force constant k. The masses are moving to the right with uniform velocity v each, the heavier mass, leading the lighter one. The spring is of natural length in the motion. Block 2 collides head on with a third block 3 of mass m, at rest, the collision being completely inelastic. Determine the velocity of blocks at the instant of maximum compression of the spring.
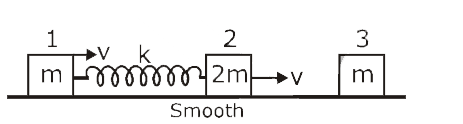
View SolutionThe left block in figure collides inelastically with the right block and sticks to it. Find the amplitude of the resulting simple harmonic motion.
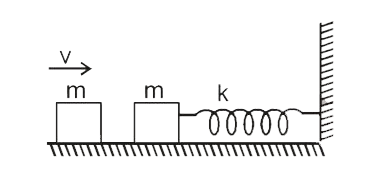
View SolutionA block of mass 'm' is connected to another block of mass 'M' by a spring (massless) of spring constant 'k'. The blocks are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is unstretched. Then a constant force 'F' starts acting on the block of mass 'M' to pull it. Find the force on the block of mass 'm'.
View SolutionA block of mass m is connected to another block of mass M by a spring (massless) of spring constant k. The blocks are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is unstretched. Then a constant force F starts acting on the block of mass M to pull it. Find the force on the block of mass m.
View SolutionTwo springs are connected to a block of mass M is placed on a frictionless surface as shown in figure. If both springs have a spring constant k, the frequency of oscillation of the block is………

View SolutionA block of m mass is moving at a velocity v which is completely unpredictable when connected to the spring of the K force constant by another all-time constant block. What will be the amplitude of the resulting simple periodic motion?
View Solution
