The diagonals of a parallelogram are 2ˆi and 2ˆj. What is the area of the parallelogram
Video Solution
Text Solution
LetA=2ˆiandB=2ˆj
Area=12|A×B|=12[2ˆi×2ˆj]
=12[4(ˆi׈j)]=12∣∣4ˆk∣∣(∴ˆi׈j=ˆk)
=2squnits.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
Diagonals of a parallelogram PQRS must be a
ArectangleBsquareCcyclic quadrilateralDrhombusIf 3ˆi+ˆj−2ˆkandˆi−3ˆj+4ˆk are the diagonals of a parallelogram, then the area of the parallelogram is
A10√3sq.unitsB5√3sq.unitsC5√2sq.unitsD10√2sq.unitsIf the diagonals of a parallelogram are 3ˆi+ˆj−2ˆkandˆi−3ˆj+4ˆk, then the lengths of its sides are
A√8,√10B√6,√14C√5,√12Dnone of these
The given figure shows a parallelogram of 20 m height. The base of the parallelogram is 40 m long.
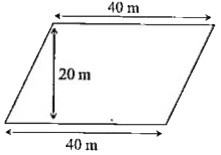
What is the area of the parallelogram?
View SolutionIf the diagonals of a parallelogram are 3i+j−2k and i−3j+4k then its area is.
View SolutionThe sides of a parallelogram are 2ˆi+4ˆ−5ˆkandˆi+2ˆj+3ˆk. The unit vector parallel to one of the diagonal is (A) 1√69(ˆi+2ˆj−8ˆk) (B) 1√69(−ˆi+2ˆj+8ˆk) (C) 1√69(−ˆi−2ˆj−8ˆk) (D) 1√69(ˆi+2ˆj+8ˆk)
View SolutionOne of diagonal of a parallelogram is 10 cm and an angle of the parallelogram is π/4. If its height be 8 cm then area of the parallelogram :
View SolutionOne of diagonal of a parallelogram is 10 cm and an angle of the parallelogram is π/4. If its height be 8 cm then area of the parallelogram :
View Solution