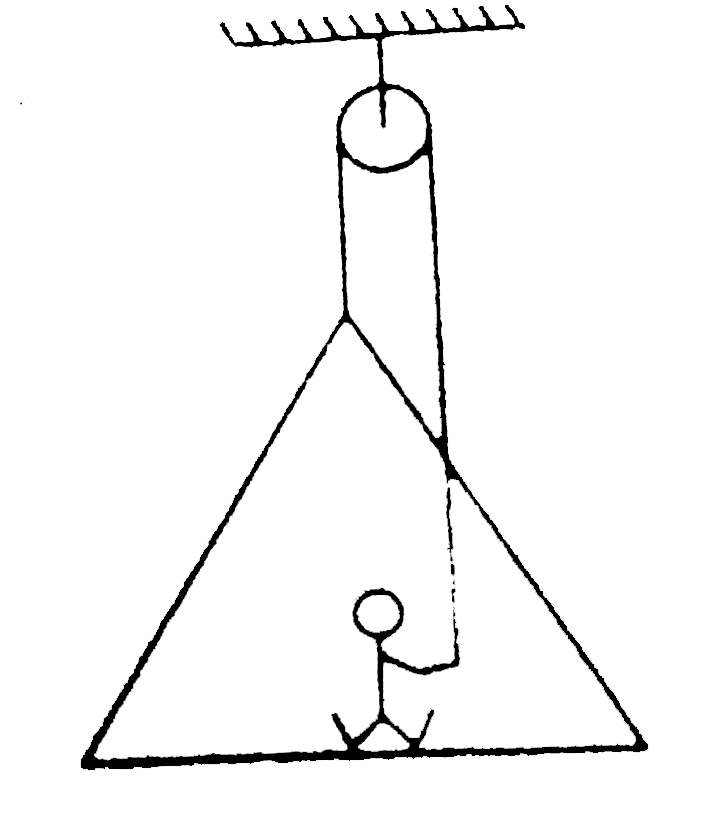
A mam of mass 50 g stands on a frame of mass 30 g. He pulls on a light rope which passes over a pulley. The other end of the rope is attached to the frame. For the system to be in equilibrium what force man must exert on the rope?
40 g
80 g
30 g
50 g
The correct Answer is:A
(a) Force exerted by man on rope transfers to it in the form of tension.
Net upwards force on the system is 2T or 2F.
Net downward force is (50+30)g=80g.
For equilibrium of system, 2F=80g or F=40g
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.320videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.420videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.120videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
- BOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective18videos
LAWS OF THERMODYNAMICS
Similar Questions
A man with mass 85 kg stands on a platform with mass 25 kg. He pulls on the free end of a rope that runs over a pulley on the ceiling and has its other end fastened to the platform. The mass of the rope and the mass of the pulley can be neglected, and the pulley is frictionless. With what force does he have to pull so that he and the platform have an upward acceleration of 2.2ms2. (Take g=10ms2)
View SolutionA 40 kg boy climbs a rope that passes over an ideal pulley. The other end of the rope is attached to a 60 kg weight placed on the ground. What is the maximum upward acceleration the boy can have without lifting the weight ? If he climbs the rope with upward acceleration 2 g, with what acceleration the weight will rise up ?
View Solution
Knowledge Check
- Question 1 - Select One
A man of mass m stands on a frame of mass M. He pulls on a light rope, which passes over a pulley. The other end of the rope is attached to the frame. For the system to be in equilibrium, what force must the man exert on the rope?
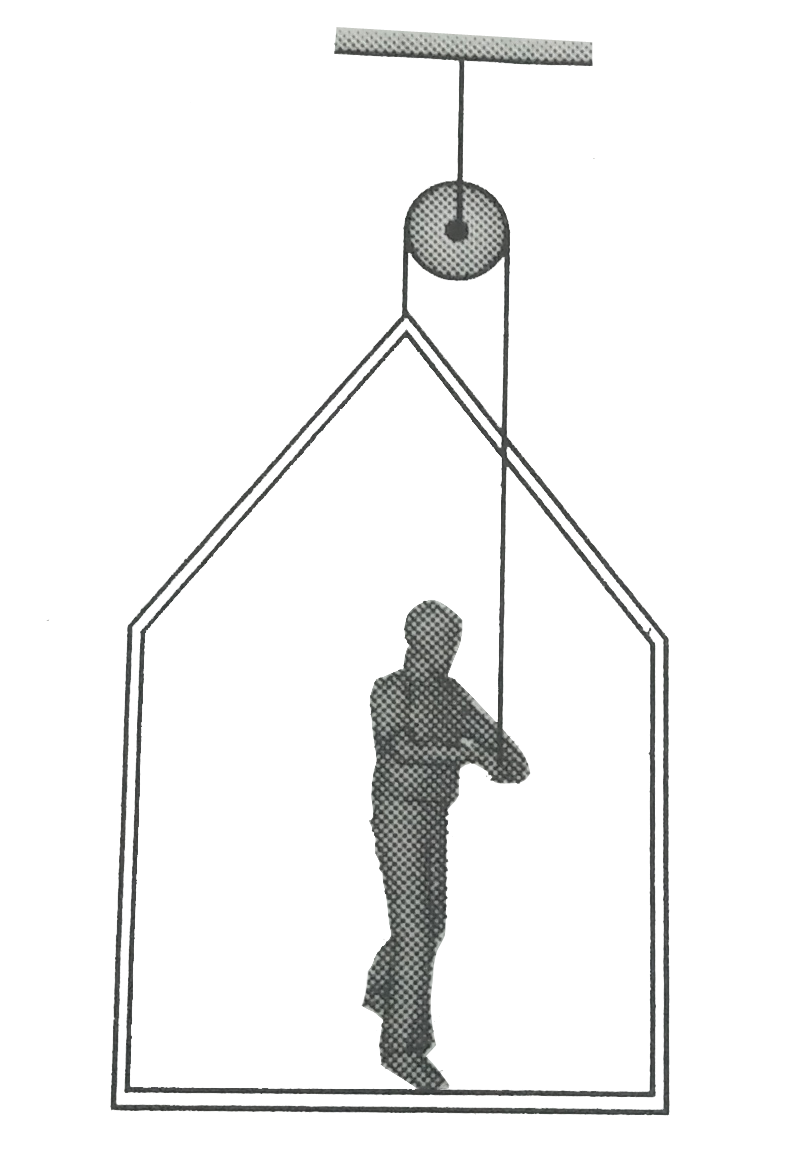 A(M+m)g2B(M+m)gC(M−m)gD(M+2m)g
A(M+m)g2B(M+m)gC(M−m)gD(M+2m)g - Question 2 - Select One
As shown in figure a monkey of 20g mass is holding a light rope that passes over a frictionless pulley. A bunch of bananas of the same mass is tied to the other end of rope. Inorder to get access to the bunch the monkey starts climbing the rope. The distance between the monkey and the bananas is
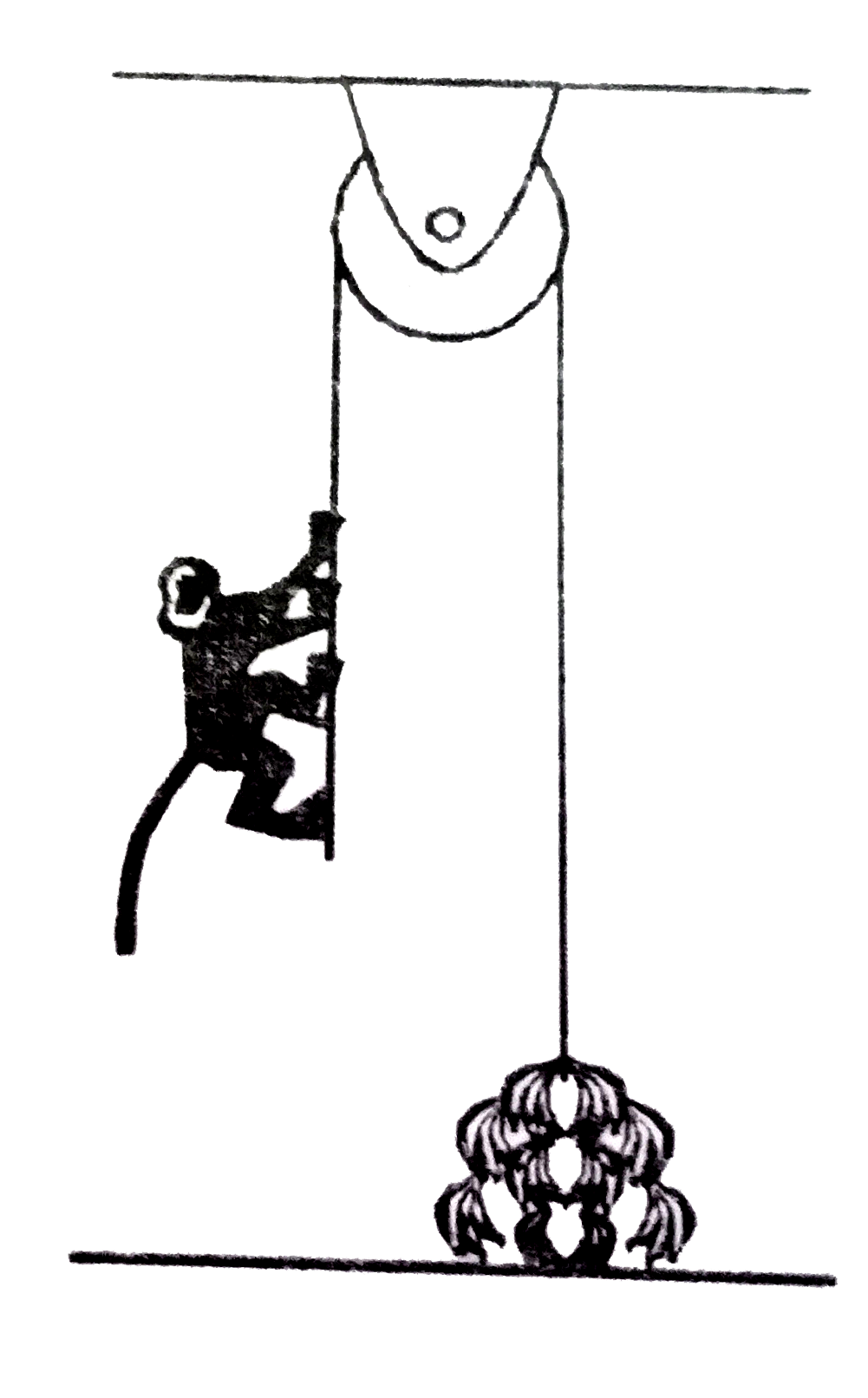 ADecreasingBIncreasingCUnchangedDNothing can be stated
ADecreasingBIncreasingCUnchangedDNothing can be stated - Question 3 - Select One
A painter of mass M stands on a platform of mass m and pulls himself up by two ropes which hang over pulley as shown in fig. He pulls each rope with force F and moves upward with a uniform acceleration a. find a, neglecting the fact that no one could do this for long time.
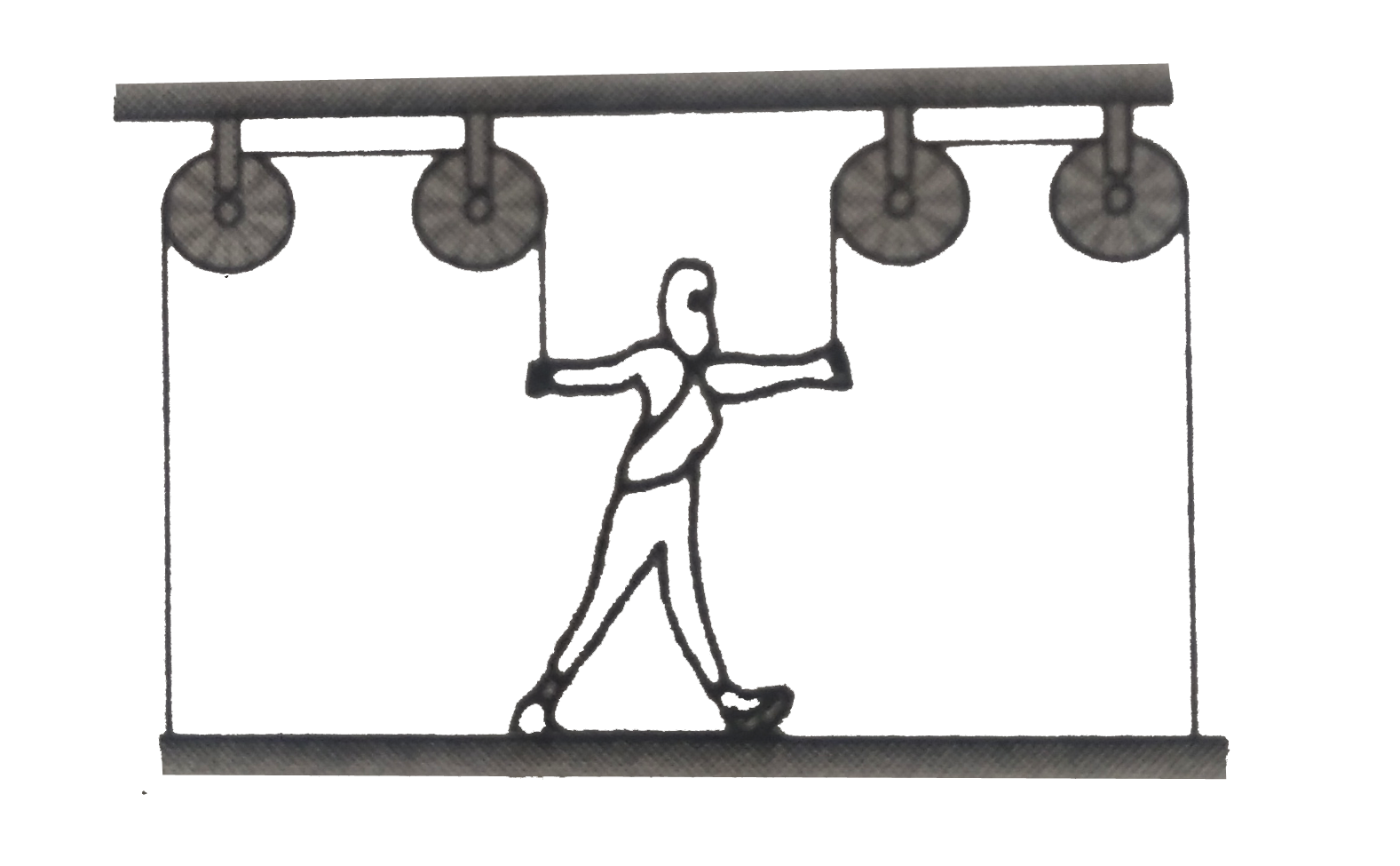 A4F+(2M+m)gM+2mB4F+(M+m)gM+2mC4F−(M+m)gM+mD4F−(M+m)g2M+m
A4F+(2M+m)gM+2mB4F+(M+m)gM+2mC4F−(M+m)gM+mD4F−(M+m)g2M+m
Block A of mass m/2 is connected to one end of light rope which passes over a pulley as shown in fig. A man of mass m climbs the other end of rope with a relative acceleration of g/6 with respect to rope. Find the acceleration of block A and tension in the rope.
 View Solution
View SolutionA 50kg person stand on a 25kg platform. He pulls on the rope which is connected to the rope which is attected to the plateform via the frictionless pulley as shown in the figure. The platform moves upwards at a steady rate if the force with which the person pulls the rope is
 View Solution
View SolutionA cart of mass M is tied to one end of a massless rope of length 10m. The other end of the rope is in the hands of a man of mass M. The entire system is on a smooth horizontal surface. The man is at x=0 and the cart at x=10m. If the man pulls the cart by the rope, the man and the cart will meet at the point
View SolutionTwo men of unequal masses hold on to the two sections of a light rope passing over a smooth light pulley. Which of the following are possible ?
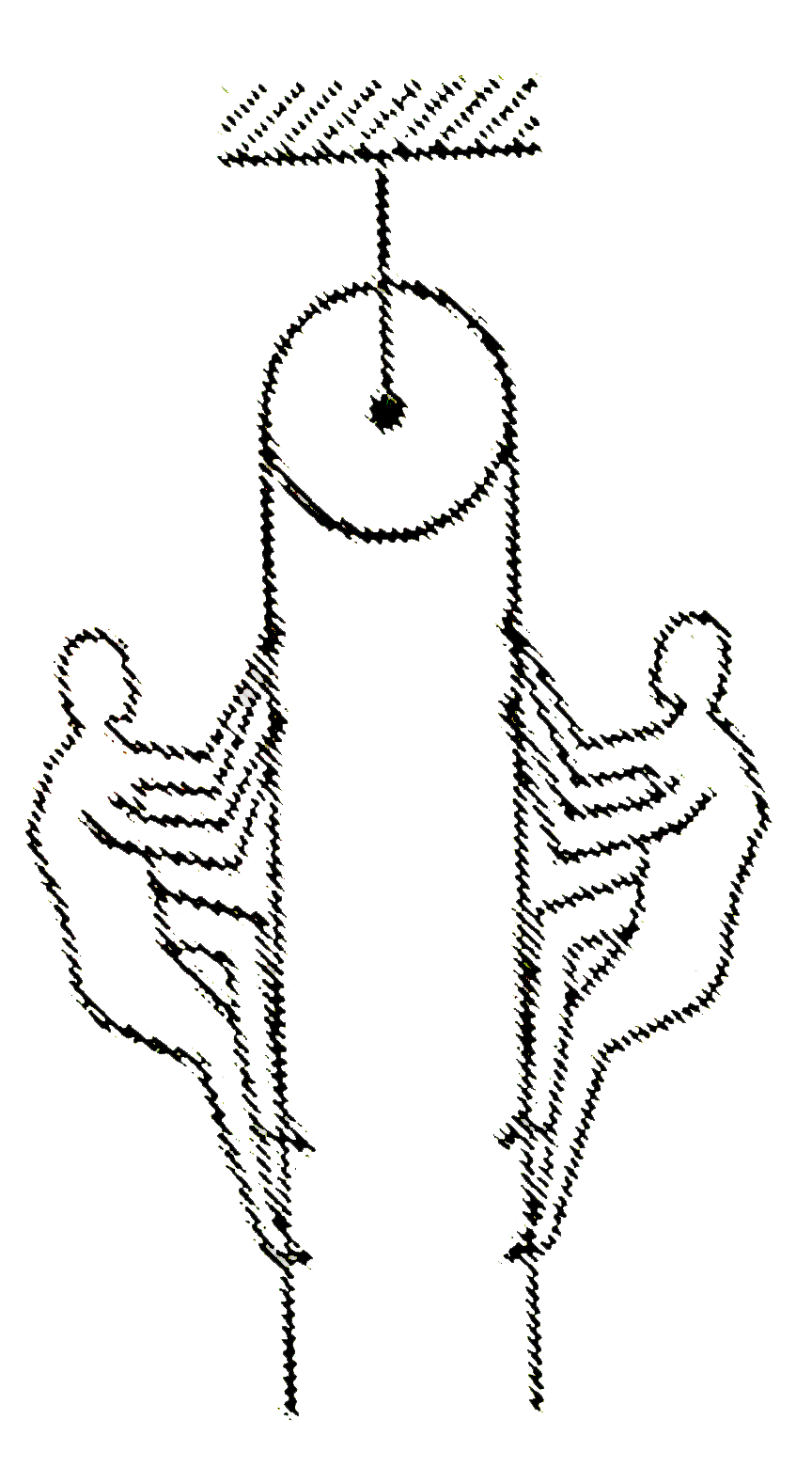 View Solution
View SolutionA cat of mass m = 1 kg climbs to a rope hung over a leight frictionless pulley. The opposite end of the rope is tied to a weight of mass M = 2m lying on a smooth horizontal plane. What is the tension of the rope where the cat move upwards with in acceleration a=2m/s2 relative to the rope ?
 View Solution
View Solution

