A smooth inclined plane of length L, having an inclination θ with horizontal is inside a lift which is moving down with retardation a. The time taken by a block to slide down the inclined plane from rest will be
√2L√asinθ
√2Lgsinθ
√2L(g−a)sinθ
√2L(g+a)sinθ
The correct Answer is:D
(d) Moving down with retardation a means, lift is accelerated upwards. With respect to lift, pseudo force on the block will be ma in downward direction, where m is the mass of block. So, downward force mg on the block will be replaced by m(g+a). Therefore, acceleration of block relative to plane will be,
ar=(g+a)sinθ(down the plane)
From , L=12art2 (s=12at2)
t=√2Lar=√2L(g+a)sinθ
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Assertion and reason
28 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Match the columns
7 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.4
20 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
A frictionless inclined plane of length l having inclination θ is placed inside a lift which is accelerating downward with on acceleration a (<g). If a block is allowed to move down the inclined plane, from rest, then the time taken by the block to slide from top of the inclined plane to the bottom of the inclined plane is
View SolutionA block can slide on a smooth inclined plane of inclination θ kept on the floor of a lift. When the lift is descending with a retardation a , the acceleration of the block relative to the incline is
View Solution
Knowledge Check
A smooth inclined plane of length L having inclination θ with the horizontal inside a lift which is moving down with retardation α . The time taken by a body to slide down the inclined plane, from rest, will be
A√2LgsinθB√2LαsinθC√2L(g+α)sinθD√2L(g−α)sinθA block is released from rest on a smooth inclined plane having inclination 37∘ with the horizontal. The inclined plane is fixed on a lift which is moving up with retardation 5ms−2. Find out the displacement of the block with respect to the lift in initial 1 second (Take g=10ms−2)
 A1.5mB1.25mC2.25mD2.5m
A1.5mB1.25mC2.25mD2.5mA smooth inclined plane is inclined at an angle θ with the horizontal. A body starts from rest and slides down the inclined surface. The time taken by the body to reach the bottom is
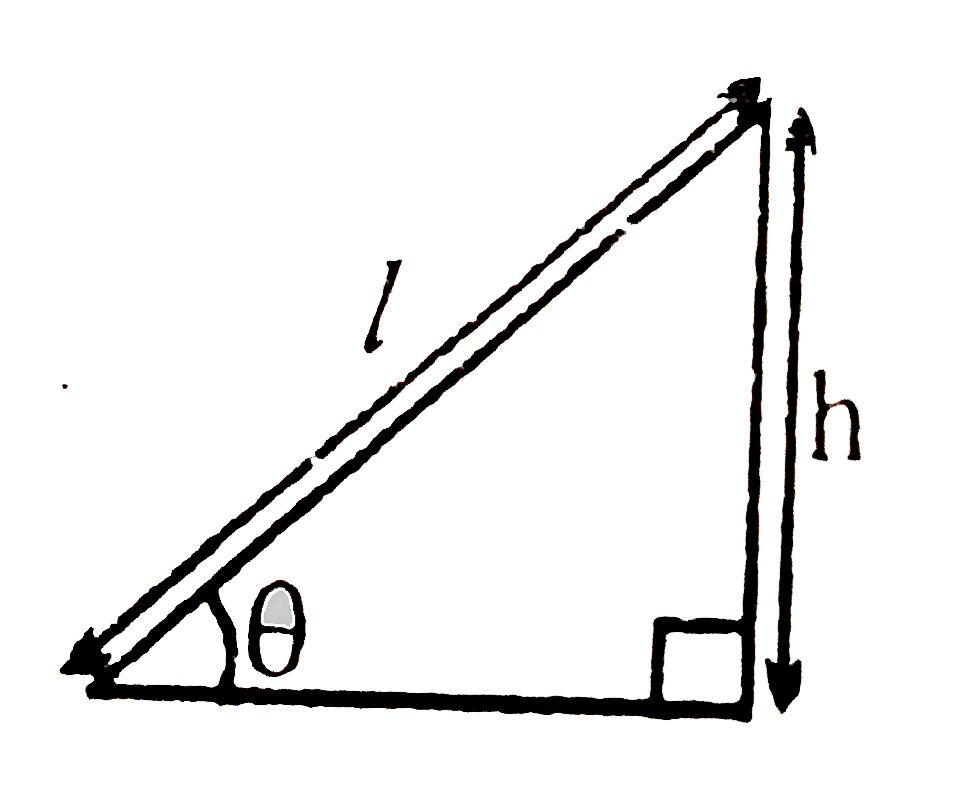 A√2hgB√2lgC√2lgsinθD1sinθ√h2g
A√2hgB√2lgC√2lgsinθD1sinθ√h2g
A block can slide on a smooth inclined plane of inclination θ kept on the floor of a lift. When the lift is descending with a retardation a , the acceleration of the block relative to the incline is
View SolutionA block can slide on a smooth inclined plane of inclination θ kept on the floor of a lift. When the lift is descending with a retardation a , the acceleration of the block relative to the incline is
View SolutionA block can slide on a smooth inclined plane of inclination θ kept on the floor of a lift. When the lift is descending with a retardation a , the acceleration of the block relative to the incline is
View SolutionA body of mass m is placed over a smooth inclined plane of inclination θ, which is placed over a lift which is moving up with an acceleration a0 . Base length of the inclined plane is L. Calculate the velocity of the block with respect to lift at the bottom, if it is allowed to slide down from the top of the plane from rest :-
View SolutionA block mass 'm' is placed on a frictionless inclined plane of inclination θ with horizontal. The inclined plane is accelerated horizontally so that the block does not slide down. In this situation vertical force exerted by the inclined plane on the block is:
View Solution
