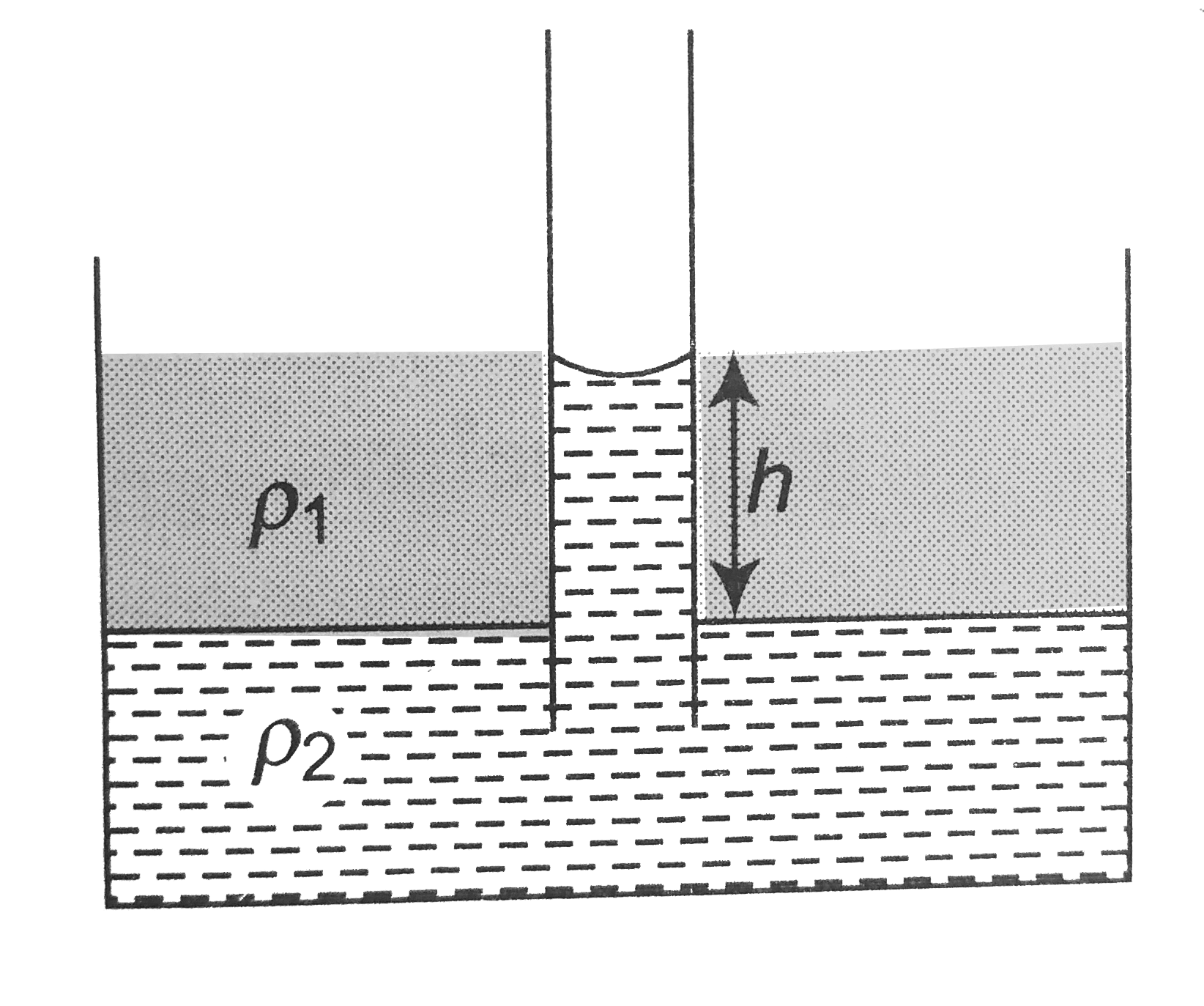
A container is partially filled with a liquid of density ρ2 A capillary tube of radius r is vertically inserted in this liquid. Now another liquid of density ρ1(ρ1<ρ2) is slowly poured in the container to a height h as shown. There is only denser liquid in the capillary tube. The rise of denser liquid in the capillary tube is also h. Assuming zero contact angle, the surface tension of heavier liquid is
A
2πrρ2gh
B
ρ2rgh2
C
r2(ρ2−ρ1)gh
D
2πr(ρ2−ρ1)gh
Video Solution
Text Solution
The correct Answer is:C
Let us write pressure equation in path ABCDE.
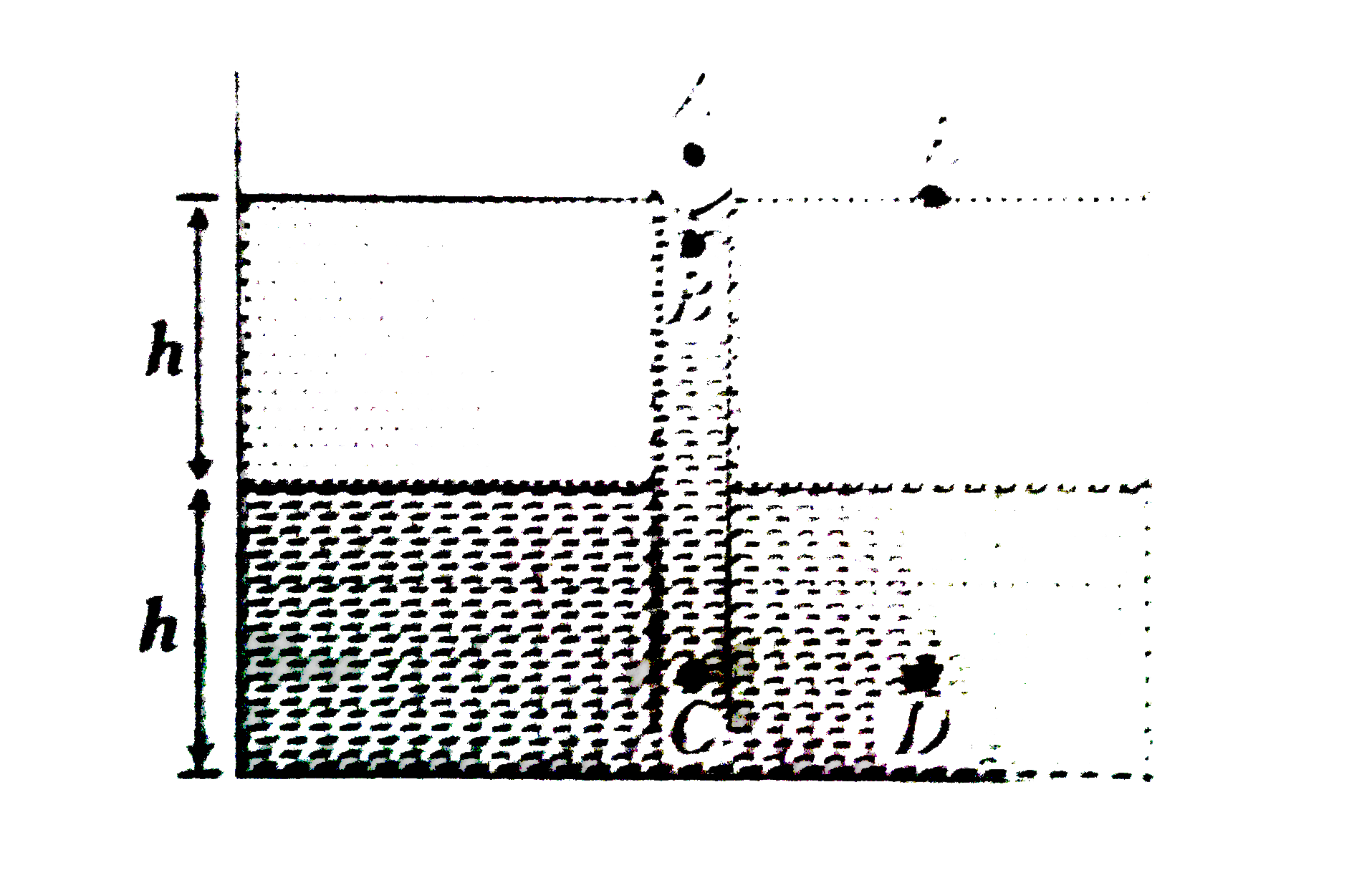
p0−2Tr+ρ2g(h+h')−ρ2gh'−ρ1gh=p0
∴T=r2(ρ2−ρ1)gh
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - B) Medical entrance special format question19videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Match the columns6videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Check point 13.410videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
Knowledge Check
- Question 1 - Select One
The rise of a liquid in a capillary tube is due to :
AosmosisBsurface tension (c) viscosityCviscosityDdiffusion - Question 2 - Select One
The rise of a liquid in a capillary tube is due to :
AviscosityBosmosisCdiffusionDsurface tension - Question 3 - Select One or More
The rise of liquid in a capillary tube depends on :
Athe material of tube and nature of liquidBthe length of tubeCthe outer radiusDthe inner radius of the tube
The rise of a liquid in a capillary tube depends on
View SolutionThe liquid in the capillary tube will rise if the angle of contact is
View SolutionWhen a liquid rises inside a capillary tube , the weight of the liquid in the tube is supported
View SolutionWhen a liquid rises inside a capillary tube , the weight of the liquid in the tube is supported
View SolutionIn gravity free space. The liquid in a capillary tube will rise to
View Solution

