In a double slit pattern , the first order and tenth order maxima fall at 12.50 mm and 14.55mm from a particular reference point. If is changed to 5500Å, find the position of zero order and tenth order fringes, other arrangements remaining the same.
As know that shift due to insertion of a slab is
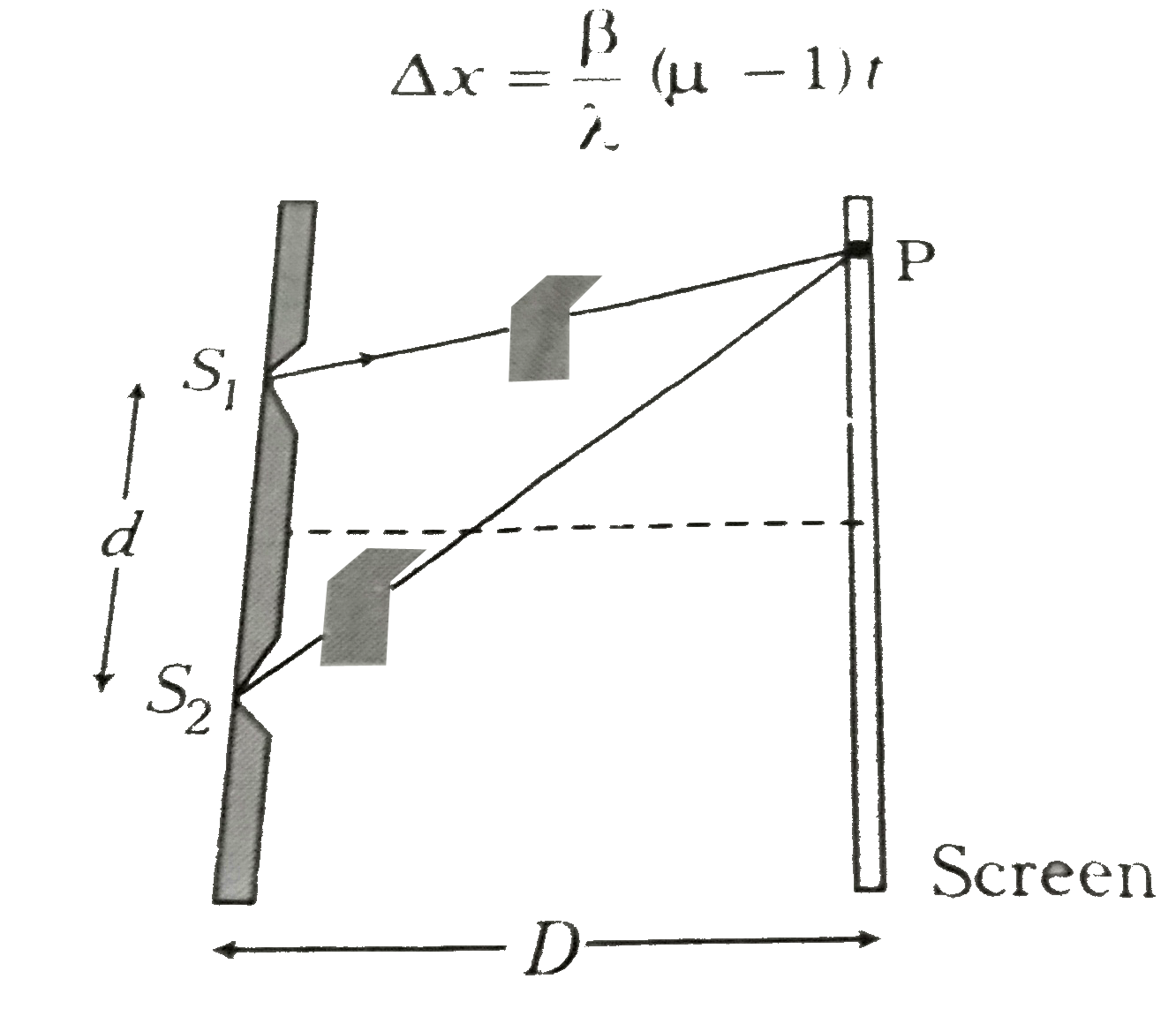
Shift due to one plate
Shift due to another path
Net shift
here, it is given that,
Hence,
Topper's Solved these Questions
Similar Questions
In a double-slit pattern (λ=6000Å), the zero-order and tenth-order maxima fall at 12.34 mm and 1473mm from a particular reference point. If λ is changed to 5000Å, find the position of the zero order and tenth order fringes, other arrangements remaining the same.
View SolutionIn a double slit experiment, the distance between the slits is 5.0 mm and the slits are 1.0m from the screen. Two interference patterns can be seen on the screen one due to light with wavelength 480nm, and the other due to light with wavelength 600nm. What is the separation on the screen between the third order bright fringes of the two intergerence patterns?
View Solution
Knowledge Check
In a double slit pattern (λ=6000Å), the first order and tenth order maxima fall at 12.50 mm and 14.55mm from a particular reference point. If λ is changed to 5500Å, find the position of zero order and tenth order fringes, other arrangements remaining the same.
A1.25 mm and 2.55 mmB1.25 mm and 2.65 mmC2.50 mm and 3.10 mmD0.50 mm and 1.89 mmIn an interference pattern the position of zeroth order maxima is 4.8 mm from a certain point P on the screen. The fringe width is 0.2 mm. The position of second maxima from point P is
A5.1 mmB5 mmC40 mmD5.2 mmIn an interference pattern produced by using a biprism, the zeroth order maxima is at a distance of 4.7mm from a point P on the screen. If the fringe width is 0.2 mm, then the distance of the second minima from the point P will be
Aonly 4.4 mmBonly 5 mmCeither 4.4 mm or 5 mm depending upon the position of PD6 mm
In a double slit experiment, the distance between the slits is 3 mm and the slits are 2 m away from the screen. Two interference patterns can be seen on the screen one due to light with wavelength 480 nm, and the other due to light with wavelength 600 nm. What is the separation on the screen between the fifth order bright fringes of the two interference patterns?
View SolutionIn Young's double slit experiment, the slits are 0.5mm apart and interference pattern is observed on a screen placed at a distance of 1.0m from the plane containg the slits. If wavelength of the incident light is 6000Å, then the separation between the third bright fringe and the central maxima is
View SolutionA parallel beam of light of all wavelength greater than 3000Å falls on a double slit in a Young's double slit experiment. It is observed that the wavelength 3600Å and 6000Å are absent at a distance of 31.5 mm from the position of the centre maximum and the orders of the interference at this point for the two wavelength differ by 7. If the distance between the slit and the screen in 1m, the sepration between the two slits is
View SolutionA young's double slit apporatus is immersed in a liquid of refractive index 1.33.It has slit separation of 1 mm and interference pattern is observed on the screen at a distance 1.33 m from plane of slits.The wavelength in air is 6300Å
Find the distance of seventh bright fringe from third bright fringe lying on the same side of central bright fringe.
View SolutionA young's double slit apparatus is immerged in a liquid of refractive index 43. It has slit separation of 1mm and interference pattern is observed on the screen at a distance 43m from plane of slits. The wavelength in air is 6300 Å
Find the distance of seventh bright fringe from third bright fringe lying on the same side of central bright fringe
View Solution
