A long wire having a semi-circular loop of radius r carries a current I, as shown in Fig. Find the magnetic field due to entire wire.

μ0I4r
μ0I24r
μ0I4r2
None of these
The correct Answer is:A
According to Biot savart Law, the magnetic induction at a point to a current carrying elemnet i δ l is given by
δB=μ04π<al×rr3orB=μ04π<alsinθr2
Directed normal to plane containing δl and
r theta being angle between δl and r
r θ being angle between δ l and r
Magnetic field due to linear portions of wire
If we consider anuy element of lenth δl of either linear portion ab or de, the angle between δ l and r is 0 or π therefore.
sinθ=0
Hence, magnetic induction due to both linear portins of wire is
μ04π<alsinθr=0
Field due to semi circular are
Now angle between a current element δ l of semi circular arc and the radius vector of the elment to point c is π/2
Therefoe the magnitude of magnetic induction B at O due to this element is
δB=μ04π<alsinπ/2r2=μ0<al4πr2
Hence magnetic indution due to whole semi circular loop is B=Σμ04π<alr2=μ04πr2=μ04πii2Σδi=μ0i4πR2(πr)=μ0i4r
Topper's Solved these Questions
- SOLVED PAPER 2017BOOK - DC PANDEYCHAPTER - SOLVED PAPER 2017EXERCISE - Solved papers 2017(JIPMER)
32 Videos
- SOLVED PAPER 2017BOOK - DC PANDEYCHAPTER - SOLVED PAPER 2017EXERCISE - Solved papers 2017(JIPMER)
32 Videos
- SEMICONDUCTORSBOOK - DC PANDEYCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
- SOLVED PAPERS 2018BOOK - DC PANDEYCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER
22 Videos
Similar Questions
A wire loop formed by joining two semicircular wires of radii R1 and R2 carries a current I as shown in figure. Find the magnetic field induction at the centre O.

View SolutionA conducting circular loop of radius a is connected to two long, straight wires . The straight wires carry a current I as shown in figure . Find the magnetic field B at the centre of the loop.

View Solution
Knowledge Check
A part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is
 Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1)
Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1)The wire loop PQRS formed by joining two semi circular wires of radii R1, and _(2)Ry carries a current I as shown the figure. The inagnitude of magnetic induction at the centre O is
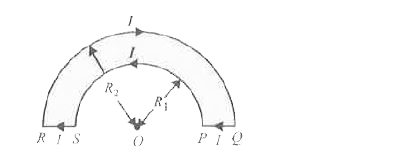 Aμ0I4(1R2−1R1)Bμ0I4(1R1−1R2)Cμ0I(1R2−1R1)Dμ0I(1R1−1R2)
Aμ0I4(1R2−1R1)Bμ0I4(1R1−1R2)Cμ0I(1R2−1R1)Dμ0I(1R1−1R2)Two very long, straight wires carrying, currents as shown in Fig. Find all locations where the net magnetic field is zero.
 Ay=√2xBy=xCy=−xDy=−(x2)
Ay=√2xBy=xCy=−xDy=−(x2)
A straight wire carrying a current of 5 A is bent into a semicircular arc of radius 2 cm as shown in the fig. Find the magnitude and direction of the magnetic field at the centre of the arc.

View SolutionA straight wire carrying a current of 12 A is bent into a semi-circular are of radius of2.0 cm as shown in the adjoining figure (a). Consider the magnetic field B at the centre of the arc. Would your answer be different if the wire were bent into a semi-circular arc of the same radius but in the opposite way a shown in figure (b)?

View SolutionA circular loop of wire is carring a current i (as shown in the figure). On applying a uniform magnetic field inward perpendicular to the plane of the loop, the loop

View SolutionA circular loop of radius a, carrying a current I, is placed in a tow dimensional magnetic field. The centre of the loop coincides with the centre of the filed The strenght of the magnetic field at the pariphery of the loop is B. find the magnetic force on the wire.

View Solution(a) Derive the expression for the magnetic field due to a current carrying coil of radius r at a distance x from the centre along the X-axis.
(b) A straight wire carrying a current of 5 A is bent into a semicular arc of radius 2 cm as shown in the fig. FInd th magnitude and direction of the magnetic field at the centre of the arc.

View Solution