Show that in a quandrilateral ABCD,AB+BC+CD+DA<2(BD+AC).
Video Solution
Text Solution
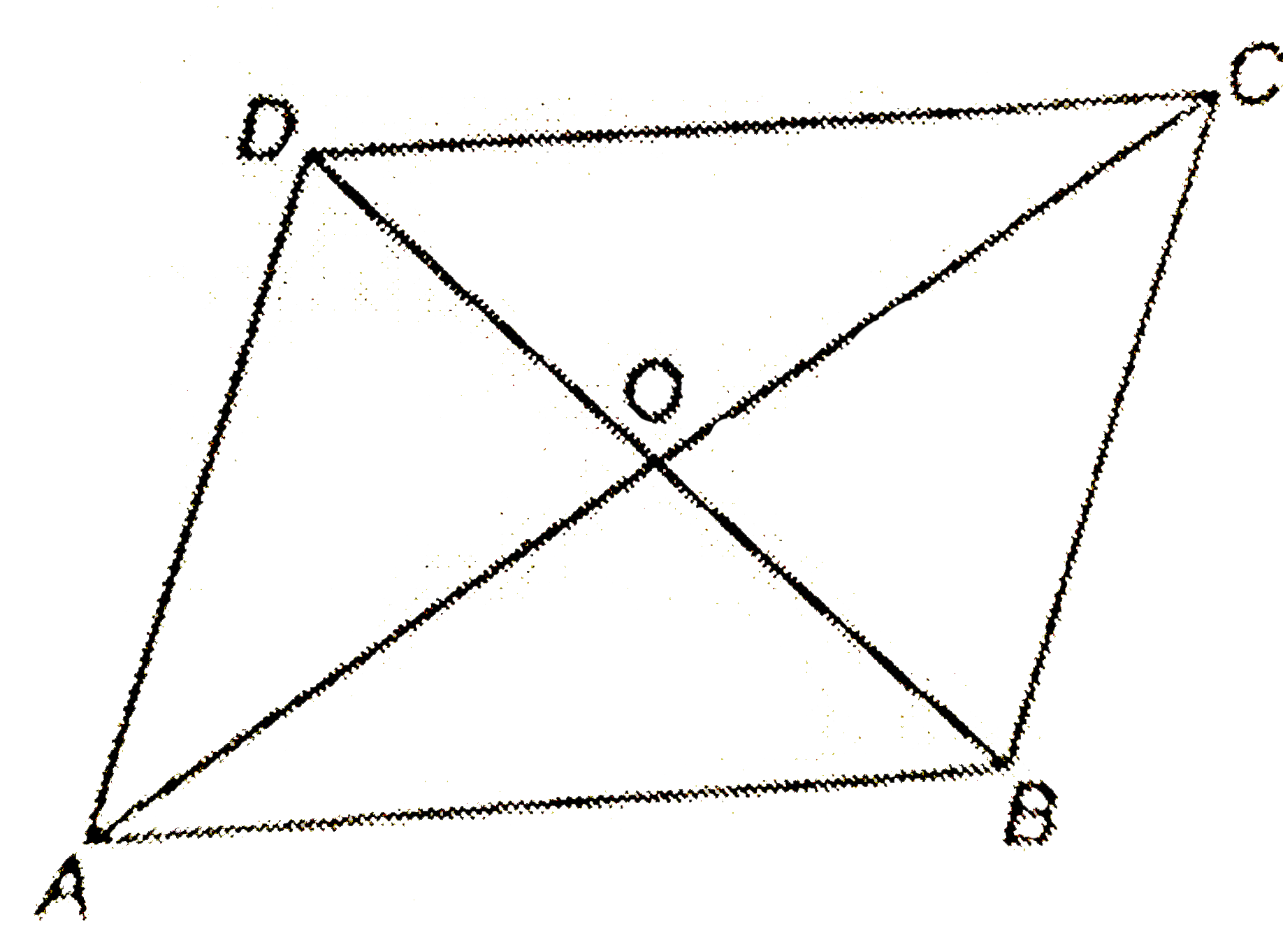
Given : A quadrilateral ABCD.
To prove : AB+BC+CD+DA<2(BD+AC)
Proof : In ΔAOB, we have
⇒ OA+OB>AB ...(1)
( ∵ sum of any two sides of a triangle is greater then the third side) In ΔBOC, we have
OB+OC>BC (same reason) ...(2)
In ΔCOD, we ahve
OC+OD>CD (same reason) ...(3)
In ΔDOA, we have
OD+OA>DA (same reason) ...(4)
Adding (1), (2), (3) and (4), we get
OA+OB+OB+OC+OC+OD+OD+OA>AB+BC+CD+DA
⇒ 2(OA+OB+OC+OD)>AB+BC+CD+DA
⇒ 2{(OA+OC)+(OC+OD)}>AB+BC+CD+DA
⇒ 2(AC+BD)>AB+BC+CD+DA
⇒ AB+BC+CD+DA<2(BD+AC)
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar5videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Exercise 7a35videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)10videos
SURFACE AREA AND VOLUME
Similar Questions
Show that in a quadrilateral ABCD ,AB+BC+CD+DA<2(BD+AC)
View SolutionShow that in a quadrilateral ABCD AB+BC+CD+DA>AC+BD
View SolutionIn a quadrilateral ABCD, show that (AB+BC+CD+DA)<2(BD+AC).
View SolutionIn a quadrilateral ABCD, show that
(AB+BC+CD+DA)>(AC+BD).View SolutionIn quadrilatcal ABCD, prove that AB+BC+CD+AD<2(BD+AC)
View SolutionIn a quadrilateral ABCD, AB= AD and CB = CD, prove that AC is perpendicular bisector of BD.
View SolutionABCD is a quadrilateral. Is AB+BC+CD+DA>AC+BD?
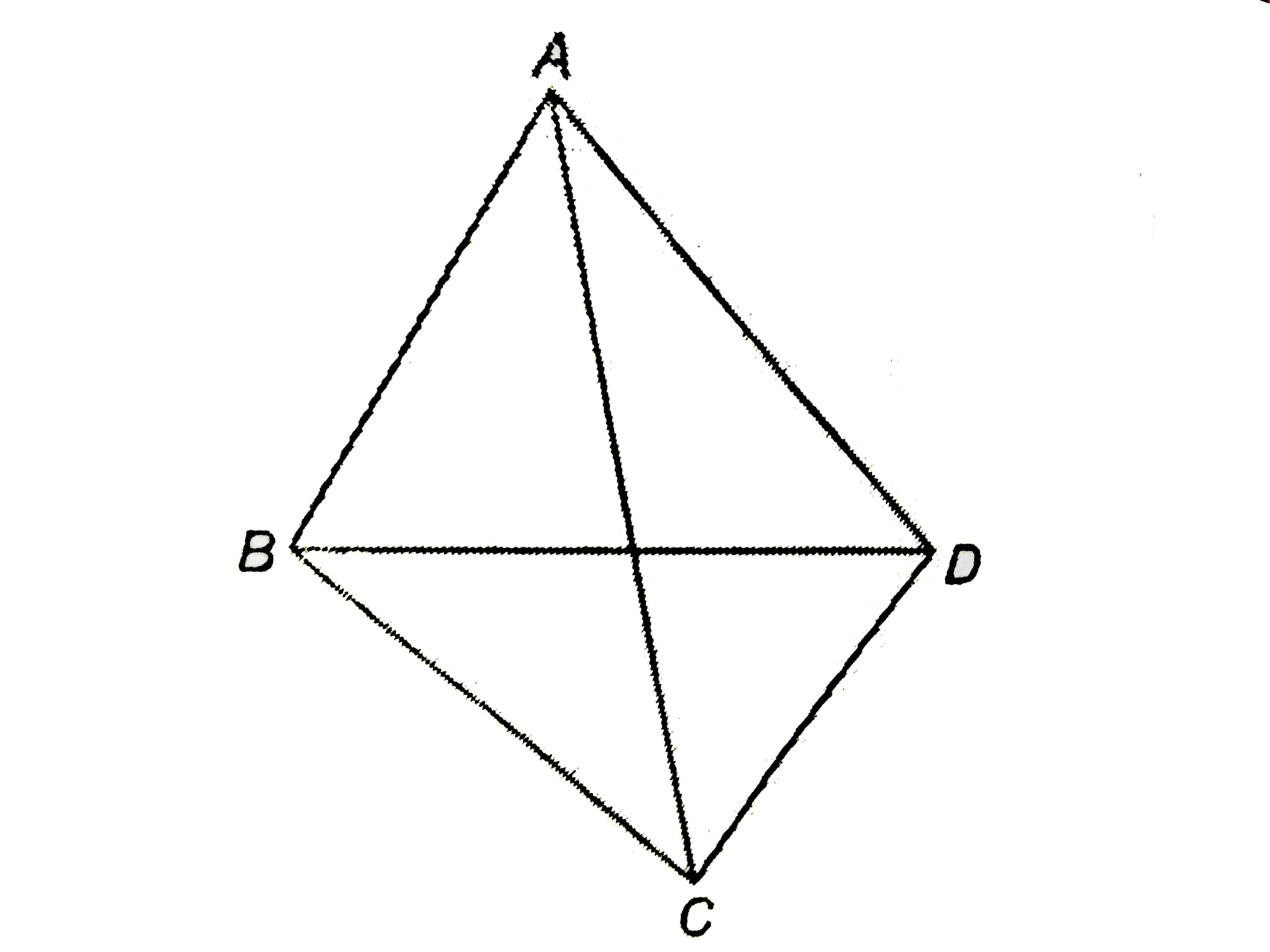
View SolutionThe given figure shows a quadrilateral ABCD. Prove that :
AB+BC+CD+DA>AC+BD
 View Solution
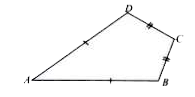
View SolutionIn quadrilateral ABCD, AB = AD and BC = CD. Show that ∠ABC=∠ADC.
 View Solution
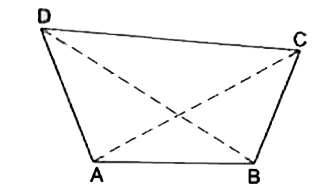
View SolutionABCD is a quadri,aterial prove that (AB+BC+CD+DA)>(AC+BD)
 View Solution
View Solution

