$3 y - 5 x < 30$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:N/a

Given inequation : $3 y - 5 x < 30$
Corresponding equation: $3 y - 5 x = 30$

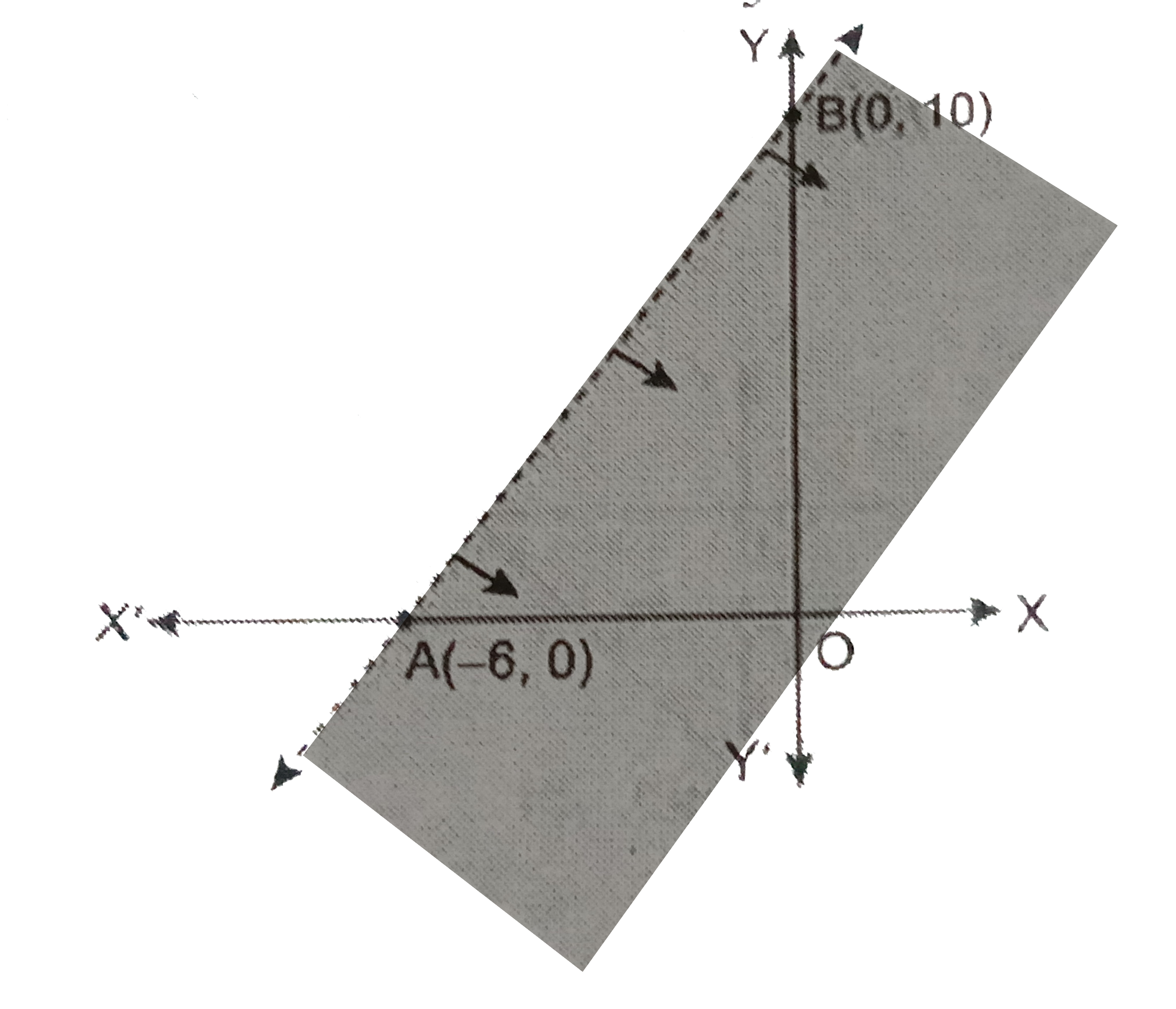
This line cuts the X-axis at point A(-6, 0) and Y-axis at point B (0, 10).
Draw a dotted line joining the points A and B . At point (0 ,o), from the inequation, $0 < 30$ ( True).
Now, shade that region of XY-plane divided by line AB in which (0, 0) lies.

|

Updated on:21/07/2023

Class 11 MATHS LINEAR INEQUALITIES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The set of values of x which satisfy $5 x + 2 < 3 x + 8$ and $\frac{x + 2}{x - 1} < 4$ is
A $(2, 3)$
B $(- \infty, 1) \cup (2, 3)$
C $(- \infty, 1)$
D $(1, 3)$
Question 2 - Select One
Solve for $y : \frac{4 y}{3} - 5 < 10$
A $y < 27 / 24$
B $t < 33 / 4$
C $y < 45 / 4$
D $y < 59 / 4$
Question 3 - Select One
Let f:R $\to$ R be a function defined as $f (x) = ⎧ ⎪ ⎪ ⎪ ⎪ ⎨ ⎪ ⎪ ⎪ ⎪ ⎩ \begin{matrix} 5 & if x \leq 1 a + b x & if 1 < x < 3 b + 5 x & if 3 \leq x < 5 30 & if x \geq 5 \end{matrix}$ then f is
Acontinuous at a=5 and b=5
Bcontiuous at a=-5 and b=10
Ccontinuous at a=0 and b=5
Dnot continuous for any values of a and b

Home
Ask Doubt
Profile