Text Solution
The correct Answer is:N/a
First inequation :
Corresponding equation :
This line passes through the points A(2, 0) and B (0, 4) . Join AB . At point (0, 0) , from the inequation (False) The solution of this inequation is that region of XY-place divided by line AB in which (0, 0) does not lie.
Second inequation :
Corresponding equation :
This line thorugh the points C(3, 0) and D (0, 3). Join CD. At point (0, 0), from the inequation, 0le3 (True) The solution of this inequation is that region of XY-plane divided by line CD in which (0, 0) lies.
Third inequation :
Corresponding equation:
This line paaes through the points C (3, 0) and E (0, -2). Join CE
At point (0, o), from the inequation, 0 le 6 (True)
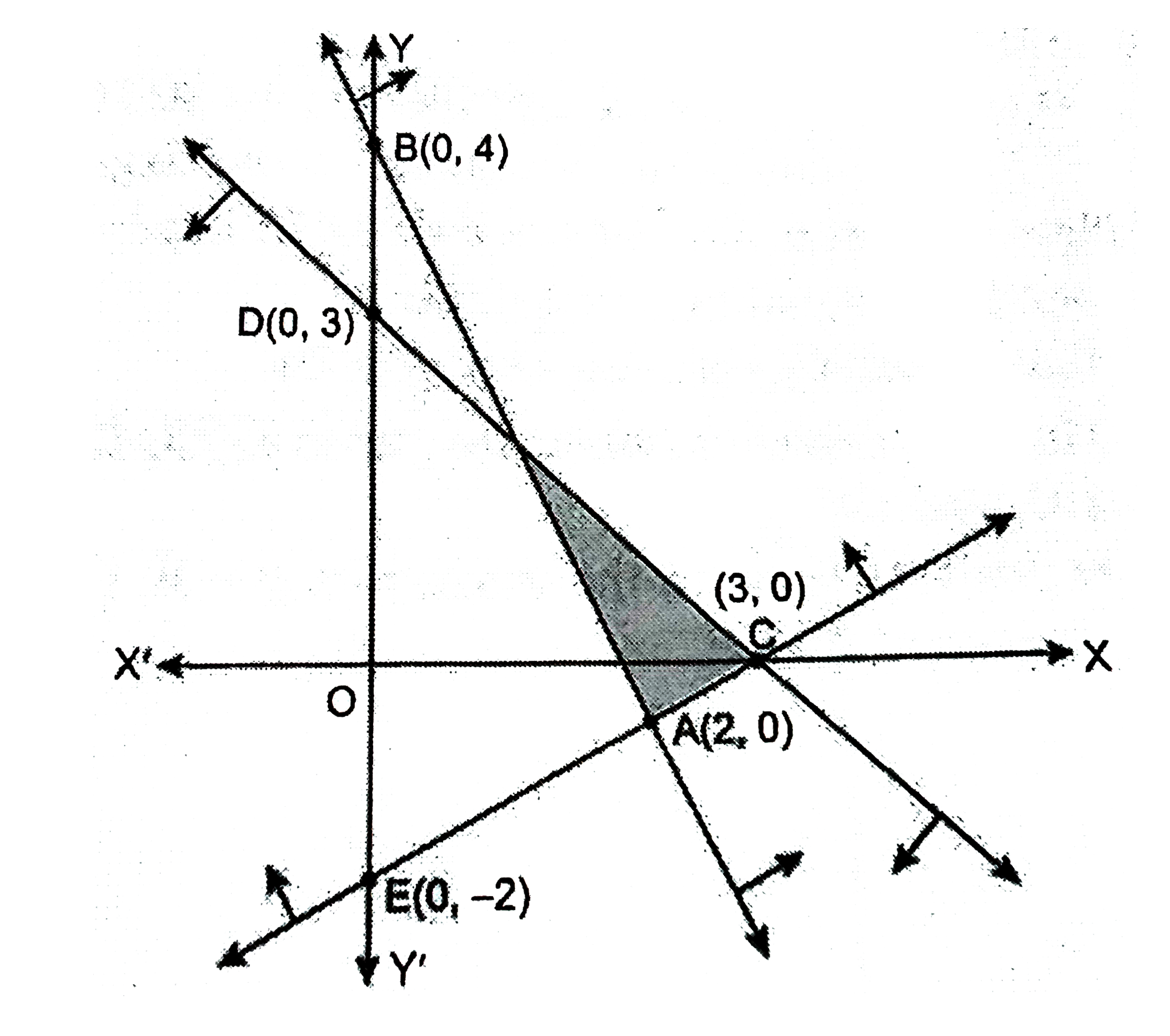
The solution of this inequation is that region of XY-plane divided by line CE in which (0, 0) lies .
The solution of given inequations is shown by the shaded region
|
Topper's Solved these Questions
- LINEAR INEQUALITIESBOOK - NAGEEN PRAKASHANCHAPTER - LINEAR INEQUALITIESEXERCISE - MISCELLANEOUS EXERCISE
14 Videos
- LINEAR INEQUALITIESBOOK - NAGEEN PRAKASHANCHAPTER - LINEAR INEQUALITIESEXERCISE - EXERCISE
76 Videos
- LIMITS AND DERIVATIVESBOOK - NAGEEN PRAKASHANCHAPTER - LIMITS AND DERIVATIVESEXERCISE - Miscellaneous Exercise
30 Videos
- MATHEMATICAL REASONING BOOK - NAGEEN PRAKASHANCHAPTER - MATHEMATICAL REASONING EXERCISE - Misellaneous exercise
7 Videos
Similar Questions
Knowledge Check
The solution set of the inequalities 2x+y≥4,x+y≤x,2x−3y≤6 is
A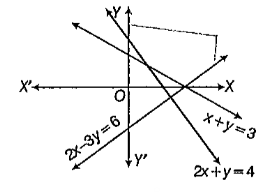 B
B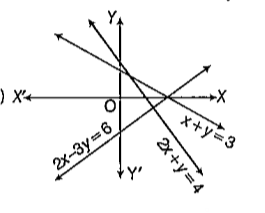 C
C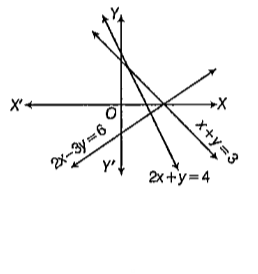 D
D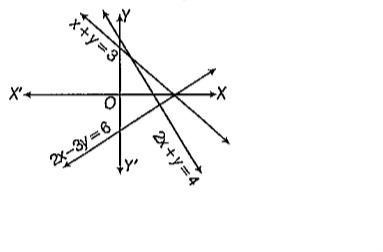
Minimize z=x+3y
Subject to
x+y≤5
2x+y≥4
x+5y≥5
x≥3
y≤3
View Solutionx+y≤5,4x+y≥4,x+5y≥5,x≤4,y≤3.
View Solution3x+2y≤14,3x+y≤9,x,y≥0
View Solution2x+y≤6,x+2y≤8,x≥0,y≥0
View Solution2x+y≤6,x+2y≤8,x≥0,y≥0
View SolutionMaximize z=4x+5y, subject to
2x+y≥7,2x+3y≤15,x≤3,x≥0,y≥0
View SolutionMaximise and Minimise :
Z=4x+3y−7 subject to the constraints:x+y≤10,x+y≥3,x≤8,y≤9,x,y≥0
View Solution
