Question

Find the area of the region
enclosed by the parabola $x^2=y$
, the line $y=x+2$
and the x-axis.

Explanation

Given curve is a parabola whose vertex is (0, 0).

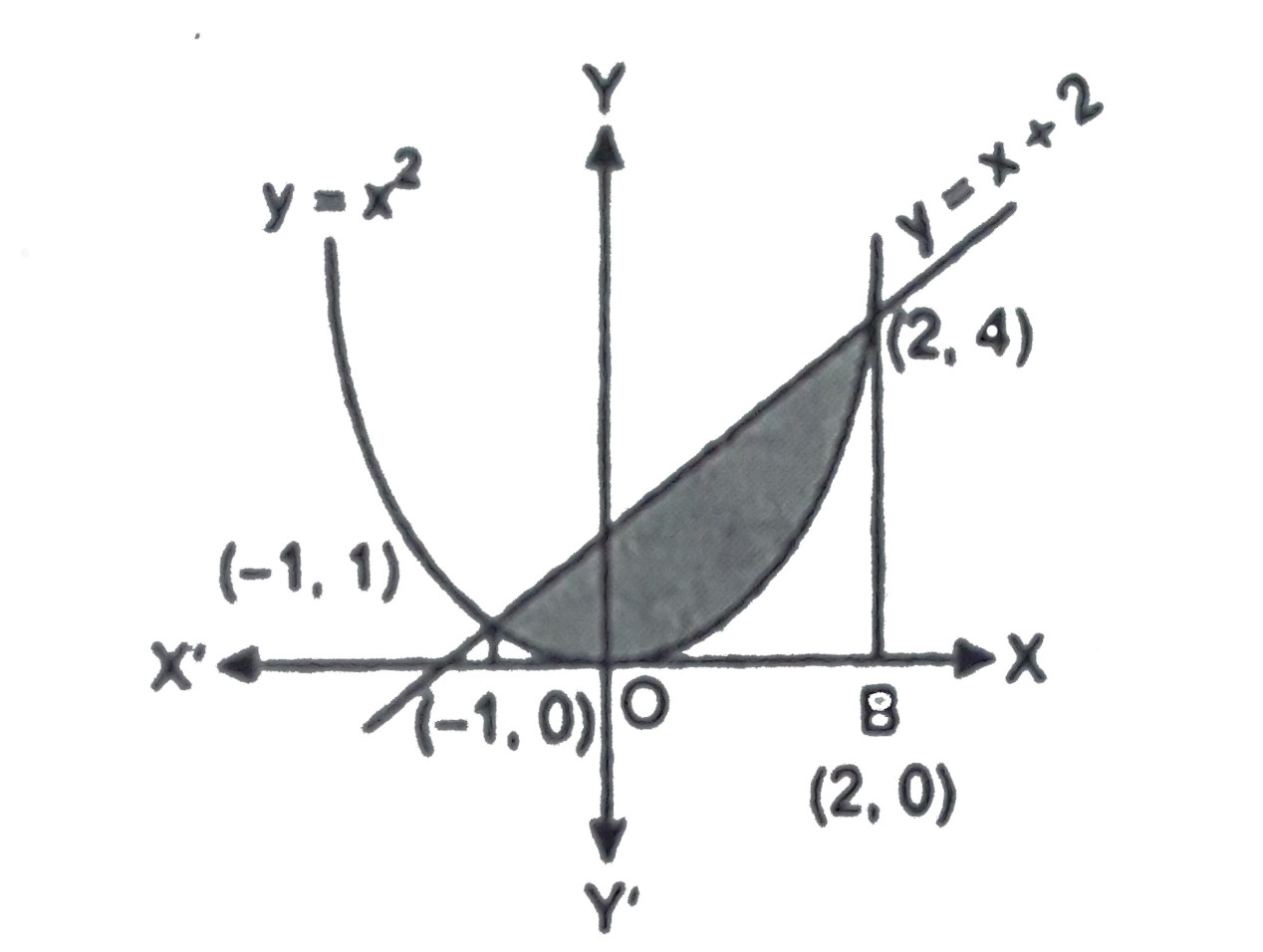
`y=x^(2) " " ` …(1)
and equation of line is
`y=x+2 " " `…(2)
On solving,
`x^(2)-x-2=0`
`implies(x-2)(x+1)=0`
`implies x=2 " or " -1`
When, `x=2,y=2+2=4`
When, `x= -1,y=(-1)+2=1`
Therefore, the points of intersection of parabola and the line are (-1, 1) and (2, 4).
` :. ` Required area `=int_(-1)^(2)(x+2)dx-int_(_1)^(2)x^(2)dx`
`=[(x^(2))/(2)+2x]_(-1)^(2)-[(x^(3))/(3)]_(-1)^(2)`
`=(2^(2))/(2)+2xx2-[((-1)^(2))/(2)+2(-1)]-(1)/(3)[2^(3)-(-1)^(3)]`
`=6-(1)/(2)+2-3=(9)/(2)` sq. units.