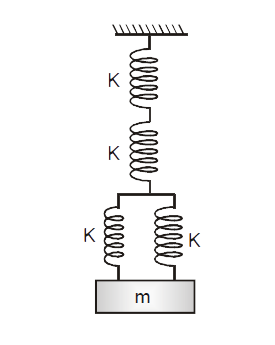
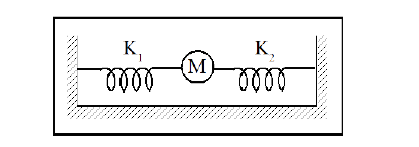
Give the expression for frequency of oscillation of a mass body as shown in the fig.
Effective spring constant =1k=1k1+k2+1k3
i.e. 1k=(k1+k2+k3)(k1+k2)k3
Period of oscillation T=2π√m(k1+k2+k3)(k1+k2)k3
Since frequency of oscillation n=1T=12π√(k1+k2)k3m(k1+k2+k3).
Topper's Solved these Questions
- OSCILLATIONSBook:SUBHASH PUBLICATIONChapter:OSCILLATIONSExercise:FIVE MARKS QUESTIONS WITH ANSWERS
Explore 4 Videos
- MOTION IN PLANEBook:SUBHASH PUBLICATIONChapter:MOTION IN PLANEExercise:NUMERICALS WITH SOLUTIONS
Explore 47 Videos
- PHYSICAL WORLDBook:SUBHASH PUBLICATIONChapter:PHYSICAL WORLDExercise:TWO MARKS QUESTIONS WITH ANSWERS
Explore 30 Videos
Similar Questions
Knowledge Check
The frequency of oscillation of the springs shown in the figure will be
 A12π√KmB12π√(K1+K2)K1K2C12π√kmD12π√K1K2m(K1+K2)
A12π√KmB12π√(K1+K2)K1K2C12π√kmD12π√K1K2m(K1+K2)The frequency of oscillation of the spring shown in the figure will be
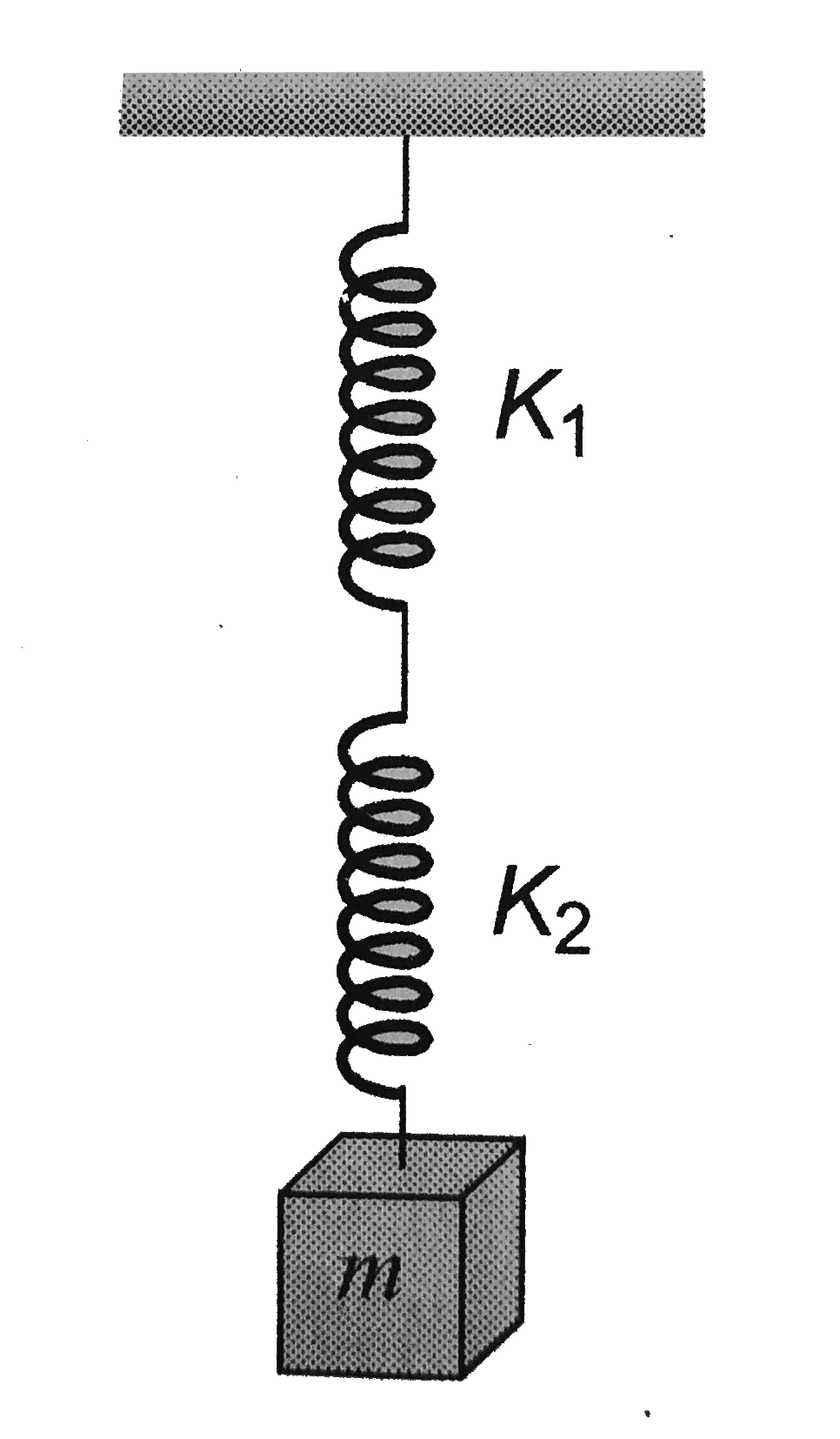 A12π√KmB12π√(K1+K2)mK1K2C2π√KmD12π ⎷(K1K2m(K1+K2))
A12π√KmB12π√(K1+K2)mK1K2C2π√KmD12π ⎷(K1K2m(K1+K2))The frequency of vertical oscillations of the three spring-mass system, shown in figure, is
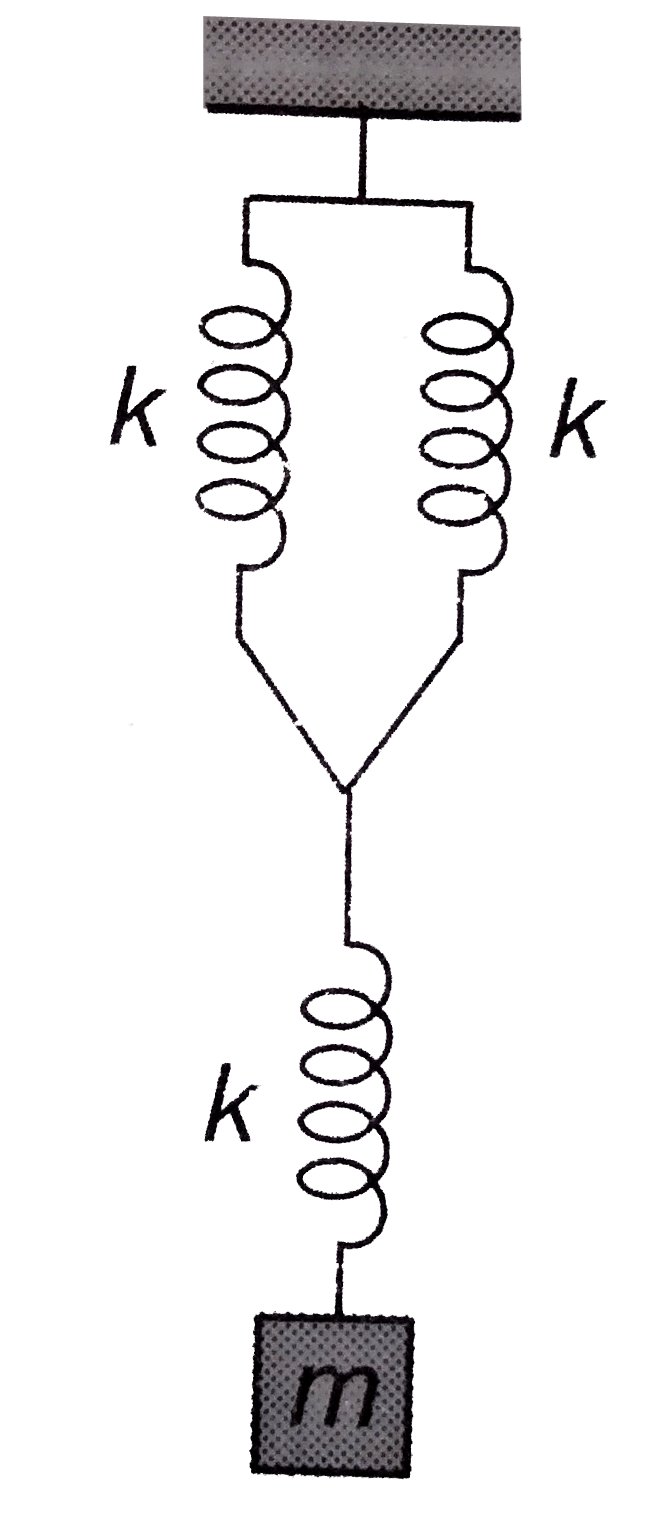 A12π√3k2mB12π√2k3mC12π√2kmD12π√k3m
A12π√3k2mB12π√2k3mC12π√2kmD12π√k3m
A mass m is suspended from a spring. Its frequency of oscillation is f. The spring is cut into two halves and the same mass is suspended from one of the pieces of the spring. The frequency of oscillation of the mass will be
View SolutionA rigid rod of mass m with a ball of mass M attached to the free end is restrained to oscillate in a vertical plane as shown in the figure. Find the natural frequency of oscillation.

View SolutionA rigid rod of mass m with a ball of mass M attached to the free end is restrained to oscillate in a vertical plane as shown in the figure. Find the natural frequency of oscillation.

View SolutionFind the expressions for time period and frequency
(i) in the oscillations of a liquid in a U tube
(ii) in the oscillations of a floating cylinder
View SolutionA mass m is suspended from a spring. Its frequency of oscillation is f. The spring is cut into two halves and the same mass is suspended from one of the pieces of the spring. The frequency of oscillation of the mass will be
View Solution