Find the points of inflection for f(x)=sinx f(x)=3x^4-4x^3

Concavity and convexity :
if $f'' (x) > 0 \forall x \in (a, b)$ then the curve y=f(x) is concave up ( or convex down) in (a,b) and $if f'' (x) < 0 \forall x \in (a, b)$ then the curve y=f(x) is concave down (or convex up ) in (a,b)

Inflection point :
The point where concavity of the curve changes is known as point of inflection (at inflection point $f'' (x)$ is equal to 0 or undefined)

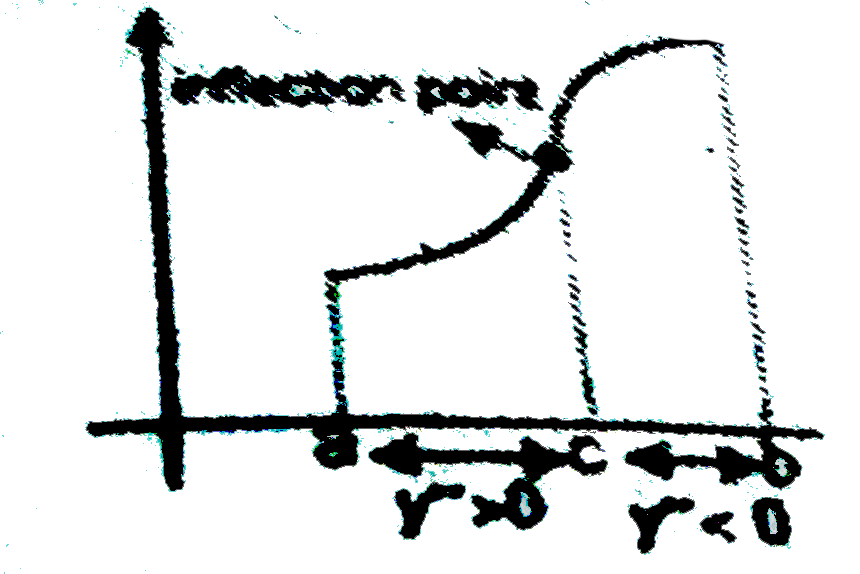
Number of point of inflection for $f (x) = {(x - 1)}^{3} {(x - 2)}^{2}$ is :
A $1$
B $2$
C $3$
D $4$
An equation of tangent line at an inflection point of $f (x) = x^{4} - 6 x^{3} + 12 x^{2} - 8 x + 3$ is
A $y = 3 x + 4$
B $y = 4$
C $y = 3 x + 2$
Dnone of these

Find the points of inflection for $f (x) = sin x$ $f (x) = 3 x^{4} - 4 x^{3}$