Veriation of momentum of perticle (p) with associated de-Broglie wavelength (λ) is shown correctly by
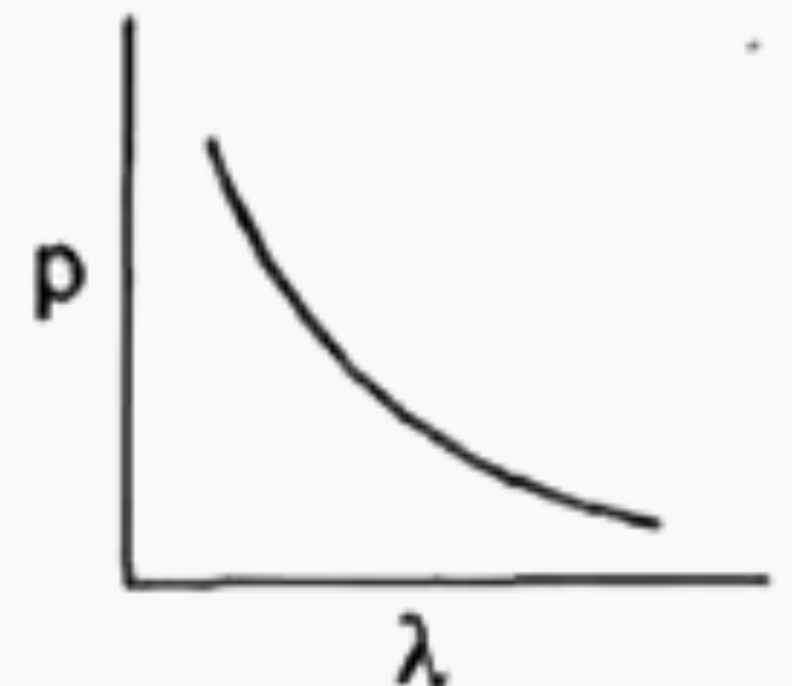
The correct Answer is:A
To solve the problem of how the momentum p of a particle varies with its associated de-Broglie wavelength λ, we can follow these steps:
Step 1: Understand the Relationship
The de-Broglie wavelength λ of a particle is given by the formula:
λ=hp
where h is Planck's constant and p is the momentum of the particle.
Step 2: Rearranging the Formula
From the de-Broglie wavelength formula, we can express momentum in terms of wavelength:
p=hλ
Step 3: Analyze the Relationship
From the equation p=hλ, we can see that:
- As the wavelength λ increases, the momentum p decreases.
- Conversely, as the wavelength λ decreases, the momentum p increases.
Step 4: Plotting the Graph
If we were to plot this relationship:
- The x-axis would represent the de-Broglie wavelength λ.
- The y-axis would represent the momentum p.
The graph would show a hyperbolic relationship, indicating that as λ increases, p decreases.
Step 5: Conclusion
From our analysis, we can conclude that the correct variation of momentum p with the associated de-Broglie wavelength λ is that momentum decreases as wavelength increases. Therefore, the correct option is the one that reflects this inverse relationship.
The de-Broglie wavelength λ of a particle is given by the formula:
λ=hp
where h is Planck's constant and p is the momentum of the particle.
From the de-Broglie wavelength formula, we can express momentum in terms of wavelength:
p=hλ
From the equation p=hλ, we can see that:
- As the wavelength λ increases, the momentum p decreases.
- Conversely, as the wavelength λ decreases, the momentum p increases.
If we were to plot this relationship:
- The x-axis would represent the de-Broglie wavelength λ.
- The y-axis would represent the momentum p.
From our analysis, we can conclude that the correct variation of momentum p with the associated de-Broglie wavelength λ is that momentum decreases as wavelength increases. Therefore, the correct option is the one that reflects this inverse relationship.
Topper's Solved these Questions
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTECHAPTER - ALTERNATING CURRENT EXERCISE - Try Yourself
15 Videos
- ALTERNATING CURRENT BOOK - AAKASH INSTITUTECHAPTER - ALTERNATING CURRENT EXERCISE - Assignment (Section - A) ( Objective Type Questions ( One option is correct))
34 Videos
- ATOMSBOOK - AAKASH INSTITUTECHAPTER - ATOMSEXERCISE - ASSIGNMENT SECTION J (Aakash Challengers )
4 Videos
Similar Questions
Knowledge Check
The momentum of a particle associated with de-Broglie's wavelength of 6Å is
A1.1×10−24kgms−1B1.1×1034kgms−1C39.6×10−34kgms−1D39.6×10−24kgms−1De Broglie wavelength λ is proportional to
A1√E for photons and 1/E for particlesB1/E for photons and 1√E for particlesC1/E for both photons and particles in motionD1√E for both photons and particles.The momentum of a particle having de-Broglie wavelength of 6Å
A1.1×1034kg−m/sB39.6×10−34kg−m/sC1.1×10−24kg−m/sD39.6×10−24kg−m/s
The de Broglie wavelength λ of a particle
View SolutionThe de Broglie wavelength λ of a particle
View SolutionWhich of the following figure represents the variation of particle momentum and the associated de - Broglie wavelength ?
View SolutionMinimum de-Broglie wavelength is associated with.
View SolutionWhich of the following figure represents the variation of particle momentum and the associated de - Broglie wavelength ?
View Solution
