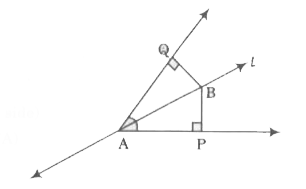
Line l is the bisector of an angle ∠A and B is any point on li. BP and BQ are perpendicular forms B to the arms of ∠A. Show that:
△APB≅△AQB
(ii) BP = BQ or B is equidisitant from the arms of ∠A.
 Solution in Kannada
Solution in Kannada
Step by step video & image solution for Line l is the bisector of an angle angleA and B is any point on li. BP and BQ are perpendicular forms B to the arms of angleA. Show that: triangleAPB ~= triangleAQB (ii) BP = BQ or B is equidisitant from the arms of angleA. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLES BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - TRIANGLES EXERCISE - EXERCISE 5.2
8 Videos
- TRIANGLES BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - TRIANGLES EXERCISE - EXERCISE 5.3
5 Videos
- SURFACE AREAS AND VOLUMES BOOK - CPC CAMBRIDGE PUBLICATIONCHAPTER - SURFACE AREAS AND VOLUMES EXERCISE - EXERSICE 13.8
10 Videos
Similar Questions
In an isosceles ABC, with AB = AC, the bisectors of ∠B and ∠C intersect each other at O. Joint A to O. Show that:
(ii) OB = OC
(ii) AO bisects ∠A
View Solution△ABC and △DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that:
(i) △ABD≅△ACD
(ii) △ABP≅△ACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC.
View SolutionFind the volume of a sphere whose radius is
(i) 7 cm,
(ii) 0.63m
View Solutionline l is the bisector of an angle ∠A and∠Bis any point on l. BP and BQ are perpendiculars from B to the arms of ∠A. Show that:
(i) ΔAPB≅ΔAQB
(ii) BP = BQ or B is equidistant from the arms of ∠A

View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionIn the following figure, ¯¯¯¯¯¯BD is produced to E. If ¯¯¯¯¯¯AD is the angle bisector of ∠A, then find the ∠BCD.
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C12_E03_005_Q01.png" width="80%"gt
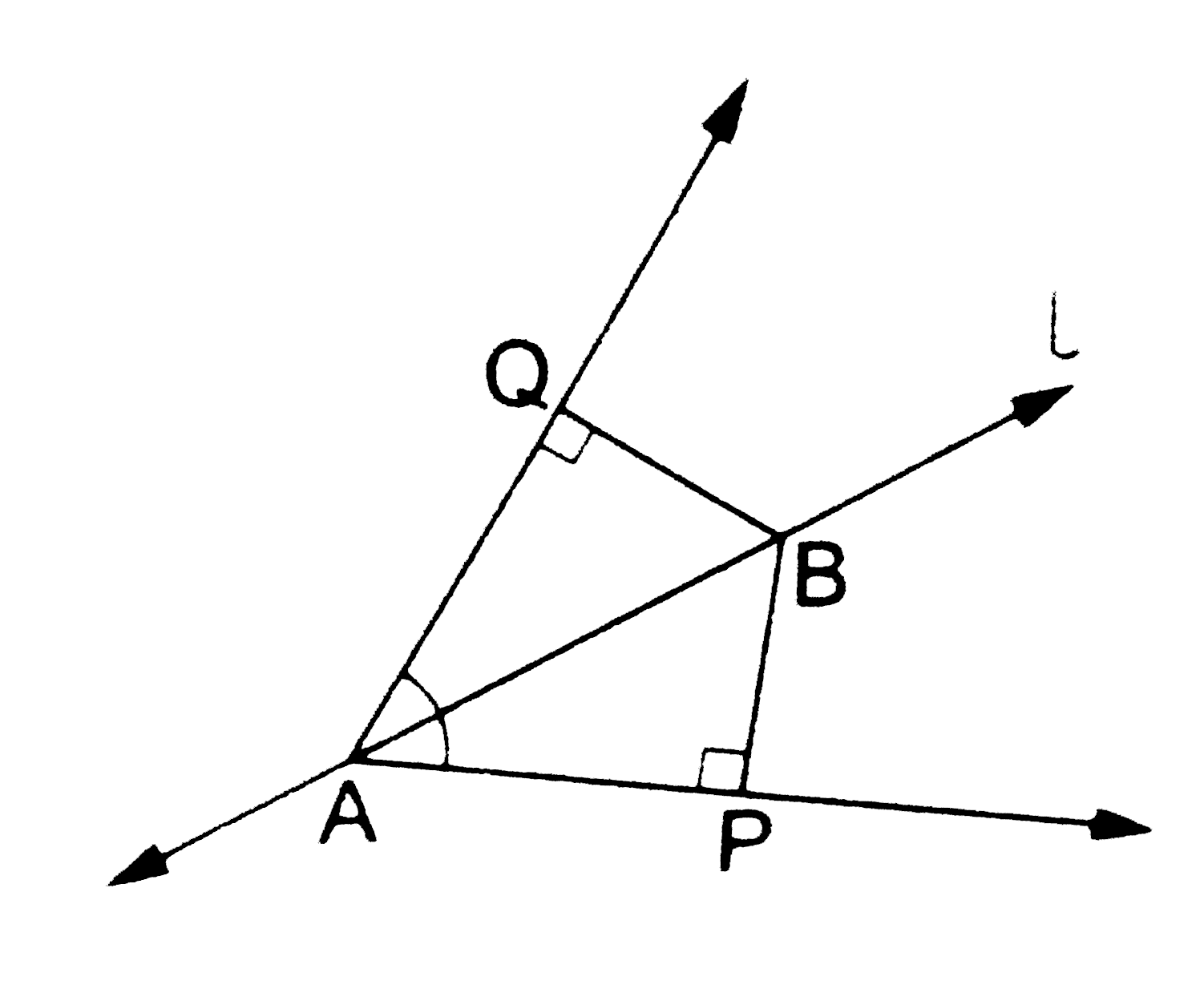
View SolutionIn the given figure, line l is the bisector of an angle ∠A and B is any point on l. If BP and BQ are perpendiculars from B to the arms of ∠A, show that
(i) ΔAPB≅ΔAQB
(ii) BP = BQ, i.e., B is equidistant from the arms of ∠A.
View Solutionरेखा l कोण A की समद्विभाजक है तथा l पर कोई बिंदु B है| BP और BQ बिंदु B से ∠Aकी भुजाओं पर लम्ब है तो दिखाइये कि-

(i) ΔAPB≅ΔAQB
(ii) BP=BQ
View SolutionangleA The angular line of y is l. B is any point on l angleA The sides of the line are the drawn verticals (see image). (i) triangleAPB cong triangleAQB (ii) BP = BQ or B u angleA Show that the sides of the plane are straight.
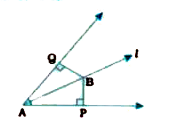
View SolutionRay 1 is the bisector of an angle ∠ A and B is any point on I. BP and BQ are perpendiculars from B to the arms of ∠ A (see the given figure). Show that:
(i) △APB=△AQB
(ii) BP = BQ or B is equidistant from the arms of ∠A
View Solutionਰੇਖਾ l, ∠A ਨੂੰ ਸਮਦੁਭਾਜਿਤ ਕਰਦੀ ਹੈ ਅਤੇ B, l ਉੱਤੇ ਕੋਈ ਬਿੰਦੂ ਹੈ।BP ਅਤੇ BQ ਬਿੰਦੂ B ਤੋਂ ∠A ਦੀਆਂ ਬਾਹਵਾਂ ਉੱਤੇ ਲੰਬ ਹਨ (ਵੇਖੋ ਚਿੱਤਰ 7.20)। ਸਿੱਧ ਕਰੋ (i) △APB≅△AQB (ii) BP = BQ ਜਾਂ B ਬਿੰਦੂ ਕੋਣ A ਦੀਆਂ ਭੁਜਾਵਾਂ ਤੋਂ ਸਮਾਨ ਦੂਰੀ 'ਤੇ ਹੈ।
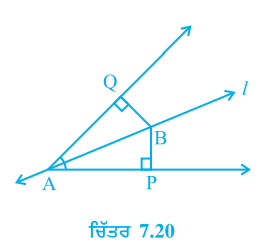
View SolutionLine l is the bisector of an angle ∠A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠A (see Fig. 7.20). Show that: (i) △APB≅△AQB (ii) BP = BQ or B is equidistant from the arms of ∠A.
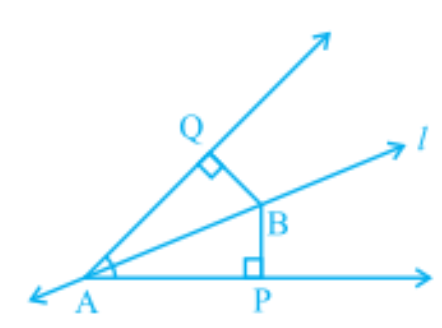
View Solution
