A shell is fired vertically upwards with a velocity v1 from the deck of a ship travelling at a speed of v2 . A person on the shore observes the motion of the shell as parabola. Its horizontal range is given by:
2v2g
2v1v22g
2v1v2g
2v21v22g
The correct Answer is:C
To solve the problem, we need to analyze the motion of the shell fired vertically upwards from the deck of a moving ship. The shell's motion can be understood as a combination of vertical and horizontal motions, leading to a parabolic trajectory as observed from the shore.
1. Understand the Initial Conditions:
- The shell is fired vertically upwards with an initial velocity v1.
- The ship is moving horizontally with a velocity v2.
2. Determine the Velocity Components:
- The velocity of the shell with respect to the ground can be expressed as:
→vshell=v2^i+v1^j
- Here, v2 is the horizontal component (along the x-axis) and v1 is the vertical component (along the y-axis).
3. Analyze the Horizontal Motion:
- The horizontal motion of the shell is uniform (no horizontal acceleration), so the horizontal displacement x after time t is given by:
x=v2⋅t
4. Analyze the Vertical Motion:
- The vertical motion is subject to gravitational acceleration. The vertical displacement y after time t is given by the equation of motion:
y=v1⋅t−12gt2
- Since the shell returns to the same vertical level from which it was fired, we set y=0:
0=v1⋅t−12gt2
5. Solve for Time of Flight:
- Rearranging the equation gives:
v1⋅t=12gt2
- Dividing both sides by t (assuming t≠0):
v1=12gt
- Solving for t gives:
t=2v1g
6. Substitute Time into Horizontal Displacement:
- Substitute t back into the horizontal displacement equation:
x=v2⋅t=v2⋅(2v1g)
- Therefore, the horizontal range R of the shell is:
R=2v1v2g
Final Answer:
The horizontal range of the shell is given by:
R=2v1v2g
- The shell is fired vertically upwards with an initial velocity v1.
- The ship is moving horizontally with a velocity v2.
- The velocity of the shell with respect to the ground can be expressed as:
→vshell=v2^i+v1^j
- Here, v2 is the horizontal component (along the x-axis) and v1 is the vertical component (along the y-axis).
- The horizontal motion of the shell is uniform (no horizontal acceleration), so the horizontal displacement x after time t is given by:
x=v2⋅t
- The vertical motion is subject to gravitational acceleration. The vertical displacement y after time t is given by the equation of motion:
y=v1⋅t−12gt2
- Since the shell returns to the same vertical level from which it was fired, we set y=0:
0=v1⋅t−12gt2
- Rearranging the equation gives:
v1⋅t=12gt2
- Dividing both sides by t (assuming t≠0):
v1=12gt
- Solving for t gives:
t=2v1g
- Substitute t back into the horizontal displacement equation:
x=v2⋅t=v2⋅(2v1g)
- Therefore, the horizontal range R of the shell is:
R=2v1v2g
The horizontal range of the shell is given by:
R=2v1v2g
Similar Questions
A ball is thrown vertically upwards with a speed of 9.8 m/s from the ground. Draw the x-t, v-t and a-t graph for its motion.
View SolutionA bullet is fired vertically upwards with velocity v form the surface of a spherical planet. If the escape velocity from the planet is vesc=v√N, then the value of N is (ignore energy loss due to atmosphere)
View Solution
Knowledge Check
- Question 1 - Select One
A shell is fired vertically upwards with a velocity v1 from a trolley moving horizontally with velocity v2. A person on the the ground observes the motion of the shell as a parabole, whose horizontal range is
A2v21v2gB2v21gC2v22gD2v1v2g - Question 2 - Select One
If the range of a gun which fires a shell with muzzle speed V is R , then the angle of elevation of the gun is
Acos−1(V2Rg)Bcos−1(gRV2)C12(V2Rg)D12sin−1(gRV2) - Question 3 - Select One
A shell is fired vertically from the earth with speed vesc/N, where N is some number greater than one and vesc is escape speed on the earth. Neglecting the rotation of the earth and air resistance, the maximum altitude attained by the shell will be (RE is radius of the earth):
AREN2−1BREN2CNREN2−1DN2REN2−1
A body is projected vertically upwards with a speed v0. Find the magnitude of time average velocity of the body during its ascent.
View SolutionA person travels along a straight road for the first half length with a velocity v1 and the second half length with velocity v2. What is the mean velocity of the person ?
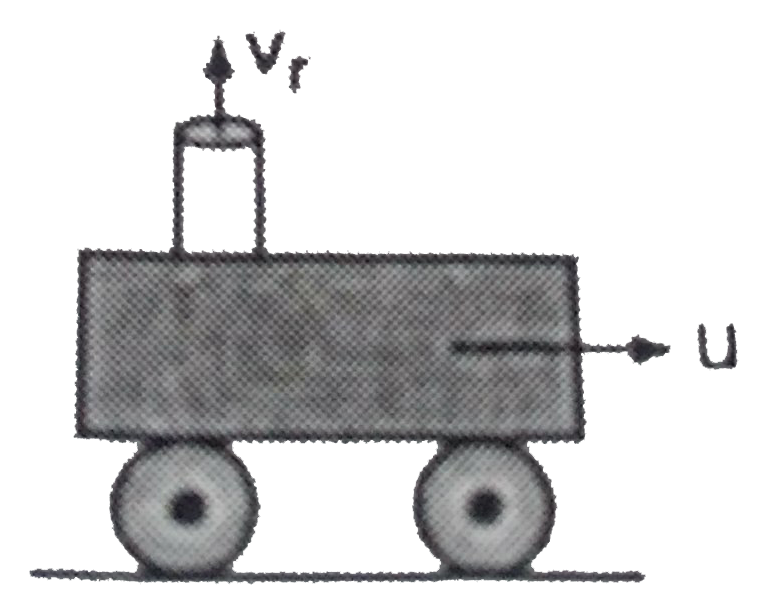
View SolutionA shell is fired vertically from a cannon which is travelling at constant speed u=30km/hr. the projectile leaves the connon with a velocity vr=20 m/s relative to the cannon . Show that the shell will land on the vehicle at the gun location and calcu late the distance by the vehicle during the flight of shell.
 View Solution
View SolutionA trolley is moving with velocity v1 in the horizontal direction . A bullet is fired from his trolley in the upward direction with a speed v2 . Observer is standing on the ground and observes the bullet to be moving in a parabolic path. What will be the range of projectile ?
View SolutionA bullet is fired vertically upwards with an initial velocity of 50 ms–1. If g = 10 ms–2, what is the ratio of the distances travelled by the bullet during the first and the last second of its upward motion ?
View Solution

