If the sequence t1,t2,t3,… are in A.P. then the sequence t6,t12,t18,… is Solution in Tamil
a Geometric Progression
an Arithmetic Progression
neither an Arithmetic Progression nor a Geometric Progression
a constant sequence
The correct Answer is:B
Step by step video, text & image solution for If the sequence t_(1), t_(2), t_(3), … are in A.P. then the sequence t_(6), t_(12), t_(18),… is by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - PREMIERS PUBLISHERSCHAPTER - NUMBERS AND SEQUENCESEXERCISE - UNIT EXERCISE12videos
NUMBERS AND SEQUENCES
- BOOK - PREMIERS PUBLISHERSCHAPTER - NUMBERS AND SEQUENCESEXERCISE - THINKING CORNER13videos
NUMBERS AND SEQUENCES
- BOOK - PREMIERS PUBLISHERSCHAPTER - NUMBERS AND SEQUENCESEXERCISE - EXERCISE 2. 914videos
NUMBERS AND SEQUENCES
- BOOK - PREMIERS PUBLISHERSCHAPTER - MENSURATIONEXERCISE - OTHER IMPORTANT OBJECTIVE TYPE QUESTIONS16videos
MENSURATION
- BOOK - PREMIERS PUBLISHERSCHAPTER - RELATIONS AND FUNCTION EXERCISE - OTHER IMPORTANT OBJECTIVE TYPE QUESTIONS25videos
RELATIONS AND FUNCTION
Similar Questions
Prove that n2−n divisible by 2 for every positive integer n.
03:35View SolutionWhen a positive integer is divided by 3
What are the possible remainders?02:27View SolutionFind the sum of the following series
1+2+3+…+6000:37View SolutionMatch the following:
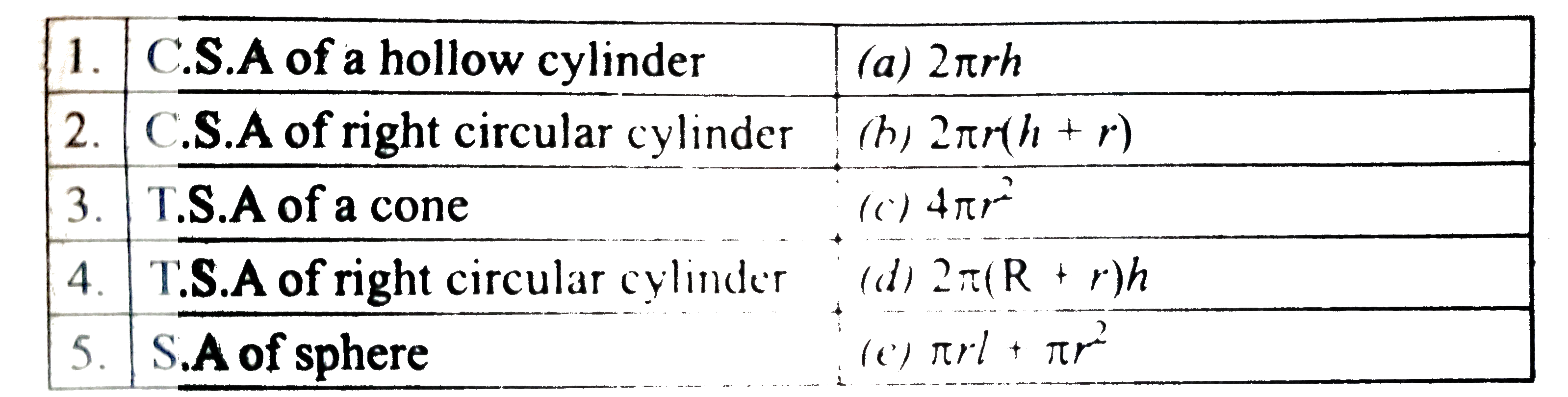 00:43View Solution
00:43View SolutionIf n(A×B)=24andA={1,3,5,7}then n (B) is
01:35View SolutionSequence {t_(n)} of positive terms is a G.P If t_(6)2,5,t_(14) form another G.P in that order then the product t_(1)t_(2)t_(3).........t_(18)t_(19) is equal to
02:29View SolutionLet {tn} be a sequence of integers in G.P. in which t4:t6=1:4andt2+t5=216. . Then t1is 12 b. 14 c. 16 d. none of these
02:23View SolutionWrite the indicated terms in each of the following sequences whose nth terms are: tn=n2+1:t1,t2,t3
01:20View SolutionWrite the indicated terms in each of the following sequences whose nth terms are: tn=2n−36:t1,t2,t3
01:34View SolutionIf for an A.P.,d=5, then t18−t13=….
01:07View Solutionनिम्न अनुक्रम के पहले छः पर ज्ञात कीजिए |
T1=12,T2=−1,Tn+2=Tn.Tn+1View SolutionWrite the correct number in the given boxes from the following A.P.
−3,−8,−13,−18,…..
Here, t1=□,t2=□,t3=□,t4=□,t2−t1=□,t3−t2=□∴a=□,d=□01:51View SolutionIn an A.P., if t18−t14=32, then d=
06:44View SolutionIf the sequence t1,t2,t3,… are in A.P. then the sequence t6,t12,t18,… is
06:27View SolutionThe area of triangle formed by the points points and t1,t2 and t3 on y3 =4ax is k|(t1−t2)(t2−t3)(t3−t1)| then K =
01:49View SolutionSuppose
(x+a)n=T0+T1+T2+…+Tn
Then (T0−T2+T4−…)2+(T1−T3+T5−….)2 is equal to02:08View Solution

