Determine the entries denoted by a11,a22,a33,a44 from the matrix :
⎛⎜
⎜
⎜
⎜
⎜⎝213459−4√7352897014⎞⎟
⎟
⎟
⎟
⎟⎠ Solution in Tamil
Step by step video & image solution for Determine the entries denoted by a_(11),a_(22),a_(33),a_(44) from the matrix : {:((2,1,3,4),(5,9,-4,sqrt(7)),(3,(5)/(2),8,9),(7,0,1,4)):} by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ALGEBRABOOK - PREMIERS PUBLISHERSCHAPTER - ALGEBRAEXERCISE - SOLUTION TO ACTIVITIES
7 Videos
- ALGEBRABOOK - PREMIERS PUBLISHERSCHAPTER - ALGEBRAEXERCISE - OTHER IMPORTANT OBJECTIVE TYPE QUESTIONS
28 Videos
- ALGEBRABOOK - PREMIERS PUBLISHERSCHAPTER - ALGEBRAEXERCISE - SOLUTION THINKING CORNER (Say True or False:)
1 Videos
- CO-ORDINATE GEOMETRY BOOK - PREMIERS PUBLISHERSCHAPTER - CO-ORDINATE GEOMETRY EXERCISE - Other Important Objective
25 Videos
Similar Questions
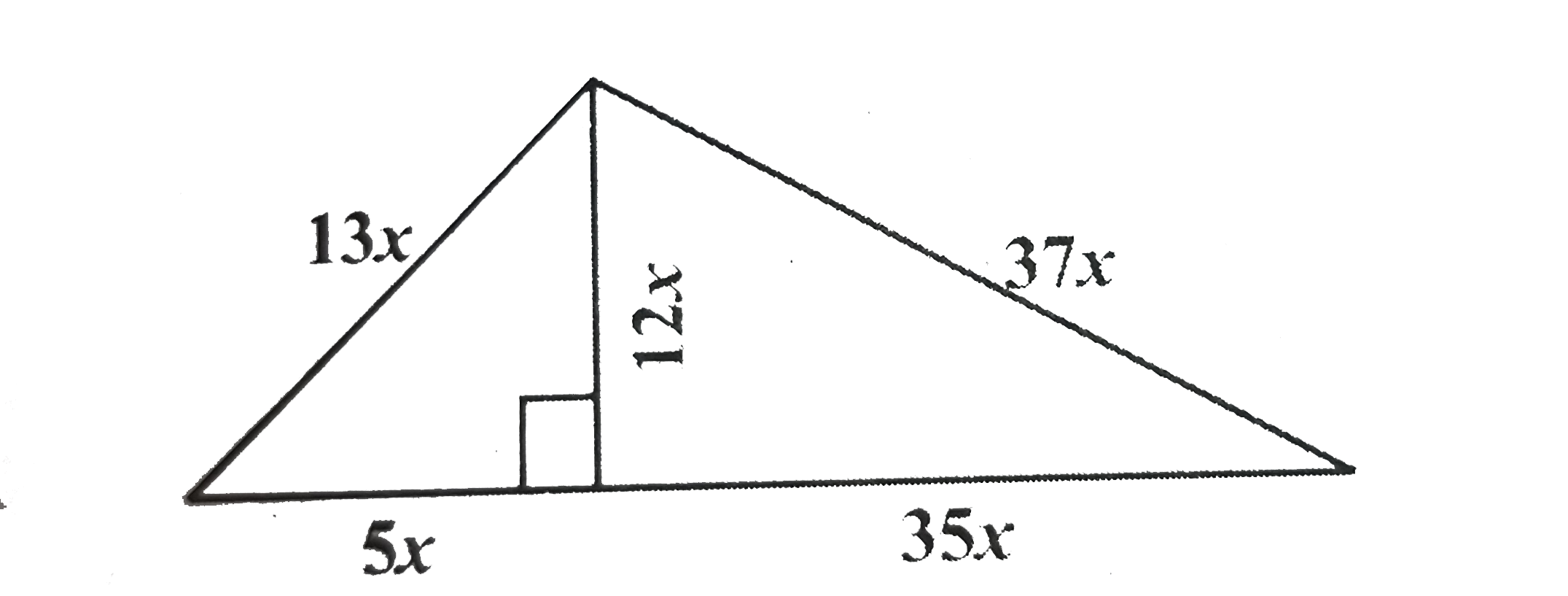
Find the ratio of the perimeter to the area of the given triangle.
View SolutionA linear equation in two variables represent a ………………. In xy plane.
View SolutionFill up the empty box in each of the given expression so that the resulting quadratic polynomial becomes a perfect square.
(i) x2+14x+ …………….
(ii) x2−24x+ ……………..
(iii) (p)2+2qp+ ………………..
View SolutionThe area of the triangle formed by the points (0,0),(3,0) and (0,4) is :
View SolutionIf A=[aij] is a 3×3 diagonal matrix such that a11=1 , a22=2 and a33=3 , then find |A| .
View SolutionLet A=[aij]3×3 be a matrix such that A.AT=4I and aij+2cij=0 where cij is the cofactor of aij∀i& j, I is the unit matrix of order 3 and AT is the transpose of the matrix A
If ∣∣ ∣∣a11+4a12a3a21a22+4a24a31a32a33+4∣∣ ∣∣+5λ∣∣ ∣∣a11+1a12a13a21a22+1a23a31a32a33+1∣∣ ∣∣=0 then λ=ab where a and b are coprime positive integers then the value of a+bis______
View SolutionIf A=⎡⎢ ⎢⎣5−26 17 08−3√2 354 3⎤⎥ ⎥⎦ then write
(i) the number of rows in A,
(ii) the number of columns in A,
(iii) the order of the matrix A,
(iv) the number of all entries in A,
(v) the elements a23,a31,a14,a33,a22 of A.
View SolutionLet A=[aij]3×3 be a scalar matrix and a11+a22+a33=15 then write matrix A.
View SolutionIf A=⎡⎢⎣a11a12a13a21a22a23a31a32a33⎤⎥⎦ and Aij denote the cofactor of element aij, then a11A21+a12A22+a13A23=
View SolutionIf A=⎡⎢⎣a11a12a13a21a22a23a31a32a33⎤⎥⎦andaij denote the cofactor of element Aij, then a11A11+a12A12+a13A13=
View SolutionIf matrix A=⎡⎢⎣10−1345067⎤⎥⎦ and its inverse is denoted by A−1=⎡⎢⎣a11a12a13a21a22a23a31a32a33⎤⎥⎦, then the value of a23=
View SolutionIn the matrix A=⎡⎢ ⎢ ⎢ ⎢ ⎢⎣8943−1√7√325143068−111⎤⎥ ⎥ ⎥ ⎥ ⎥⎦, write
Write the elements a22,a23,a24,a34,a43,a44,
View Solutionif a = 3 and a7=33, then a11 is
View SolutionFind minors and cofactors of the elements of the determinant|2−3560415−7|and verify that a11A31+a12A32+a13A33=0
View SolutionLet A=[aij]3×3 be a matrix such that AAT=4I and aij+2cij=0, where Cij is the cofactor of aij and I is the unit matrix of order 3.
∣∣ ∣∣a11+4a12a13a21a22+4a23a31a32a33+4∣∣ ∣∣+5λ∣∣ ∣∣a11+1a12a13a21a22+1a23a31a32a33+1∣∣ ∣∣=0
then the value of λ is
View Solution
