यदि f1(x) और f2(x) क्रमशः प्रान्त D1 और D2 पर परिभाषित हैं, तो f1(x)+f2(x), प्रान्त D1⋃D2,पर परिभाषित है।
The correct Answer is:असत्य
Step by step video, text & image solution for यदि f_(1)(x) और f_(2)(x) क्रमशः प्रान्त D_(1) और D_(2) पर परिभाषित हैं, तो f_(1)(x) + f_(2)(x), प्रान्त D_(1)uuuD_(2),पर परिभाषित है। by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - फलनEXERCISE - मैट्रिक्स-सुमेल प्रकार2videos
फलन
- BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - फलनEXERCISE - दृढ़कथन-कारण प्रकार1videos
फलन
- BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - फलनEXERCISE - रिक्त स्थानों की पूर्ति कीजिए6videos
फलन
- BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - प्रायिकताEXERCISE - एकल पूर्णांक प्रश्न2videos
प्रायिकता
- BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - लघुगणक एवं उनके गुणEXERCISE - एकल पूर्णांक प्रश्न1videos
लघुगणक एवं उनके गुण
Similar Questions
माना स्तम्भ I में परिभाषित फलन का प्रान्त (−π2,π2) एक तथा परास (−∞,∞) है
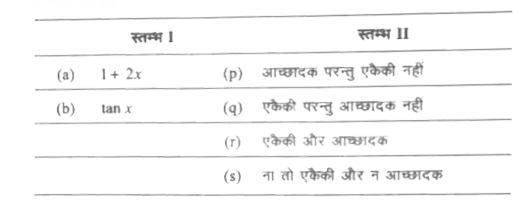 05:42View Solution
05:42View Solutionमाना सभी वास्तविक x के लिए f(x)=2+cosx
कथन - 1 : सभी वास्तविक t के लिए [t,t+π] में एक बिन्दु इस प्रकार है कि f'(c)=0 क्योंकि
कथन - 2 : f(t)=f(t+2π) प्रत्येक वास्तविक t के लिए02:07View Solutionf(x)=3sin(√π216−x)का मान अन्तराल .............. में है।
02:44View Solutionतीन स्वतंत्र घटनाओं E1,E2 तथा E3 में से केवल E1 के घटने की प्रायिकता α है केवल E2 के घटने की प्रायिकता β है तथा केवल E3 के घटने की प्रायिकता γ है। माना कि घटनाओं E1,E2 या E3 में से किसी के भी न घटने की प्रायिकता p, समीकरणों (α−2β)p=αβ तथा (β−3γ)p=2βγ को संतुष्ट करती है। सभी प्रायिकताएं अंतराल (0,1) में स्थित मानी जाती है तब E1के घटने की प्रायिकताE3के घटने की प्रायिकता=
04:39View Solutionपद 6+log32⎛⎜ ⎜⎝13√2 ⎷4−13√2 ⎷4−13√2√4−13√2…………..⎞⎟ ⎟⎠ का मान है
05:22View SolutionFor x varepsilon R,x!=0,x!=1, Let f_(0)(x)=(1)/(1-x) and f_(n+1)(x)=f_(0)(f_(n)(x)),n=0,1,2,3...... Then the value of f_(100)(3)+f_(1)((2)/(3))+f_(2)((3)/(2)) is equal to:
03:35View SolutionIf f_(1)(x) and f_(2)(x) are defined on domains D_(1) and D_(2), respectively,then f_(1)(x)+f_(2)(x) is defined on D_(1)nn D_(2) .
07:47View SolutionLet f1:R→Randf2:C→C are two functions such that f1(x)=x3andf2=x3.Prove that f1andf2are not same.
View SolutionLet f1(x)andf2(x) be twice differentiable functions where F(x)=f1(x)+f2(x)andG(x)=f1(x)−f2(x),∀x∈R,f1(0)=2andf2(0)=1.Iff'1(x)=f2(x)andf'2(x)=f1(x),∀x∈R. then the number of solutions of the equation (F(x))2=9x4G(x)is...... .
06:31View SolutionIf f1(x)=2x+3,f2(x)=3x2+5,f3(x)=x+cosx are defined from R→R, then f1,f2 and f3 are
01:55View Solutionf1(x)=x+a,f2(x)=x2+bx+c and Δ=∣∣ ∣ ∣∣111f1(x1)f1(x2)f1(x3)f2(x1)f2(x2)f2(x3)∣∣ ∣ ∣∣, then Δ is independent of
07:47View SolutionSupposed that f_(1):RtoR,f_(2):[0,oo)toR,f_(3):RtoR And f_(4):rto[0,oo) As follows f_(1)(x)={((|x),यदि,xlt0),(e^(x),यदि,xge0):} f_(2)(x)=x^(2) f_(3)(x)={(sinx, यदि,xlt0),(x, यदि,xge0):} And f_(a)(x)={(f_(2)(f_(1)(x)),यदि,xlt0),(f_(2)(f_(1)(x))-1,यदि ,xge0):}
05:28View SolutionF(x) को दो वास्तविक फलनों f1(x),x∈IR, तथा f2(x)={sin1xयदिx≠00यदिx=0
के गुणनफल के रूप में निम्न प्रकार | 0, यदि x = 0 है से परिभाषित कीजिये F(x)={f1(x)f2(x)यदिx≠00यदिx=0
कथन-1: Fx, IR पर सतत् है।
कथन-2 : f1(x) तथा f2(x) , IR पर सतत् है।View Solutionयदि fn(x)=efn−1(x)∀n∈N तथा f0(x)=x तो प्रदर्शित कीजिए कि ddx{fn(x)}=f1(x).f2(x)........fn(x) है।
06:07View SolutionAssertion (A) : If f:R→R defined by f(x)=sin−1(x2)+logx10, then the domain of the function is (0,2].
Reason (R) : If D1 and D2 are domains of f1 and f2 , then the domain of f1+f2 is D1∩D206:01View Solution
