यदि शून्येतर x के लिये , af(x)+bf(1x)=1x−5 जहाँ a≠b तो ∫21f(x)dx=
The correct Answer is:1a2−b2[alog2−5a+72b]
Step by step video, text & image solution for यदि शून्येतर x के लिये , af(x)+bf(1/x)=1/(x)-5 जहाँ a ne b तो int_(1)^(2)f(x) dx = by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- निश्चित समाकलन BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - निश्चित समाकलन EXERCISE - मैट्रिक्स-सुमेल प्रकार
1 Videos
- निश्चित समाकलन BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - निश्चित समाकलन EXERCISE - विश्लेषणात्क प्रश्न
28 Videos
- निश्चित समाकलन BOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - निश्चित समाकलन EXERCISE - बहुविकल्पीय प्रश्न II (एक से अधिक विकल्प सही है )
7 Videos
- द्विपद प्रमेयBOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - द्विपद प्रमेयEXERCISE - एकल पूर्णांक प्रश्न
3 Videos
- परवलयBOOK - JEE Main & Advanced (Hindi Medium)CHAPTER - परवलयEXERCISE - एकल पूर्णांक प्रश्न
5 Videos
Similar Questions
स्तम्भ I में दिए गए समाकलनो का स्तम्भ II में दिए गए मानो के साथ सुमेल कराये।
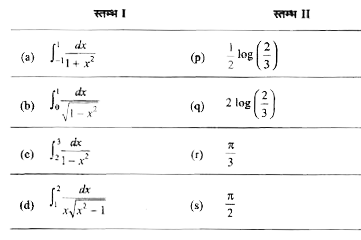
View Solution∫10(tx+1−x)ndx का मान ज्ञात कीजिए , जहाँ n एक धनात्मक पूर्णांक तथा प्राचल t, x से स्वतंत्र है। अतः प्रदर्शित कीजिए कि ∫10xk(1−x)n−kdx=1[nCk(n+1)],k=0,1,....n के लिए होगा।
View Solutionमाना Sn=n∑k=1nn2+kn+k2 एवं Tn=n∑k=0nn2+kn+k2 , n = 1, ,2, 3 ....... के लिए , तब
View Solution(1+x)n+5 के तीन क्रमागत पदों के गुणांक 5 : 10 : 14 के अनुपात में है, तब n का मान ज्ञात कीजिए ।
View Solutiony2=8x एक परवलय है। मान लीजिए एक त्रिभुज का क्षेत्रफल है जो नाभिलम्ब जीवा के सिरों और परवलय के बिन्दु P(12,2) द्वारा निर्मित है और Δ2 उस त्रिभुज का क्षेत्रफल है, जो बिन्दु P पर और नाभिलम्ब जीवा के सिरों पर खींची गई स्पर्शियों द्वारा बना है। तब Δ1Δ2 है।
View Solutionif for nonzero x,af(x)+bf((1)/(x))=(1)/(x)-5 where a!=b then f(2)=
View SolutionIf f(x) is monotonic differentiable function on [a,b], then int_(a)^(b)f(x)dx+int_(f(a))^(f(b))f^(-1)(x)dx=(a)bf(a)-af(b)(b) bf (b)-af(a)(c)f(a)+f(b)(d) cannot be found
View SolutionIf af(x)+bf((1)/(x))=x-1,x!=0 and a!=b, then f(2) is equal to ?
View SolutionIf af(x+1)+bf((1)/(x+1))=x,x!=-1,a!=b then f(2) is equal to
View Solution∫31f(x)dxजहाँ f(x)={2x+1यदि 1≤x≤2x2+1यदि 2≤x≤3
View SolutionIf for non-zero x,af(x)+bf(1x)=1x−5 where a≠b, then find ∫21f(x)dx.
View SolutionIf af(x)+bf(1/x)=x+5/x, (a ne b) , Then the value of f (x) is
View SolutionIf f(x) is monotonic differentiable function on [a , b] , then int_a^bf(x)dx+int_(f(a))^(f(b))f^(-1)(x)dx= (a) bf(a)-af(b) (b) bf(b)-af(a) (c) f(a)+f(b) (d) cannot be found
View SolutionWhich of the following is incorrect? ∫b+ca+cf(x)dx=∫baf(x+c)dx ∫bcacf(x)dx=c∫baf(cx)dx ∫a−af(x)dx=12∫a−a(f(x)+f(−x)dx None of these
View Solutionif for nonzero x, af(x)+bf(1x)=1x−5, where a≠b then f(2) =
View Solution
