Let the function be defined as
f(x)={4x0<x≤1<br>(−4x+5)1<x<2 then limx→1f(x) is :Solution in Tamil
4
does not exist
−4
1
The correct Answer is:B
Step by step video, text & image solution for Let the function be defined as f(x)={4x 0ltxle1(-4x+5) 1ltxlt2 then lim_(xrarr1)f(x) is : by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - PREMIERS PUBLISHERSCHAPTER - DIFFERENTIAL CALCULUS LIMITS AND CONTINUITYEXERCISE - SOLUTION TO EXERCISE 9.625videos
DIFFERENTIAL CALCULUS LIMITS AND CONTINUITY
- BOOK - PREMIERS PUBLISHERSCHAPTER - DIFFEREMTIAL CALCULUS DIFFERENTIABILITY AND METHODS OF DIFFERENTITAION EXERCISE - PROBLEMS FOR PRACTICE73videos
DIFFEREMTIAL CALCULUS DIFFERENTIABILITY AND METHODS OF DIFFERENTITAION
- BOOK - PREMIERS PUBLISHERSCHAPTER - EXAMINATION QUESTION PAPER MARCH 2019EXERCISE - PART -IV4videos
EXAMINATION QUESTION PAPER MARCH 2019
Similar Questions
Choose the correct or the most suitable answer from the given four alternatives.
limx→0sinxx01:38View SolutionIfy=13√x+log5x+8,f∈ddydx.
01:36View SolutionWrite any five different forms of an equation of a straight line.
05:47View SolutionLet f(x)={5x−4,0<x≤14x3−3x,1<x<2.
Find limx→1f(x).03:49View Solutionफलन f(x)={5x−4,0<x≤14x3−3x,1<x<2 है -
02:53View Solutionयदि f(x)={5x−4,0<x≤14x3−3x,1<x<2
सिद्ध कीजिए की limx→1f(x) एक अस्तित्व है।03:01View SolutionLet f:[2,∞)→R be the function defined by f(x)=x2−4x+5, then the range of f is
04:01View SolutionThe value of a for which the function f(x)={5x−4if0<x≤14x2+3axif1<x<2} is continous at every point of its domain is
03:30View SolutionShow that f(x)={5x−4, when 0<x≤1,4x3−3x , when 1<x<2 is continuous at x=1.
04:38View SolutionIf function f(x)={(5x-4,यदि 0ltxle1),(4x^(2)+3bx,यदि 1ltxlt2):} Each point of its domain is continuous, so the value of b will be
02:20View Solutionlimx→0f(x)and limx→1f(x)
if f(x)=⎧⎪⎨⎪⎩0⋅x≤0x≤11−2x0<x≤13−4xx>105:45View SolutionGiven the function f(x)={−x2ifx≤o, 5x−4if0<x≤1, 4x2−3xif1<x<2, 3x+4ifx≥2 Complete the following table
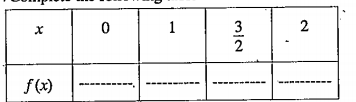 02:43View Solution
02:43View SolutionIf f(x)={5x−4,0<x≤14x2+3ax,1<x<2
04:53View SolutionLet f:[2,∞)→R be the function defined by f(x)=x2−4x+5. Then the range of f is
01:45View SolutionLet f(x)={5x−4,0<x≤14x3−3x,1<x<2.
Find limx→1f(x).03:49View Solution

