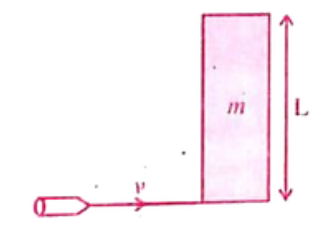
A rod of length L and mass M is hinged at point O. A small bullet of mass m ihits the rod with velocity v as shown in the figure. The bullet gets embedded in the rod. Calculate the angular velocity of the system just after the impact?
 Solution in Tamil
Solution in Tamil
Before impact, the bullet is moving. The initial impact, angular momentum of the system about O is
Li=mv×L=mvL……..1
After the bullet gets embedded in the rod, suppose the system attains angular velocity ω.
The moment of inerita of the bullet rod system about the axis through O is,
I= (M.I of bullet + M.I of rod)
=mL2+13ML2
I=(M+3m3)L2
Final angular momentum of the system is
Lf=Iω=(M+3m3)L2ω..2
By conservation of angular momentum,
Lf=Li
(M+3m3)L2ω=mvL
∴ Angular velocity
ω=3mv(M+3m)L
Topper's Solved these Questions
- BOOK - PREMIERS PUBLISHERSCHAPTER - MOTION OF SYSTEM OF PARTICLES AND BODIESEXERCISE - OTHER IMPORTANT QUESTIONS & ANSWER (CONCEPTUAL QUESTIONS)38videos
MOTION OF SYSTEM OF PARTICLES AND BODIES
- BOOK - PREMIERS PUBLISHERSCHAPTER - LAW OF MOTION EXERCISE - OTHER IMPORTANT QUESTIONS & ANSWERS (VI Numerical Problems)62videos
LAW OF MOTION
- BOOK - PREMIERS PUBLISHERSCHAPTER - NATURE OF PHYSICAL WORLD AND MEASUREMENTEXERCISE - OTHER IMPORTANT QUESTIONS & ANSWERS (III. Short Answer Questions.)24videos
NATURE OF PHYSICAL WORLD AND MEASUREMENT
Similar Questions
Should the centre of mass of a body necessarily lie inside the body?
10:20View SolutionA scooterist moving with a speed of 36mkmh−1 sees a child standing in the middle of the raod he applies the brakes and brings the scooter to rest in 5s just in time to saved child calculate the average retarding force on the vehicle if mass of the vehicle and drive is 300 kg
02:30View SolutionWhat is meant by Parallax?
08:13View SolutionA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the velocity of the centre of mass C and the angular, velocity of the system about the centre of mass after the collision.06:37View SolutionConsider a rod of mass M and length L pivoted at its centre is free to rotate in a vertical position plane. The rod is at rest in the vertical position. A bullet of mass M moving horizontally at a speed v strikes and embedded in one end of the rod. The angular velocity of the rod just after the collision will be.
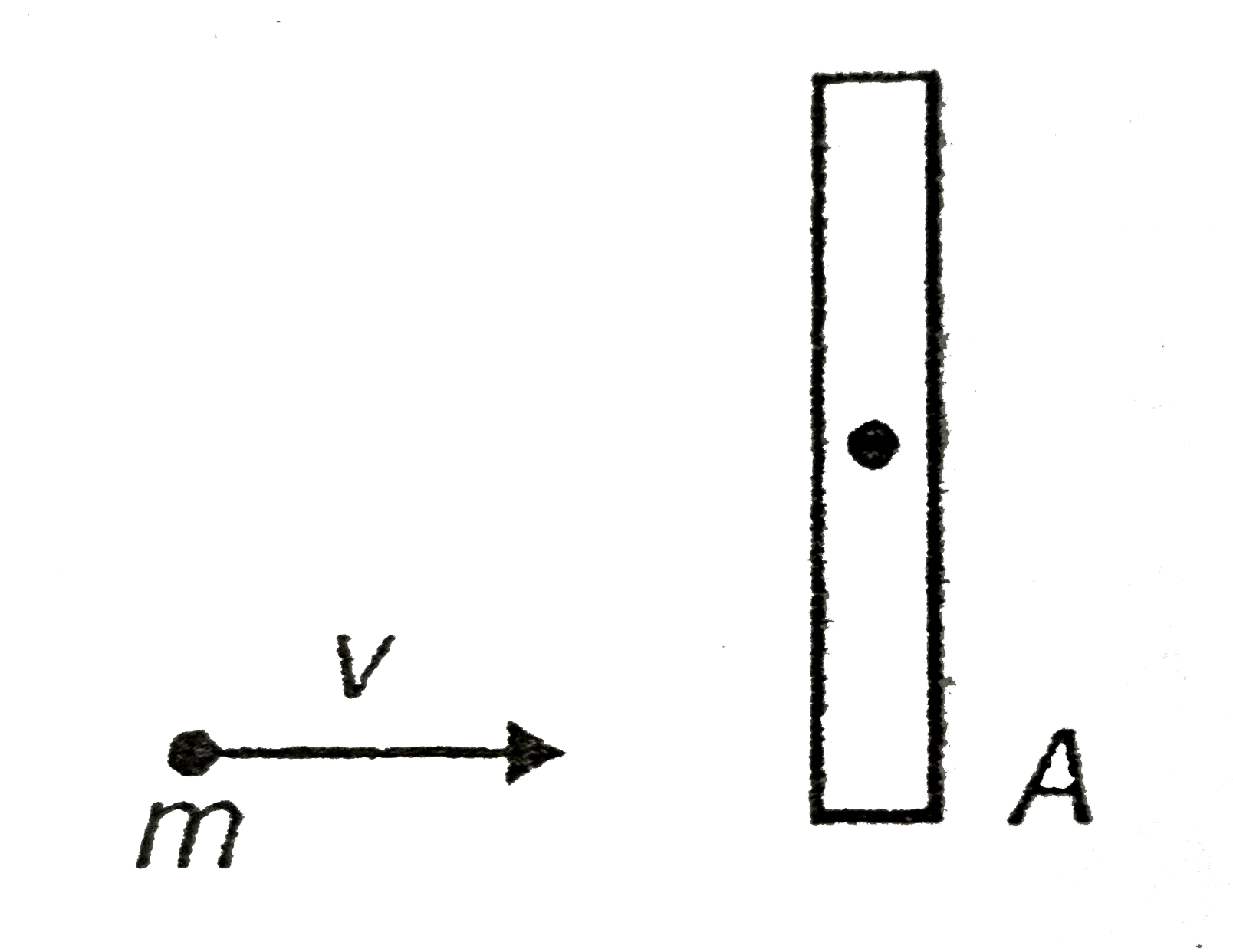
05:32View SolutionA rod of mass m and length l hinged at the centre is placed on a horizontal surface. A bullet of mass m moving with velocity v strikes the end. A of the rod and gets embedded in it. The angular velocity with which the systems rotates about its centre of mass after the bullet strikes the rod
 05:11View Solution
05:11View SolutionA rod of mass 4m and length L is hinged at the mid point. A ball of mass 'm' moving with speed V in the plane of rod, strikes at the end at an angle of 45∘ and sticks to it. The angular velocity of system after collision is-
03:13View SolutionA rod AB of length L and mass M is free to move on a frictionless horizontal surface . It is moving with a velocity v , as shown in figure. End B of rod AB strikes the end of the wall. Assuming elastic impact , the angular velocity of the rod AB , just after
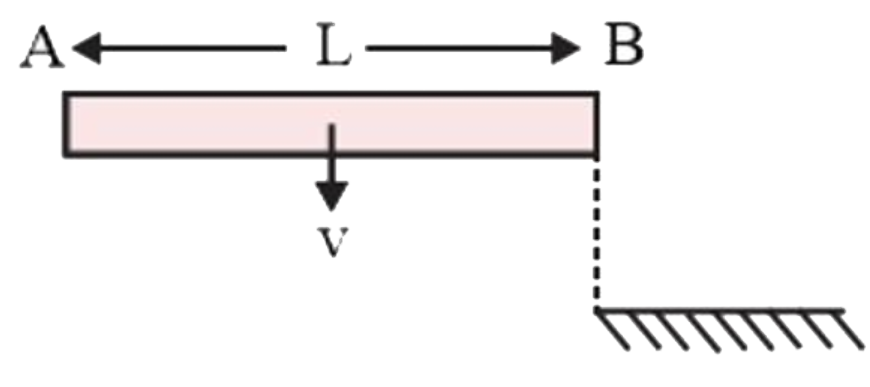 View Solution
View SolutionL लम्बाई तथा M द्रव्यमान की एक छड़ के बिंदु O पर कब्जा लगा है। एक छोटी m द्रव्यमान की गोली चित्रानुसार छड़ पर दागी जाती है गोली छड़ के भीतर धंस जाती है। टकराने के ठीक बार निकाय का कोणीय वेग ज्ञात कीजिए।
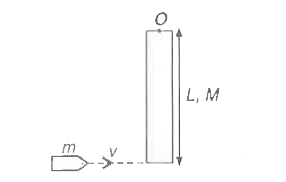 07:56View Solution
07:56View SolutionA rod of length L and mass M is hinged at point O. A small bullet of mass m ihits the rod with velocity v as shown in the figure. The bullet gets embedded in the rod. Calculate the angular velocity of the system just after the impact?
 09:15View Solution
09:15View SolutionA rod AB of length L and mass M is free to move on a frictionless horizontal surface. It is moving with a velocity v, as shown in figure. End B of rod AB strikes the end of the wall. Assuming elastic impact, the angular velocity of the rod AB, just after impact, is
06:00View Solution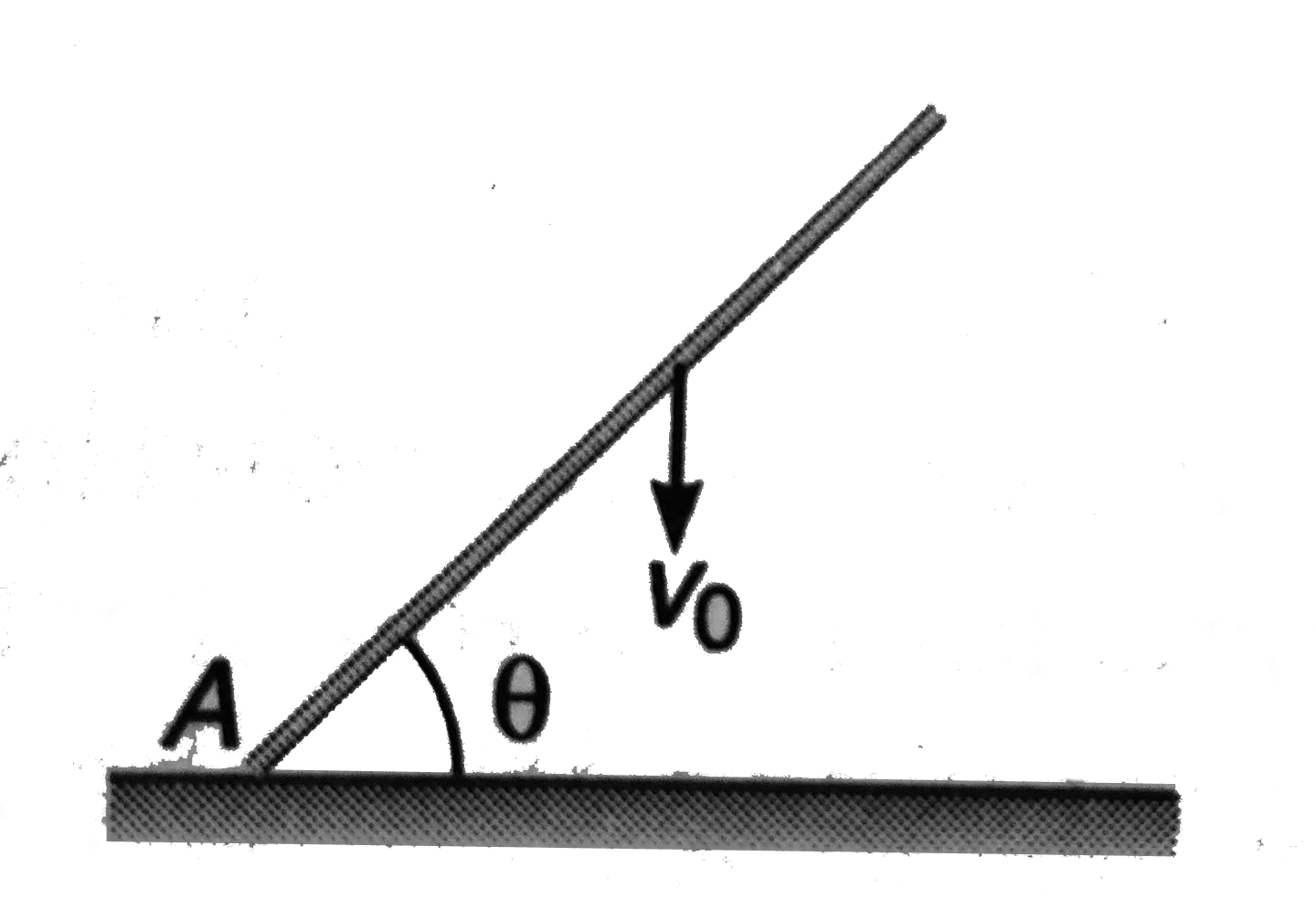
A rod of length l forming an angle θ with the horizontal strikes a frictionless floor at A with its centre of mass velocity v0 and no angular velocity. Assuming that the impact at A is perfectly elastic. Find the angular velocity of the rod immediately after the impact.04:33View SolutionA rod of mass M and length L is kept on a frictionless horizontal floor. A particle of mass m moving perpendicular to the rod with a speed v strikes at the end of the rod and sticks there. Calculate angular velocity acquired by the combined system.
View SolutionA rod of mass m,length l pivoted at its centre is free to rotate in a vertical plane.The rod is at rest and is in vertical position.A bullet of mass m/2 moving horizontally with speed v strikes and sticks to one end of the rod. Angular velocity of system just after collision is
View SolutionA rod of mass 4m and length L is hinged at the mid point. A ball of mass 'm' moving with speed V in the plane of rod, strikes at the end at an angle of 45^@ and sticks to it. The angular velocity of system after collision is-
03:33View Solution

