Assume that you are in another solar system and provided with the set of data given below consisting of the planet's semi major axes and time periods. Can you infer the relation connecting semi major axis and time period?

Time period T2∝a3
T1=2,a1=8
a1=22×2=2T21(T1=2)
a2=32×2=2T22(T2=3)
a3=42×2=2T23(T3=4)
a4=52×2=2T24(T4=5)
a5=62×2=2T25(T5=6)
∴a∝2T2
Topper's Solved these Questions
- GRAVITATIONBOOK - PREMIERS PUBLISHERSCHAPTER - GRAVITATIONEXERCISE - OTHER IMPORTANT QUESTION & ANSWERS (MULTIPLE CHOICE QUESTIONS)
162 Videos
- GRAVITATIONBOOK - PREMIERS PUBLISHERSCHAPTER - GRAVITATIONEXERCISE - OTHER IMPORTANT QUESTION & ANSWERS (VERY SHORT ANSWER QUESTION)
43 Videos
- GRAVITATIONBOOK - PREMIERS PUBLISHERSCHAPTER - GRAVITATIONEXERCISE - EVALUATION TEXTBOOK QUESTION & ANSWERS (CONCEPTUAL QUESTIONS)
16 Videos
- KINEMATICS BOOK - PREMIERS PUBLISHERSCHAPTER - KINEMATICS EXERCISE - Other important questions & answers (V. Numerical problems).
166 Videos
Similar Questions
You are at a distance of R=1.5×106 m from the centre of an unknown planet. You notice that if you throw a ball horizontally it goes completely around the planet hitting you in the back 90,000 seconds later with exactly the same speed that you originally threw it. If the length of semi major axis of ball is 2R, what is the of the planet. Express in form a×10b kg and fill a+b in OMR sheet.
[Take:G=203×10−11Nm2/kg2,π2=10]
View SolutionTwo planets of equal mass orbit a much massive star (figure). Planet m1 moves in circular orbit of radius 1×108km with period 2yr. Planet m2 moves in an elliptical orbit with closed distance r1=1×108km and farthest distance r2=1.8×108km, as shows.
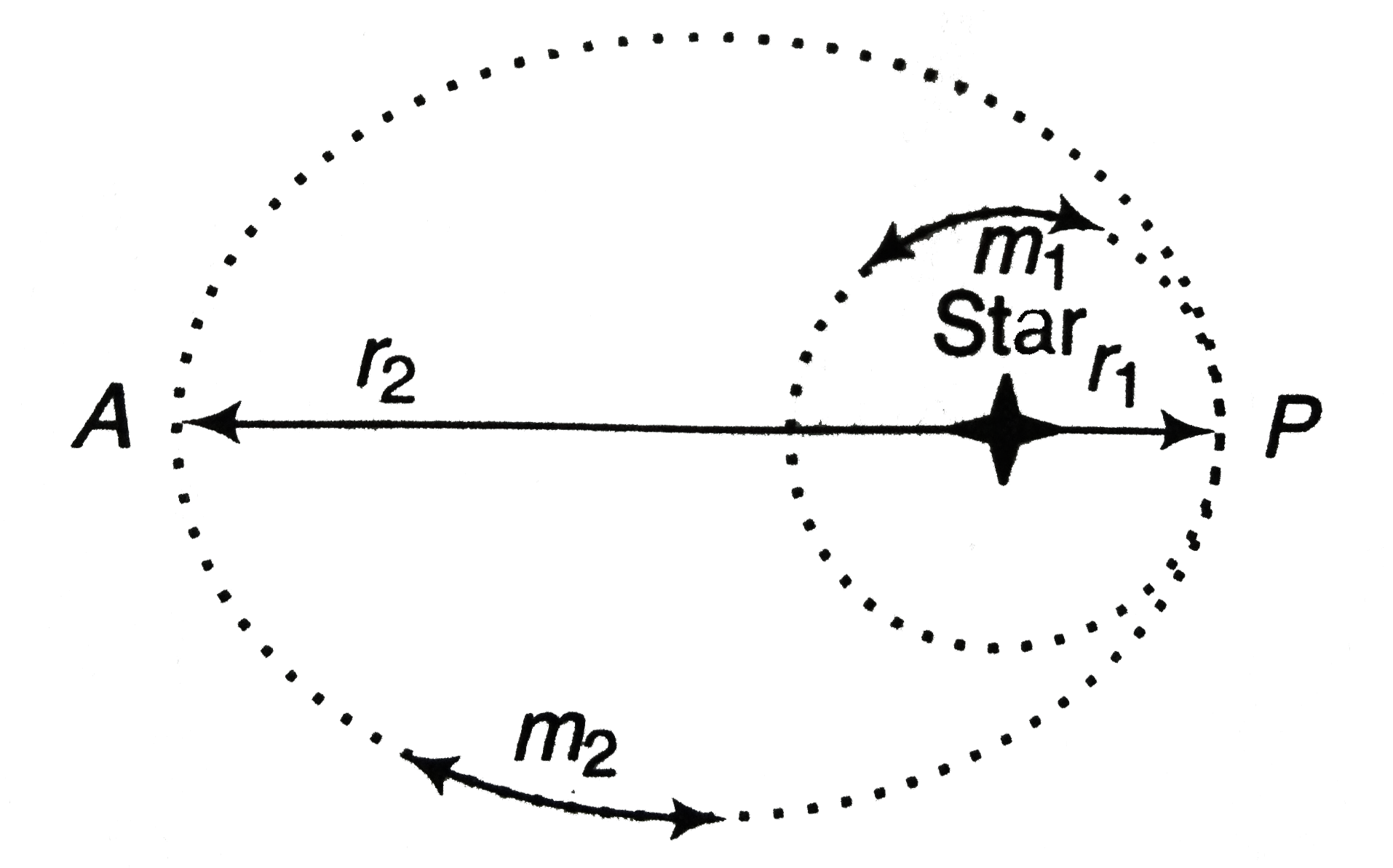
(a) Using the fact that the mean radius of an elliptical orbit is the length of the semi-major axis, find the period of m2's orbit.
(b) Which planet has the greater speed at point P? Which has the greater total energy?
(c ) Compare the speed of planet m2 at P with that at A.
View Solution
Knowledge Check
A planet revolves round the sun in an elliptical orbit of semi minor and semi major axes x and y respectively. Then the time period of revolution is proportional to
A(x+y)32B(y−x)32Cx32Dy32A planet revolves in an elliptical orbit around the sun. The semi-major and minor axes are a and b , then the time period is given by :
AT2∝b3BT2∝(a+b2)3CT2∝a3DT2∝(a−b2)3Assertion : The time period of a satellite revolving very close to the surface of earth is less.
Reason : According to Kepler's law, square of time period of revolution is directly proportional to the cube of semi-major axis.AIf both assertion and reason are correct and reason is a correct explanation of the assertion.BIf both assertion and reason are correct but reason is not the correct explanation of assertion.CIf assertion is correct but reason is incorrect.DIf assertion is incorrect but reason is correct.
Two planets of equal mass orbit a much massive star (figure). Planet m1 moves in circular orbit of radius 1×108km with period 2yr. Planet m2 moves in an elliptical orbit with closed distance r1=1×108km and farthest distance r2=1.8×108km, as shows.

(a) Using the fact that the mean radius of an elliptical orbit is the length of the semi-major axis, find the period of m2's orbit.
(b) Which planet has the greater speed at point P? Which has the greater total energy?
(c ) Compare the speed of planet m2 at P with that at A.
View SolutionThe following items consist of two statements, statement I and statement II. You are to examine these two statements carefully and select the answers to these items using the code given below:
Statement I : The social institutions of caste in India underwent major changes in the colonial period.
Statement II : Caste, in contemporary society is more a product of ancient Indian tradition than of colonialism.
View SolutionKepler's law starts that square of the time period of any planet moving around the sun in an elliptical orbit of semi-major axis (R) is directly proportional to
View SolutionAssertion : For circular orbits, the law of periods is T2∝r3, where M is the mass of sun and r is the radius of orbit.
Reason : The square of the period T of any planet about the sum is proportional to the cube of the semi-major axis a of the orbit.
View SolutionKepler's law starts that square of the time period of any planet moving around the sun in an elliptical orbit of semi-major axis (R) is directly proportional to
View Solution
