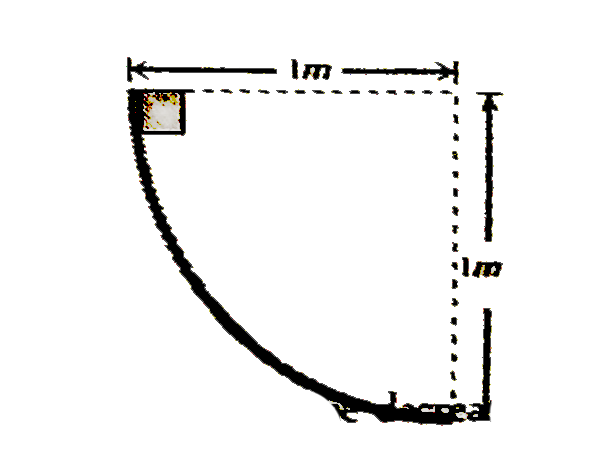
A body of mass 2kg slides down a curved track which is quadrant of a circle of radius 1 metre . All the surfaces are frictionless. If the body starts from rest, its speed at the bottom of the track is
4.43ms−1
2ms−1
0.5ms−1
19.6ms−1
Equating gain of kinetic energy with loss of gravitational potential energy, we get
12mv2=mgh
or v=√2gh
or v=√2×.9.8×1 ms−1<br>v=sqrt(19.6)" "ms^(-1)=4.43ms^(-1)
Topper's Solved these Questions
Similar Questions
A body of mass 5kg is moving along a circle of a radius 1m with a uniform speed of 5 ms.What is the acceleration of body?
View SolutionA block of mass M, with a semicircular track of radius R rest on a horizontal frictionless surface. A uniform cylinder of radius r and mass m is released from rest at the point of the semicircular track. The cylinder slips in the semicircular frictionless track. How far the block moved when the cylinder reaches the bottom of the track ?
View Solution
Knowledge Check
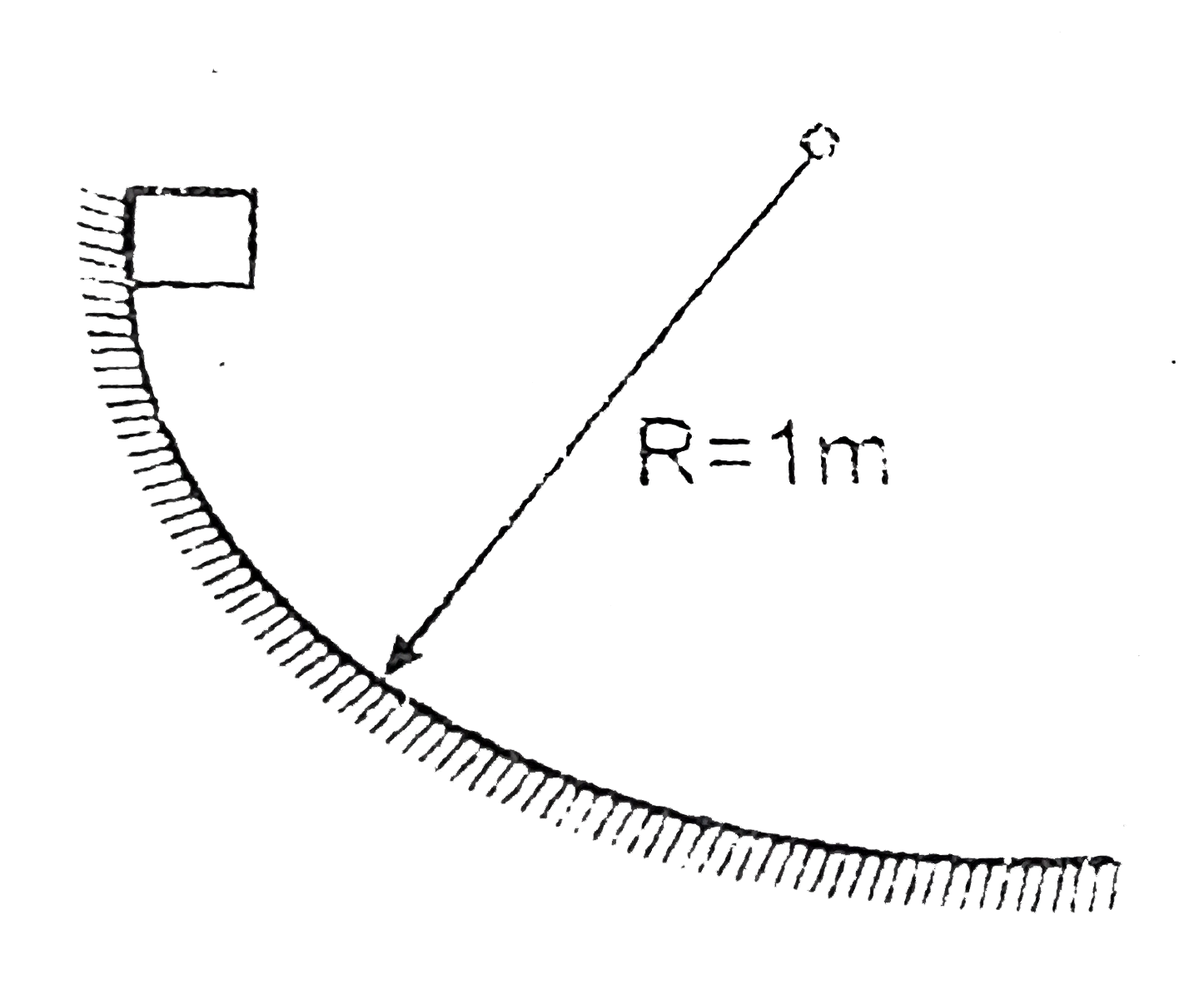
A body slides down a fixed curved track that is one quadrant of a circle of radius R, as in the figure. If there is no friction and the body starts from rest, its speed at the bottom of the track is
A5gRB√5gRC√2gRD√gRA block of mass 1 kg slides down a vertical curved track that is one quadrant of a circle of radius 1m. Its speed ast the the bottom si 2m/s. The work done by frictional force is :
 A8JB−8JC4JD−4J
A8JB−8JC4JD−4JA block of mass 1kg slides down a curved track which forms one quadrant of a circle of radius 1m as shown in figure. The speed of block at the bottom of track is v=2ms−1. The work done by the of friction is
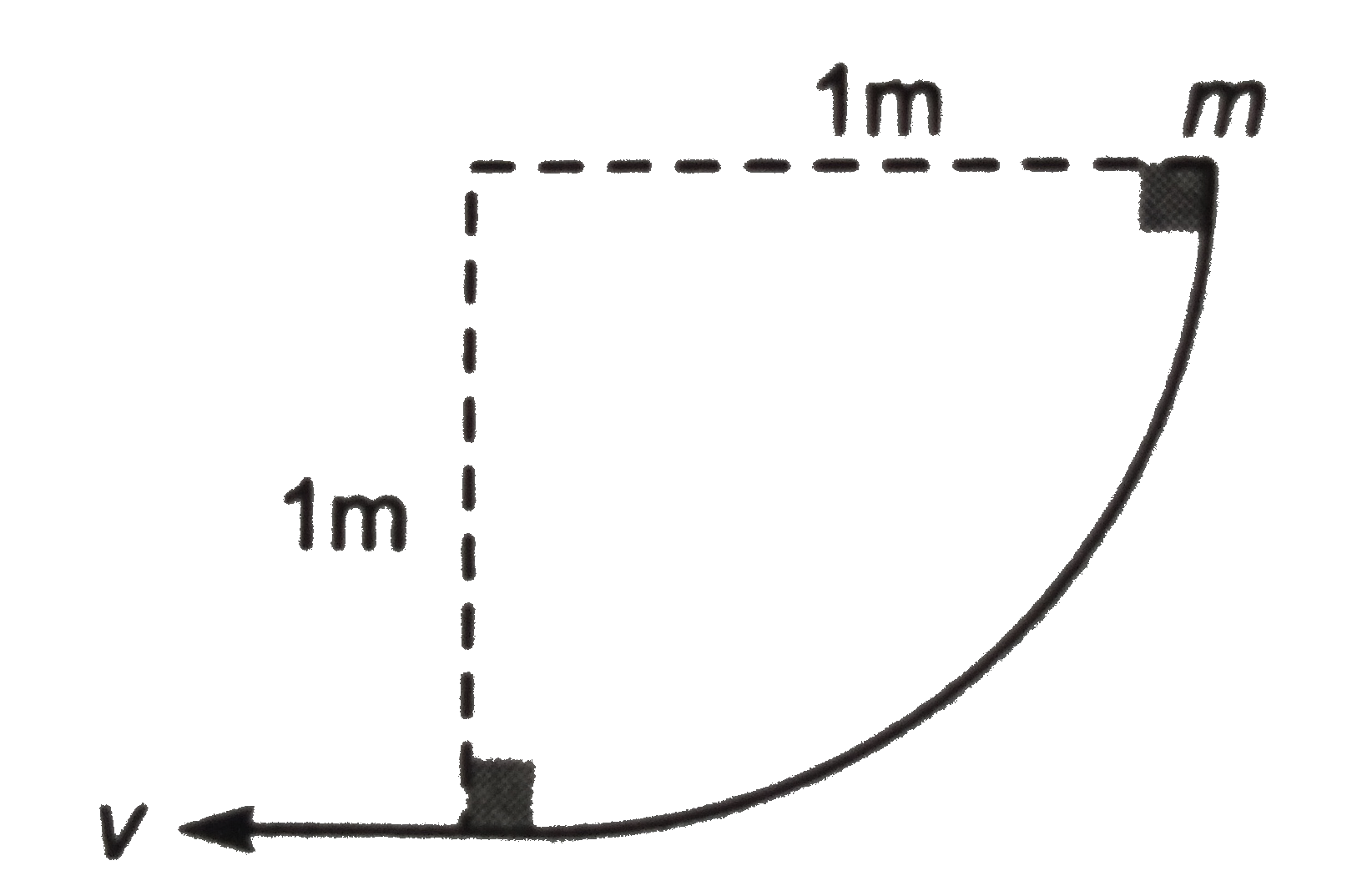 .A+4JB−4JC−8JD+8J
.A+4JB−4JC−8JD+8J
A ball rolls down an inclined track 2 m long 4s. Find (i) acceleration (ii) time taken to cover the second metre of the track and (iii) speed of the vall at the bottom of the track.
View SolutionA block of mass 1 kg slides down a vertical curved track that is one quadrant of a circle of radius 1m. Its speed ast the the bottom si 2m/s. The work done by frictional force is :

View SolutionA small body of mass m slides without friction from the top of a hemisphere of radius r. At what height will the body be detached from the surface of hemisphere?

View SolutionA body of mass 'm' is rotated by means of a string along a verticle of radius 'r' with constant speed .The difference in tension when the body is at the bottom and at the top of the verticle circle is
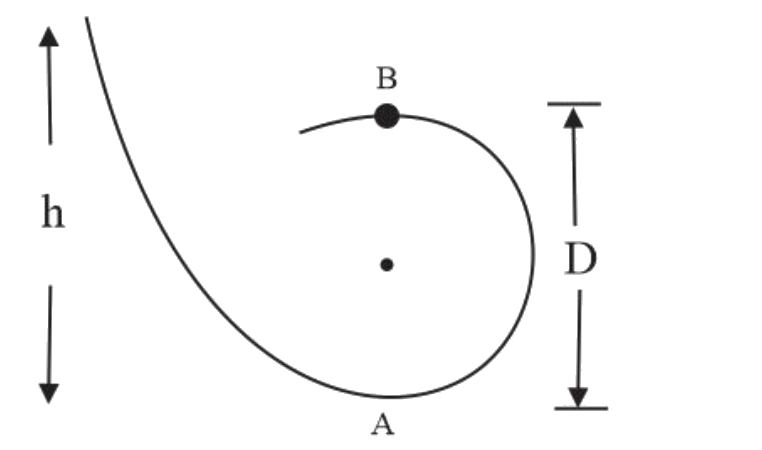
View SolutionA body slides down on a frictionless track which ends in a circular loop of diameter D. The minimum height h in terms of D so that the body may just complete the circular loop, is
View Solution
