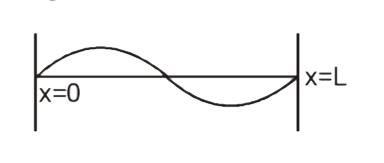
The equation of standing wave of the second resonance can be
,

A

$P_{e x} = 2 A sin 2 π (y = 1 c m) cos 2 π 9340) t$

B

$P_{e x} = 2 A sin 2 π (y = 1 c m) cos 2 π 9340) t$

C

$P_{e x} = 2 A sin 2 π (y = 1 c m) cos 2 π 9340) t$

D

$P_{e x} = 2 A sin 2 π (y = 1 c m) cos 2 π 9340) t$

Text Solution

Verified by Experts

$k = (2 π \frac{0}{λ} = \frac{2 π}{1} = 2 π ω = 2 π f = (2 π) (340)$
first node will be formed at $y = - 1$ instead of $y = 0$ so eqn of standing wave is $P_{e x} = 2 A sin 2 π (y + 1 c m) cos 2 π (340) t$ .

|

Updated on:21/07/2023

Class 12 PHYSICS EXPERIMENTAL PHYSICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
In a standing wave on a string.
AIn one time period all the particles are simultaneously at rest twice.
BAll the particles must be at their positive extremes simultaneously once in one time period.
CAll the particles may be at their positive extremes simultaneously once in a time period.
DAll the particles are never at rest simultaneously.
Question 2 - Select One
A string of length l along x-axis is fixed at both ends and is vibrating in second harmonic. If at $t = 0$ , $y = 2.5 m m$ for incident wave, the equation of standing wave is ( $T$ is tension and $μ$ is linear density)
A $(2.5 m m) sin (\frac{2 π}{l} x) cos (2 π \sqrt{(\frac{T}{μ l^{2}}) t})$
B $(5 m m) sin (\frac{π}{l} x) cos 2 π t$
C $(5 m m) sin (\frac{2 π}{l} x) cos (2 π \sqrt{(\frac{T}{μ l^{2}}) t})$
D $(5 m m) cos (\frac{2 π}{l} x) cos (2 π \sqrt{(\frac{T}{μ l^{2}}) t})$

RESONANCE-EXPERIMENTAL PHYSICS-PART -II

Home
Ask Doubt
Profile