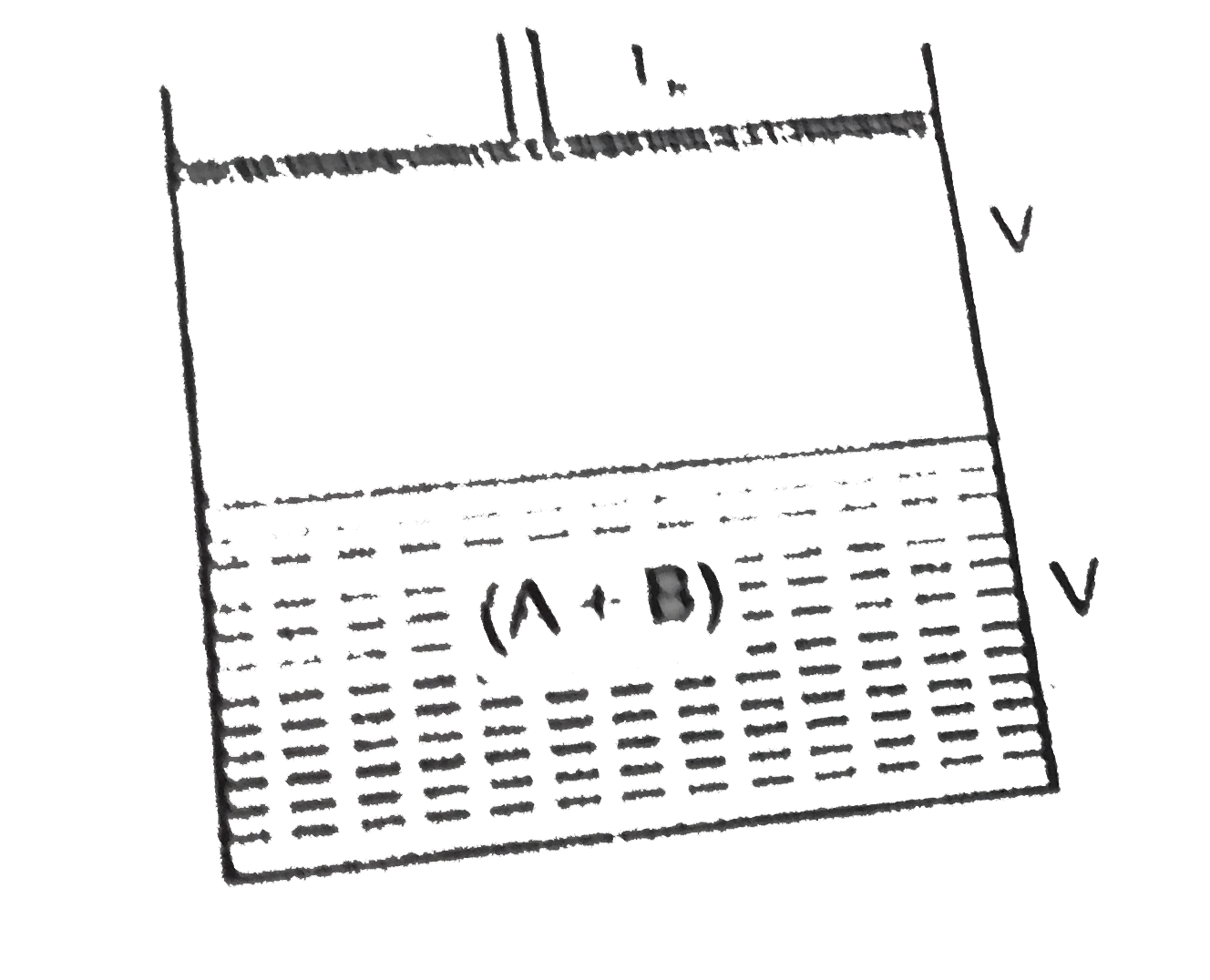
The system shown in the figure is in equilibrium, where A and B are isomeric liquids and form an ideal solution at TK.standard vapour pressure of A and B are P∘AandP∘B respectively at TK.We collect the vapour of A and B in two container of volume V, first container is maintained at 2TK and second container is maintained at 3T2K.At the temperature greater than TK, both A and B exist in only gaseous form.Total vapour pressure of the system at TK is given as
P=P∘AXA+P∘BXB
where XAandXB are the mole fraction of A and B in a liquid mixture.
In container (1)
We assume that collected gases behave ideally at 2TK and there can take place isomerisation reaction in which A converted into B by first order kinetics
Reaction is given as
AA−−→B
In container (II)
At the given temperature 3T2,A and B are ideal in nature and non mixing in nature.A small pin hole is made into container.We can determine the initial rate of effusion of both gases in vaccum by the expression
r=kp√m
Where P= pressure difference between system and surrounding
K=positibe constant
M=Molecular weight of the gas.
If partial vapour pressure of A is twice that of partial vapour pressure of B and total V.P. of equal to 2 at T=50 K and V=8.21 lit, then number of mole of A and B in vapour phase is :
83,43
3,1
2,2
1,3
The correct Answer is:A
PT=PA+PB=3PB
So, PB=23 and PA=2−23=43 Now PAPB=n1n2=2
So, n1=2n2
PTV=nTRT
2×8.21=nT×0.082×50
nT=4=n1+n2 So,n2=43 n1=83
Topper's Solved these Questions
Similar Questions
A red solid is insoluble in water.However it becomes soluble if some KI is added to water.Heating the red solid in a test tube results in liberation of some violet coloured fumes and droplets of a metal appear on the cooler parts of the test tube.The red solid is :
View SolutionSodium (Na=23) crystallizes in bcc arrangement with the interfacial separation between the atoms at the edge 53.6±. The density of sodium crystal is :
View SolutionThe system shown in the figure is in equilibrium, where A and B are isomeric liquids and form an ideal solution at TK . Standard vapour pressures of A and B are P_(A)^(0) and P_(B)^(0) , respectively, at TK . We collect the vapour of A and B in two containers of volume V , first container is maintained at 2 T K and second container is maintained at 3T//2 . At the temperature greater than T K , both A and B exist in only gaseous form. We assume than collected gases behave ideally at 2 T K and there may take place an isomerisation reaction in which A gets converted into B by first-order kinetics reaction given as: Aoverset(k)rarrB , where k is a rate constant. In container ( II ) at the given temperature 3T//2 , A and B are ideal in nature and non reacting in nature. A small pin hole is made into container. We can determine the initial rate of effusion of both gases in vacuum by the expression r=K.(P)/(sqrt(M_(0))) where P= pressure differences between system and surrounding K= positive constant M_(0)= molecular weight of the gas If partial vapour pressure of A is twice that of partial vapour pressure of B and total vapour pressure 2 atm at T K , where T=50 K and V=8.21 L , then the number of moles of A and B in vapour phase is:
View SolutionThe system shown in the figure is in equilibrium, where A and B are isomeric liquids and form an ideal solution at TK. Standard vapour pressures of A and B are P0A and P0B, respectively, at TK. We collect the vapour of A and B in two containers of volume V, first container is maintained at 2TK and second container is maintained at 3T/2. At the temperature greater than TK, both A and B exist in only gaseous form.
We assume than collected gases behave ideally at 2TK and there may take place an isomerisation reaction in which A gets converted into B by first-order kinetics reaction given as:
Ak−−→B, where k is a rate constant.
In container (II) at the given temperature 3T/2, A and B are ideal in nature and non reacting in nature. A small pin hole is made into container. We can determine the initial rate of effusion of both gases in vacuum by the expression

r=K.P√M0
where P= pressure differences between system and surrounding
K= positive constant
M0= molecular weight of the gas
Vapours of A and B are passed into a container of volume 8.21L, maintained at 2TK, where T=50K and after 5min, moles of B=8/3. The pressure developed into the cotainer after two half lives isView SolutionThe system shown in the figure is in equilibrium, where A and B are isomeric liquids and form an ideal solution at TK. Standard vapour pressures of A and B are P0A and P0B, respectively, at TK. We collect the vapour of A and B in two containers of volume V, first container is maintained at 2TK and second container is maintained at 3T/2. At the temperature greater than TK, both A and B exist in only gaseous form.
We assume than collected gases behave ideally at 2TK and there may take place an isomerisation reaction in which A gets converted into B by first-order kinetics reaction given as:
Ak−−→B, where k is a rate constant.
In container (II) at the given temperature 3T/2, A and B are ideal in nature and non reacting in nature. A small pin hole is made into container. We can determine the initial rate of effusion of both gases in vacuum by the expression

r=K.P√M0
where P= pressure differences between system and surrounding
K= positive constant
M0= molecular weight of the gas
If vapours are collected in a container of volume 8.21L maintained at 3T/2K, where T=50K, then the ratio of initial rate of effusion of gases A and B is given asView SolutionThe system shown in the figure is in equilibrium, where A and B are isomeric liquids and form an ideal solution at TK.standard vapour pressure of A and B are P∘AandP∘B respectively at TK.We collect the vapour of A and B in two container of volume V, first container is maintained at 2TK and second container is maintained at 3T2K.At the temperature greater than TK, both A and B exist in only gaseous form.Total vapour pressure of the system at TK is given as
P=P∘AXA+P∘BXB
where XAandXB are the mole fraction of A and B in a liquid mixture.
In container (1)
We assume that collected gases behave ideally at 2TK and there can take place isomerisation reaction in which A converted into B by first order kinetics
Reaction is given as
AA−−→B
In container (II)
At the given temperature 3T2,A and B are ideal in nature and non mixing in nature.A small pin hole is made into container.We can determine the initial rate of effusion of both gases in vaccum by the expression
r=kp√m
Where P= pressure difference between system and surrounding
K=positibe constant
M=Molecular weight of the gas.
Vapour is collected and passed into a container of volume 8.21 lit, maintainer at 100 K and after 5 min number of mole of B=83.Then calculate pressure develop into the container after two half lives.02:27View SolutionThe system shown in the figure is in equilibrium, where A and B are isomeric liquids and form an ideal solution at TK.standard vapour pressure of A and B are P_A^@ and P_B^@ respectively at TK.We collect the vapour of A and B in two container of volume V, first container is maintained at 2TK and second container is maintained at (3T)/2 K.At the temperature greater than TK, both A and B exist in only gaseous form.Total vapour pressure of the system at TK is given as P=P_A^@X_A+P_B^@X_B where X_A and X_B are the mole fraction of A and B in a liquid mixture. In container (1) We assume that collected gases behave ideally at 2TK and there can take place isomerisation reaction in which A converted into B by first order kinetics Reaction is given as AoversetAtoB In container (II) At the given temperature (3T)/2 ,A and B are ideal in nature and non mixing in nature.A small pin hole is made into container.We can determine the initial rate of effusion of both gases in vaccum by the expression r=(kp)/sqrtm Where P= pressure difference between system and surrounding K=positibe constant M=Molecular weight of the gas. If vapour is collected in container of volume 8.21 lit maintained at 75 K then ratio of initial rate of effusion of Gas A and B is given as :
View SolutionTwo liquids A and B have vapour pressure in the ratio P_A^@ : P_B^@=1:3 at a certain temperature. Assume A and B form an ideal solution and the ratio of mole fractions of A to B in the vapour phase is 4:3. Then the mole fraction of B in the solution at the same temperature is :-
View SolutionTwo liquids A and B have vapour pressure in the ratio P∘A:P∘B=1:3 at a certain temperature. Assume A and B form an ideal solution and the ratio of mole fractions of A to B in the vapour phase is 4:3. Then the mole fraction of B in the solution at the same temperature is :-
01:46View SolutionTwo liquids A and B form an ideal solution. At 300 K, the vapour pressure of a solution containing 1 mole of A and 3 moles of B is 550 mm of Hg. At the same temperature, if one mole of B is added to this solution, the vapour pressure of the solution increases by 10 mm of Hg. Determine the vapour pressure of A and B in their pure states
04:40View SolutionPA and PB are the vapour pressure of pure liquid components. A and B, respectively of an ideal binary solution. If xA represents the mole fraction of component A, the total pressure of the solution will be
03:09View SolutionThe system shown in the figure is in equilibrium, where A and B are isomeric liquids and form an ideal solution at TK. Standard vapour pressures of A and B are P0A and P0B, respectively, at TK. We collect the vapour of A and B in two containers of volume V, first container is maintained at 2TK and second container is maintained at 3T/2. At the temperature greater than TK, both A and B exist in only gaseous form.
We assume than collected gases behave ideally at 2TK and there may take place an isomerisation reaction in which A gets converted into B by first-order kinetics reaction given as:
Ak−−→B, where k is a rate constant.
In container (II) at the given temperature 3T/2, A and B are ideal in nature and non reacting in nature. A small pin hole is made into container. We can determine the initial rate of effusion of both gases in vacuum by the expression

r=K.P√M0
where P= pressure differences between system and surrounding
K= positive constant
M0= molecular weight of the gas
Vapours of A and B are passed into a container of volume 8.21L, maintained at 2TK, where T=50K and after 5min, moles of B=8/3. The pressure developed into the cotainer after two half lives is01:59View SolutionThe system shown in the figure is in equilibrium, where A and B are isomeric liquids and form an ideal solution at TK. Standard vapour pressures of A and B are P0A and P0B, respectively, at TK. We collect the vapour of A and B in two containers of volume V, first container is maintained at 2TK and second container is maintained at 3T/2. At the temperature greater than TK, both A and B exist in only gaseous form.
We assume than collected gases behave ideally at 2TK and there may take place an isomerisation reaction in which A gets converted into B by first-order kinetics reaction given as:
Ak−−→B, where k is a rate constant.
In container (II) at the given temperature 3T/2, A and B are ideal in nature and non reacting in nature. A small pin hole is made into container. We can determine the initial rate of effusion of both gases in vacuum by the expression

r=K.P√M0
where P= pressure differences between system and surrounding
K= positive constant
M0= molecular weight of the gas
If vapours are collected in a container of volume 8.21L maintained at 3T/2K, where T=50K, then the ratio of initial rate of effusion of gases A and B is given as02:53View SolutionPA and PB are the vapour pressure of pure liquid components, A and B, respectively of an ideal binary solution. If XA represents the mole fraction of component A, the total pressure of the solution will be
06:53View SolutionThe vapour pressure of a liquid solution containing A and B is 99 torr. Calculate mole % of B in vapour phase.
(Given : P0A = 100 torr , P0B = 80 torr)View Solution

