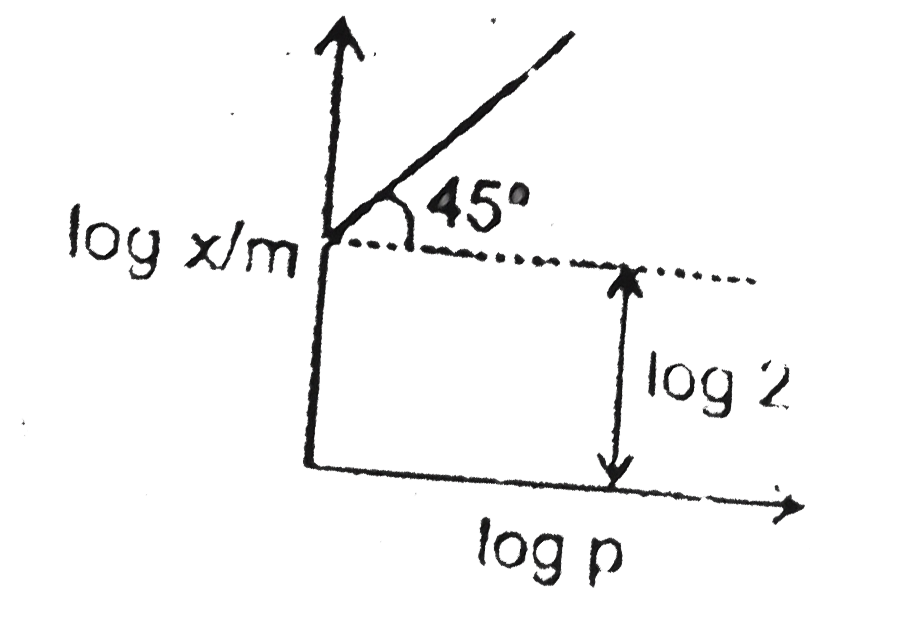
At 2 atm pressure the value of $\frac{x}{m}$ will be :(log 2=0.3010)

Video Solution

Text Solution

Verified by Experts

The correct Answer is:4

$\frac{x}{m} = K P^{1 / n}$
$log \frac{x}{m} = log K + \frac{1}{n} log P$
$∴ K = 2 & \frac{1}{n} = 1 or n = 1$
On putting in $e q^{n} (1)$
$\frac{x}{m} = 2 \times 2 = 4$

|

Updated on:21/07/2023

Class 12 CHEMISTRY QUESTION BANK

Topper's Solved these Questions

BOOK - RESONANCECHAPTER - QUALITATIVE ANALYSIS PART 1EXERCISE - A.L.P
39videos
QUALITATIVE ANALYSIS PART 1
BOOK - RESONANCECHAPTER - SOLID STATEEXERCISE - PHYSICAL CHEMITRY (SOLID STATE)
45videos
SOLID STATE

Similar Questions

Knowledge Check

Question 1 - Select One
If $2^{x} 3^{2 x} = 100$ then the value of x is (log 2 = 0.3010, log 3 = 0.4771)
A2.3
B1.59
C1.8
D1.41
Question 2 - Select One
If $2^{x} 3^{2 x} = 100$ then the value of x is (log 2 = 0.3010, log 3 = 0.4771)
A2.3
B1.59
C1.8
D1.41
Question 3 - Select One
If $2^{x} {.3}^{2 x} = 100$ , then the value of x is
$(log 2 = 0.3010, log 3 = 0.4771)$
AA) $2.3$
BB) $1.59$
CC) $1.8$
DD) $1.41$

RESONANCE-RANK BOOSTER-All Questions

Home
Ask Doubt
Profile