A game of chance consists of spinning an arrow which is equally likely to come to rest pointing to all of the numbers 1, 2, 3, ...,12. What is the probability that it will point to (i) 10 (ii) an odd number (iii) a number which is multiple of 3?
n(S) = Total number of elementary events= 12.
(i) Favourable number of elementary events that points to 10 = 1
∴ Required probability 112
(ii) Let A be the event "point of the arrow head towards an odd number"
∴ n(A)={1,3,5,7,9,11}=6
∴ P(A)=n(A)n(S)=612=12
(iii) Let E be the event "pointing towards a number which is a multiple of 3"
∴ n(E)={3,6,9,12}=4
∴ P(E)=n(A)n(S)=412=13
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - PROBABILITYEXERCISE - Problems From NCERT /exemplar10videos
PROBABILITY
- BOOK - NAGEEN PRAKASHANCHAPTER - PROBABILITYEXERCISE - Exercise29videos
PROBABILITY
- BOOK - NAGEEN PRAKASHANCHAPTER - POLYNOMIALSEXERCISE - Revision Exercise Long Answer Questions4videos
POLYNOMIALS
- BOOK - NAGEEN PRAKASHANCHAPTER - QUADRATIC EQUATIONSEXERCISE - Revision Exercise Long Answer Questions6videos
QUADRATIC EQUATIONS
Similar Questions
A game of chance consists of spinning an arrow which is equally likely to come to rest pointing to one of the numbers 1, 2, 3……., 12 as shown in the Fig. What is the probability that it will point to:
(i) 6? (ii) an even number? (iii) a prime number? (iv) a multiple of 4?
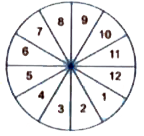 View Solution
View Solutiona game of chance consists of spinning an arrow which is equally likely to come to rest pointing to one of the numbers 1,2,3,….,12 as shown in the figure. What is the probability that it will point to
(i) 6? (ii) an even numbers?
(iii) a prime number? (iv) a number which is a multiple of 5?
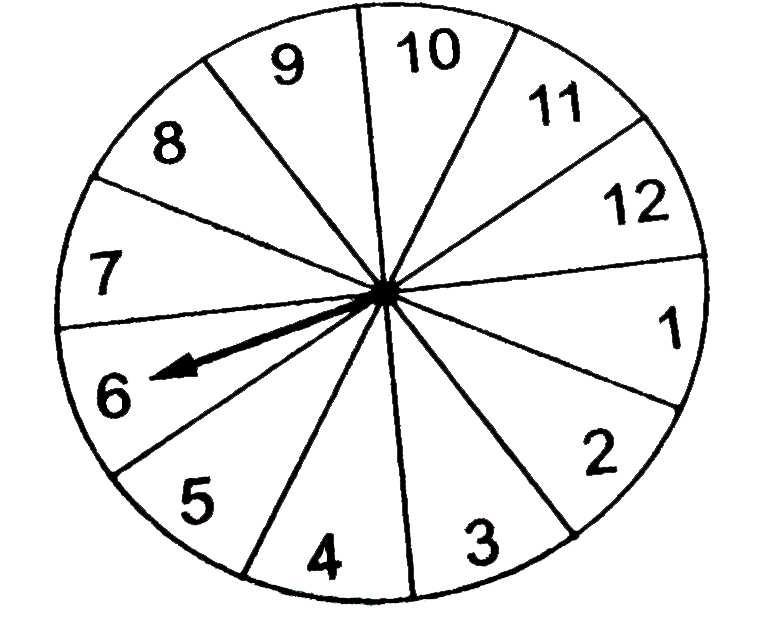 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A game of chance consists of spinning an arrow, which comes to rest pointing at one of the numbers 1,2,3,4,5,6,7,8 and these are equally likely outcomes. Find the probability that the arrow will point at any factor of 8.
A58B78C18D38 - Question 2 - Select One
A number is selected from the numbers 1,2 …, 15. What is the probability that it is a multiple of 4?
A715B25C15D215
A game of change consists of spinning an arrow which is equally likely to come to rest pointing to one of the number, 1, 2, 3, .., 12 as shown in Fig. 13.3. What is the probability that it will point to: (FIGURE) (i) 10? (ii) an odd number? (iii) a number which is multiple of 3? (iv) an even number?
View SolutionA game of chance consists of spinning an arrow which comes to rest pointing at one of the numbers 1,2 3,4,5,6,7,8 and these are equally likely outcomes what is the probability that it will point at :
(i) 8?
(ii) an odd number ?
(iii) a number greater than 2 ?
(iv) a number less than 9 ?View SolutionA game of chance consist of spinning an arrow which comes to rest pointing at one of the numbers 15,20,25,30,35,40,45,50 and these are equally likely outcomes. What is the probability that it will point at
(i) an even number? (ii) at a number multiple of 5
(iii) at a number divisible by 3 (iv) at a prime number.View SolutionA game of chance consists of spinning an arrow which comes to rest pointing at one of the numbers 1,2,3,4,5,6,1,8 (see Figure), and these are equally likely outcomes. What is the probability that it will point at(i) 8?(ii) an odd number?(iii) a number greater than 2?(iv) a number less than 9?
View SolutionA game of chance consists of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 (See Fig. 131), and these are equally likely outcomes. What is the probability that it will point at (i) 8? (ii) an odd number? (iii) a number greater than 2? (iv) a number less than 9?
View SolutionA game of chance consists of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 (see figure) and these are equally likely outcomes. What is the probability that it will point at :
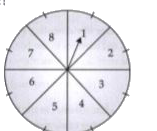
(i) 8? (ii) an odd number?
(iii) a number greater than 2? (iv) a number less 9?View Solution

